No CrossRef data available.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Published online by Cambridge University Press: 23 April 2018
Let 
${\mathcal{A}}=\{a_{1}<a_{2}<\cdots \,\}$ be a set of nonnegative integers. Put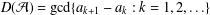 
$D({\mathcal{A}})=\gcd \{a_{k+1}-a_{k}:k=1,2,\ldots \}$. The set 
${\mathcal{A}}$ is an asymptotic basis if there exists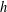 
$h$ such that every sufficiently large integer is a sum of at most 
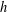$h$ (not necessarily distinct) elements of 
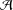${\mathcal{A}}$. We prove that if the difference of consecutive integers of 
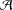${\mathcal{A}}$ is bounded, then 
${\mathcal{A}}$ is an asymptotic basis if and only if there exists an integer 
$a\in {\mathcal{A}}$ such that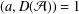 
$(a,D({\mathcal{A}}))=1$.
This work was supported by the National Natural Science Foundation of China (Grant No.11471017).
You have
Access