Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-24T00:34:05.485Z Has data issue: false hasContentIssue false
  • English
  • Français

A NOTE ON SCHMIDT’S CONJECTURE

Part of: Arithmetic algebraic geometry Diophantine equations

Published online by Cambridge University Press:  09 June 2017

DIMITRIOS POULAKIS Open the ORCID record for DIMITRIOS POULAKIS [Opens in a new window]
DIMITRIOS POULAKIS*
Affiliation:
Department of Mathematics, Aristotle University of Thessaloniki, 54124 Thessaloniki, Greece email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

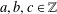Schmidt [‘Integer points on curves of genus 1’, Compos. Math. 81 (1992), 33–59] conjectured that the number of integer points on the elliptic curve defined by the equation $y^{2}=x^{3}+ax^{2}+bx+c$, with $a,b,c\in \mathbb{Z}$, is 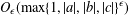$O_{\unicode[STIX]{x1D716}}(\max \{1,|a|,|b|,|c|\}^{\unicode[STIX]{x1D716}})$ for any 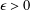$\unicode[STIX]{x1D716}>0$. On the other hand, Duke [‘Bounds for arithmetic multiplicities’, Proc. Int. Congress Mathematicians, Vol. II (1998), 163–172] conjectured that the number of algebraic number fields of given degree and discriminant 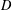$D$ is 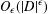$O_{\unicode[STIX]{x1D716}}(|D|^{\unicode[STIX]{x1D716}})$. In this note, we prove that Duke’s conjecture for quartic number fields implies Schmidt’s conjecture. We also give a short unconditional proof of Schmidt’s conjecture for the elliptic curve $y^{2}=x^{3}+ax$.

Keywords

elliptic curvediscriminantquartic number field

MSC classification

Primary: 11G05: Elliptic curves over global fields
Secondary: 11D25: Cubic and quartic equations 11D45: Counting solutions of Diophantine equations
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 96 , Issue 2 , October 2017 , pp. 191 - 195
Copyright
© 2017 Australian Mathematical Publishing Association Inc. 

References

Berczes, A., ‘On the number of solutions of norm form equations’, Period. Math. Hungar. 43(1–2) (2001), 165–176.Google Scholar
Bhargava, M., Shankar, A., Taniguchi, T., Thorne, F., Tsimerman, J. and Zhao, Y, ‘Bounds on 2-torsion in class groups of number fields and integral points on elliptic curves’, Preprint 2017, arXiv:1701.02458v1.Google Scholar
Chabauty, C., ‘Démonstration de quelques lemmes de rehaussement’, C. R. Acad. Sci. Paris 217 (1943), 413–415.Google Scholar
Draziotis, K., ‘On the number of integer points on the elliptic curve y 2 = x 3 + Ax ’, Int. J. Number Theory 7(3) (2011), 611–621.CrossRefGoogle Scholar
Duke, W., ‘Bounds for arithmetic multiplicities’, in: Proceedings of the International Congress of Mathematicians, Vol. II (Berlin, 1998), 163–172 (electronic supplement).Google Scholar
Evertse, J.-H., ‘On equations in S-units and the Thue–Mahler equation’, Invent. Math. 75 (1984), 561–584.CrossRefGoogle Scholar
Evertse, J.-H. and Silverman, J.-H., ‘Uniform bounds for the number of solutions to Y n = f (X)’, Math. Proc. Cambridge Philos. Soc. 100 (1986), 237–248.CrossRefGoogle Scholar
Hardy, G. H. and Wright, E. M., An Introduction to the Theory of Numbers, 5th edn (Oxford University Press, Oxford, 1979).Google Scholar
Helfgott, H. A. and Venkatesh, A., ‘Integral points on elliptic curves and 3-torsion in class groups’, J. Amer. Math. Soc. 19(3) (2006), 527–550.Google Scholar
Kappe, L.-C. and Warren, B., ‘An elementary test for the Galois group of a quartic polynomial’, Amer. Math. Monthly 96(2) (1989), 133–137.Google Scholar
Klüner, J., ‘The number of S 4 -fields with given discriminant’, Acta Arith. 122(2) (2006), 185–194.Google Scholar
Poulakis, D., ‘Integer points on algebraic curves with exceptional units’, J. Aust. Math. Soc. 63 (1997), 145–164.CrossRefGoogle Scholar
Poulakis, D., ‘The number of solutions of the Mordell equation’, Acta Arith. 88(2) (1999), 173–179; Corrigendum, Acta Arith. 92(4) (2000), 387–388.Google Scholar
Schmidt, W., ‘Integer points on curves of genus 1’, Compos. Math. 81(1) (1992), 33–59.Google Scholar
Silverman, J. H., The Arithmetic of Elliptic Curves, Graduate Texts in Mathematics, 106 (Springer, New York, 1986).Google Scholar
Travesa, A., ‘Nombre d’extensions abéliennes sur ℚ’, Sémin. Théor. Nombres Bordeaux 2 (1990), 413–423.Google Scholar
Yang, L., Hou, X. R and Zeng, Z. B., ‘A complete discrimination system for polynomials’, Sci. China E 39 (1996), 628–646.Google Scholar