Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-28T22:31:46.767Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on injectors in finite soluble groups

Published online by Cambridge University Press:  17 April 2009

John Cossey
John Cossey
Affiliation:
Department of Pure Mathematics, Faculty of Arts, Australian National University, Canberra, ACT.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Groups of nilpotent length four containing a subgroup which covers and avoids the same chief factors as an 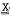-injector for some Fitting class 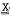but which is not itself an 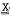-injector have been constructed by F.P. Lockett (in his PhD thesis) and T.R. Berger and John Cossey (in preparation). Graham A. Chambers (J. Algebra 16 (1970), 442–455) has shown that such a subgroup cannot exist in a group of p–length one for all primes p. The main result of this paper closes the small gap remaining: it includes Chambers' result and establishes also that such a subgroup cannot exist in a group of nilpotent length three.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 17 , Issue 3 , December 1977 , pp. 419 - 421
Copyright
Copyright © Australian Mathematical Society 1977

References

[1]Berger, T.R. and Cossey, John, “More Fitting formations”, in preparation.Google Scholar
[2]Chambers, Graham A., “p–normally embedded subgroups of finite soluble groups”, J. Algebra 16 (1970), 442–455.CrossRefGoogle Scholar
[3]Lockett, F.P., “On the theory of Pitting classes of finite soluble groups” (PhD thesis, University of Warwick, Coventry, 1971).Google Scholar