Article contents
A NOTE ON ASYMPTOTIC NONBASES
Published online by Cambridge University Press: 13 October 2016
Abstract
Let 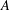 $A$ be a subset of
$A$ be a subset of  $\mathbb{N}$ , the set of all nonnegative integers. For an integer
$\mathbb{N}$ , the set of all nonnegative integers. For an integer 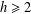 $h\geq 2$ , let
$h\geq 2$ , let 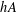 $hA$ be the set of all sums of
$hA$ be the set of all sums of 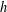 $h$ elements of
$h$ elements of 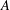 $A$ . The set
$A$ . The set 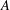 $A$ is called an asymptotic basis of order
$A$ is called an asymptotic basis of order 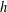 $h$ if
$h$ if 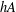 $hA$ contains all sufficiently large integers. Otherwise,
$hA$ contains all sufficiently large integers. Otherwise, 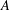 $A$ is called an asymptotic nonbasis of order
$A$ is called an asymptotic nonbasis of order 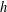 $h$ . An asymptotic nonbasis
$h$ . An asymptotic nonbasis 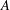 $A$ of order
$A$ of order 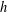 $h$ is called a maximal asymptotic nonbasis of order
$h$ is called a maximal asymptotic nonbasis of order 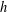 $h$ if
$h$ if 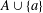 $A\cup \{a\}$ is an asymptotic basis of order
$A\cup \{a\}$ is an asymptotic basis of order  $h$ for every
$h$ for every 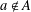 $a\notin A$ . In this paper, we construct a sequence of asymptotic nonbases of order
$a\notin A$ . In this paper, we construct a sequence of asymptotic nonbases of order  $h$ for each
$h$ for each  $h\geq 2$ , each of which is not a subset of a maximal asymptotic nonbasis of order
$h\geq 2$ , each of which is not a subset of a maximal asymptotic nonbasis of order 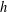 $h$ .
$h$ .
MSC classification
- Type
- Research Article
- Information
- Copyright
- © 2016 Australian Mathematical Publishing Association Inc.
References
- 1
- Cited by


