A monic polynomial 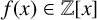$f(x)\in {\mathbb Z}[x]$ of degree N is called monogenic if $f(x)$ is irreducible over ${\mathbb Q}$ and $\{1,\theta ,\theta ^2,\ldots ,\theta ^{N-1}\}$ is a basis for the ring of integers of 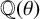${\mathbb Q}(\theta )$, where 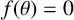$f(\theta )=0$. We prove that there exist exactly three distinct monogenic trinomials of the form 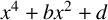$x^4+bx^2+d$ whose Galois group is the cyclic group of order 4. We also show that the situation is quite different when the Galois group is not cyclic.