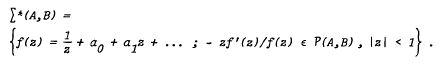Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-23T01:28:34.620Z Has data issue: false hasContentIssue false
  • English
  • Français

Meromorphic starlike univalent functions

Published online by Cambridge University Press:  17 April 2009

V. V. Anh  and
P. D. Tuan
V. V. Anh
Affiliation:
Department of Mathematics, Queensland Institute of Technology, P. O. Box 2434, Brisbane, 4001 Queensland, Australia.
P. D. Tuan
Affiliation:
First Interstate Bank of California, 600 South Spring Street, Los Angeles, CA90014, U.S.A.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let B be the class of functions ω(z) regular in |z| < 1 and satisfying ω(0) = 0, |ω(z)|<1 in |z|<1. We denote by P(A, B), −1 ≤ B < A ≤1, the class of functions p(z) = l+p1z+… regular in |z| < 1 and such that p(z) = [1+Aω(z)]/[1+Bω(z)] for some ω(z) ∈ Β. This paper establishes sharp lower and upper bounds on |z| = r<1 for the functional

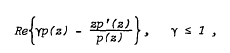
where p(z) varies in P(A, B). The results are then used to study certain geometric properties of the corresponding class of meromorphic starlike univalent functions

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 30 , Issue 3 , December 1984 , pp. 395 - 410
Copyright
Copyright © Australian Mathematical Society 1984

References

[1]Duren, P., “Subordination” (Lecture Notes in Mathematics, Springer-Verlag, 599, 1977).CrossRefGoogle Scholar
[2]Janowski, W., “Some extremal problems for certain families of analytic functions I”, Ann. Polon. Math., 28 (1973), 298–326.CrossRefGoogle Scholar
[3]Karunakaran, V., “On a class of meromorphic starlike functions in the unit disc”, Math. Chronicle, 4 (1976), 112–121.Google Scholar
[4]Padmanabhan, K.S., “On certain classes of starlike functions in the unit disk”, J. Indian Math. Soc., 32 (1968), 89–103.Google Scholar
[5]Pommerenke, C., “On meromorphic starlike functions”, Pacific J. Math., 13 (1963), 221–235.CrossRefGoogle Scholar
[6]Wiatrowski, P., “On the radius of convexity of some family of functions regular in the ring 0<|z|<1”, Ann. Polon. Math., 25 (1971), 85–98.Google Scholar