No CrossRef data available.
Article contents
THE MAXIMAL IDEAL IN THE SPACE OF OPERATORS ON 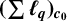 $\boldsymbol {(\sum {\ell }_{q})_{c_{0}}}$
$\boldsymbol {(\sum {\ell }_{q})_{c_{0}}}$
Published online by Cambridge University Press: 21 February 2022
Abstract
We study the isomorphic structure of
 $(\sum {\ell }_{q})_{c_{0}}\ (1< q<\infty )$
and prove that these spaces are complementably homogeneous. We also show that for any operator T from
$(\sum {\ell }_{q})_{c_{0}}\ (1< q<\infty )$
and prove that these spaces are complementably homogeneous. We also show that for any operator T from
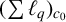 $(\sum {\ell }_{q})_{c_{0}}$
into
$(\sum {\ell }_{q})_{c_{0}}$
into
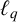 ${\ell }_{q}$
, there is a subspace X of
${\ell }_{q}$
, there is a subspace X of
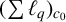 $(\sum {\ell }_{q})_{c_{0}}$
that is isometric to
$(\sum {\ell }_{q})_{c_{0}}$
that is isometric to
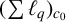 $(\sum {\ell }_{q})_{c_{0}}$
and the restriction of T on X has small norm. If T is a bounded linear operator on
$(\sum {\ell }_{q})_{c_{0}}$
and the restriction of T on X has small norm. If T is a bounded linear operator on
 $(\sum {\ell }_{q})_{c_{0}}$
which is
$(\sum {\ell }_{q})_{c_{0}}$
which is
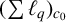 $(\sum {\ell }_{q})_{c_{0}}$
-strictly singular, then for any
$(\sum {\ell }_{q})_{c_{0}}$
-strictly singular, then for any
 $\epsilon>0$
, there is a subspace X of
$\epsilon>0$
, there is a subspace X of
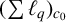 $(\sum {\ell }_{q})_{c_{0}}$
which is isometric to
$(\sum {\ell }_{q})_{c_{0}}$
which is isometric to
 $(\sum {\ell }_{q})_{c_{0}}$
with
$(\sum {\ell }_{q})_{c_{0}}$
with
 $\|T|_{X}\|<\epsilon $
. As an application, we show that the set of all
$\|T|_{X}\|<\epsilon $
. As an application, we show that the set of all
 $(\sum {\ell }_{q})_{c_{0}}$
-strictly singular operators on
$(\sum {\ell }_{q})_{c_{0}}$
-strictly singular operators on
 $(\sum {\ell }_{q})_{c_{0}}$
forms the unique maximal ideal of
$(\sum {\ell }_{q})_{c_{0}}$
forms the unique maximal ideal of
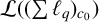 $\mathcal {L}((\sum {\ell }_{q})_{c_{0}})$
.
$\mathcal {L}((\sum {\ell }_{q})_{c_{0}})$
.
MSC classification
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 106 , Issue 2 , October 2022 , pp. 340 - 348
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
Footnotes
Bentuo Zheng’s research is supported in part by Simons Foundation Grant 585081.



