Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-25T16:10:06.176Z Has data issue: false hasContentIssue false
  • English
  • Français

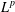$L^{p}$ REGULARITY OF THE WEIGHTED BERGMAN PROJECTION ON THE FOCK–BARGMANN–HARTOGS DOMAIN

Part of: Holomorphic functions of several complex variables

Published online by Cambridge University Press:  08 January 2020

LE HE Open the ORCID record for LE HE [Opens in a new window] ,
YANYAN TANG Open the ORCID record for YANYAN TANG [Opens in a new window]  and
ZHENHAN TU Open the ORCID record for ZHENHAN TU [Opens in a new window]
LE HE
Affiliation:
School of Mathematics and Statistics,Wuhan University, Wuhan, Hubei430072, PR China email [email protected]
YANYAN TANG
Affiliation:
School of Mathematics and Statistics,Wuhan University, Wuhan, Hubei430072, PR China email [email protected]
ZHENHAN TU*
Affiliation:
School of Mathematics and Statistics,Wuhan University, Wuhan, Hubei430072, PR China email [email protected]
*
✉[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The Fock–Bargmann–Hartogs domain 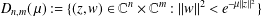$D_{n,m}(\,\unicode[STIX]{x1D707}):=\{(z,w)\in \mathbb{C}^{n}\times \mathbb{C}^{m}:\Vert w\Vert ^{2}<e^{-\unicode[STIX]{x1D707}\Vert z\Vert ^{2}}\}$, where $\unicode[STIX]{x1D707}>0$, is an unbounded strongly pseudoconvex domain with smooth real-analytic boundary. We compute the weighted Bergman kernel of $D_{n,m}(\,\unicode[STIX]{x1D707})$ with respect to the weight $(-\unicode[STIX]{x1D70C})^{\unicode[STIX]{x1D6FC}}$, where $\unicode[STIX]{x1D70C}(z,w):=\Vert w\Vert ^{2}-e^{-\unicode[STIX]{x1D707}\Vert z\Vert ^{2}}$ and 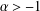$\unicode[STIX]{x1D6FC}>-1$. Then, for 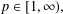$p\in [1,\infty ),$ we show that the corresponding weighted Bergman projection 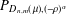$P_{D_{n,m}(\,\unicode[STIX]{x1D707}),(-\unicode[STIX]{x1D70C})^{\unicode[STIX]{x1D6FC}}}$ is unbounded on 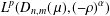$L^{p}(D_{n,m}(\,\unicode[STIX]{x1D707}),(-\unicode[STIX]{x1D70C})^{\unicode[STIX]{x1D6FC}})$, except for the trivial case $p=2$. This gives an example of an unbounded strongly pseudoconvex domain whose ordinary Bergman projection is 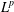$L^{p}$ irregular when 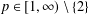$p\in [1,\infty )\setminus \{2\}$, in contrast to the well-known positive 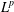$L^{p}$ regularity result on a bounded strongly pseudoconvex domain.

Keywords

Fock–Bargmann–Hartogs domainLp regularityweighted Bergman kernelweighted Bergman projection

MSC classification

Primary: 32A36: Bergman spaces
Secondary: 32A25: Integral representations; canonical kernels (Szegő, Bergman, etc.) 32A07: Special domains (Reinhardt, Hartogs, circular, tube)
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 102 , Issue 2 , October 2020 , pp. 282 - 292
Check for updates
Copyright
© 2020 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The project is supported by the National Natural Science Foundation of China (No. 11671306).

References

Barrett, D. and Şahutoğlu, S., ‘Irregularity of the Bergman projection on worm domains in ℂn’, Michigan Math. J. 61(1) (2013), 187–189.CrossRefGoogle Scholar
Bi, E. C., Feng, Z. M. and Tu, Z. H., ‘Balanced metrics on the Fock–Bargmann–Hartogs domains’, Ann. Global Anal. Geom. 49 (2016), 349–359.CrossRefGoogle Scholar
Bommier-Hato, H., Engliš, M. and Youssfi, E. H., ‘Bergman-type projection in generalized Fock spaces’, J. Math. Anal. Appl. 389(2) (2012), 1086–1104.CrossRefGoogle Scholar
Chakrabarti, D. and Zeytuncu, Y., ‘L p mapping properties of the Bergman projection on the Hartogs triangle’, Proc. Amer. Math. Soc. 144(4) (2016), 1643–1653.CrossRefGoogle Scholar
Charpentier, P. and Dupain, Y., ‘Estimates for the Bergman and Szegö projections for pseudoconvex domains of finite type with locally diagonalizable Levi form’, Publ. Mat. 50(2) (2006), 413–446.CrossRefGoogle Scholar
Chen, L., ‘Weighted Bergman projections on the Hartogs triangle’, J. Math. Anal. Appl. 446(1) (2017), 546–567.CrossRefGoogle Scholar
Čučković, Ž. and Zeytuncu, Y. E., ‘Mapping properties of weighted Bergman projection operators on Reinhardt domain’, Proc. Amer. Math. Soc. 144(3) (2015), 537–552.Google Scholar
Edholm, L. D. and McNeal, J. D., ‘The Bergman projection on fat Hartogs triangles: L p boundedness’, Proc. Amer. Math. Soc. 144(5) (2016), 2185–2196.CrossRefGoogle Scholar
Huo, Z., ‘L p estimates for the Bergman projection on some Reinhardt domains’, Proc. Amer. Math. Soc. 146(6) (2018), 2541–2553.CrossRefGoogle Scholar
Janson, S., Peetre, J. and Rochberg, R., ‘Hankel forms and the Fock space’, Rev. Mat. Iberoam. 3 (1987), 61–138.CrossRefGoogle Scholar
Kim, H., Ninh, V. T. and Yamamori, A., ‘The automorphism group of a certain unbounded non-hyperbolic domain’, J. Math. Anal. Appl. 409(2) (2014), 637–642.CrossRefGoogle Scholar
Krantz, S. and Peloso, M., ‘The Bergman kernel and projection on non-smooth worm domains’, Houston J. Math. 34 (2008), 873–950.Google Scholar
Lanzani, L. and Stein, E. M., ‘The Bergman projection in L p for domains with minimal smoothness’, Illinois J. Math. 56(1) (2013), 127–154.CrossRefGoogle Scholar
Ligocka, E., ‘On the Forelli–Rudin construction and weighted Bergman projection’, Studia Math. 94(3) (1989), 257–272.CrossRefGoogle Scholar
McNeal, J. D., ‘The Bergman projection as a singular integral operator’, J. Geom. Anal. 4(1) (1994), 91–103.CrossRefGoogle Scholar
McNeal, J. D., ‘Estimates on the Bergman kernels of convex domains’, Adv. Math. 109(1) (1994), 108–139.CrossRefGoogle Scholar
McNeal, J. D. and Stein, E. M., ‘Mapping properties of the Bergman projection on convex domains of finite type’, Duke Math. J. 73(1) (1994), 177–199.CrossRefGoogle Scholar
Pasternak-Winiarski, Z., ‘On the dependence of the reproducing kernel on the weight of integration’, J. Funct. Anal. 94(1) (1990), 110–134.CrossRefGoogle Scholar
Phong, D. H. and Stein, E. M., ‘Estimates for the Bergman and Szegö projections on strongly pseudoconvex domains’, Duke Math. J. 44(3) (1977), 695–704.CrossRefGoogle Scholar
Rudin, W., Function Theory in the Unit Ball of ℂn, reprint of the 1980 edition (Springer, Berlin, 2008).Google Scholar
Tu, Z. H. and Wang, L., ‘Rigidity of proper holomorphic mappings between certain unbounded non-hyperbolic domains’, J. Math. Anal. Appl. 419 (2014), 703–714.CrossRefGoogle Scholar
Yamamori, A., ‘The Bergman kernel of the Fock–Bargmann–Hartogs domain and the polylogarithm function’, Complex Var. Elliptic Equ. 58(6) (2013), 783–793.CrossRefGoogle Scholar
Zeytuncu, Y. E., ‘L p regularity of weighted Bergman projections’, Trans. Amer. Math. Soc. 365(6) (2013), 2959–2976.CrossRefGoogle Scholar