Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-20T06:20:32.043Z Has data issue: false hasContentIssue false
  • English
  • Français

Local analytic structure in certain commutative topological algebras

Published online by Cambridge University Press:  17 April 2009

R.J. Loy
R.J. Loy
Affiliation:
Department of Pure Mathematics, School of General Studies, Australian National University, Canberra, ACT.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let B be a topological algebra with Fréchet space topology, A an algebra with locally convex topology and 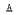an algebra of formal power series over A in n commuting indeterminates which carries a Fréchet space topology. In a previous paper the author showed, for the case n = 1, that a homomorphism of B into whose range contains polynomials is necessarily continuous provided the coordinate projections of 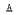into A satisfy a certain equicontinuity condition. This result is here extended to the case of general n, and also to weaker topological assumptions.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 6 , Issue 2 , April 1972 , pp. 161 - 167
Copyright
Copyright © Australian Mathematical Society 1972

References

[1]Carpenter, Ronn, “Singly generated homogeneous F-algebras”, Trans. Amer. Math. Soc. 150 (1970), 457–468.Google Scholar
[2]Clayton, Dennis, “A local characterization of analytic structure in a commutative Banach algebra”, preprint.Google Scholar
[3]Edwards, R.E., Functional analysis (Holt, Rinehart and Winston, New York, Chicago, San Francisco, Toronto, London, 1965).Google Scholar
[4]Gunning, Robert C., Rossi, Hugo, Analytic functions of several complex variables (Prentice-Hall, Englewood Cliffs, New Jersey, 1965).Google Scholar
[5]Loy, R.J., “Uniqueness of the Fréchet space topology on certain topological algebras”, Bull. Austral. Math. Soc. 4 (1971), 1–7.Google Scholar
[6]Michael, Ernest A., Locally multiplicatively-convex topological algebras (Mem. Amer. Math. Soc. 11. Amer. Math. Soc., Providence, Rhode Island, 1952).Google Scholar
[7]Rickart, C.E., “Analytic phenomena in general function algebras”, Pacific J. Math. 18 (1966), 361–377.Google Scholar