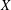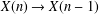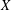Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bačák, Miroslav
and
Kovalev, Leonid V.
2016.
Lipschitz Retractions in Hadamard Spaces via Gradient Flow Semigroups.
Canadian Mathematical Bulletin,
Vol. 59,
Issue. 4,
p.
673.
Akofor, Earnest
2019.
On Lipschitz retraction of finite subsets of normed spaces.
Israel Journal of Mathematics,
Vol. 234,
Issue. 2,
p.
777.
Castañeda-Alvarado, Enrique
Orozco-Zitli, Fernando
and
Reyes-Quiroz, Mónica A.
2021.
Lipschitz retractions on symmetric products of trees.
Indian Journal of Pure and Applied Mathematics,
Vol. 52,
Issue. 4,
p.
1072.
Kovalev, Leonid V.
2022.
Lipschitz Clustering in Metric Spaces.
The Journal of Geometric Analysis,
Vol. 32,
Issue. 7,
Bačák, Miroslav
2023.
Old and new challenges in Hadamard spaces.
Japanese Journal of Mathematics,
Vol. 18,
Issue. 2,
p.
115.
Kovalev, Leonid V.
2025.
Anti-absorbing ternary operations on metric spaces.
Topology and its Applications,
Vol. 360,
Issue. ,
p.
109153.
Sofi, M.
2025.
Geometry, Groups and Mathematical Philosophy.
Vol. 811,
Issue. ,
p.
183.