Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-23T02:12:27.091Z Has data issue: false hasContentIssue false
  • English
  • Français

LINEAR QUOTIENTS AND MULTIGRADED SHIFTS OF BOREL IDEALS

Part of: Algebraic combinatorics Commutative algebra: Homological methods Arithmetic rings and other special rings General commutative ring theory

Published online by Cambridge University Press:  30 January 2019

SHAMILA BAYATI Open the ORCID record for SHAMILA BAYATI [Opens in a new window] ,
IMAN JAHANI Open the ORCID record for IMAN JAHANI [Opens in a new window]  and
NADIYA TAGHIPOUR Open the ORCID record for NADIYA TAGHIPOUR [Opens in a new window]
SHAMILA BAYATI*
Affiliation:
Faculty of Mathematics and Computer Science, Amirkabir University of Technology (Tehran Polytechnic), 424 Hafez Ave., Tehran 15914, Iran email [email protected]
IMAN JAHANI
Affiliation:
Faculty of Mathematics and Computer Science, Amirkabir University of Technology (Tehran Polytechnic), 424 Hafez Ave., Tehran 15914, Iran email [email protected]
NADIYA TAGHIPOUR
Affiliation:
Faculty of Mathematics and Computer Science, Amirkabir University of Technology (Tehran Polytechnic), 424 Hafez Ave., Tehran 15914, Iran email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We investigate whether the property of having linear quotients is inherited by ideals generated by multigraded shifts of a Borel ideal and a squarefree Borel ideal. We show that the ideal generated by the first multigraded shifts of a Borel ideal has linear quotients, as do the ideal generated by the 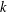$k$th multigraded shifts of a principal Borel ideal and an equigenerated squarefree Borel ideal for each 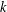$k$. Furthermore, we show that equigenerated squarefree Borel ideals share the property of being squarefree Borel with the ideals generated by multigraded shifts.

Keywords

Borel idealfree resolutionlinear quotientmultigraded shiftsquarefree Borel ideal

MSC classification

Primary: 13D02: Syzygies, resolutions, complexes
Secondary: 13A02: Graded rings 13F20: Polynomial rings and ideals; rings of integer-valued polynomials 05E40: Combinatorial aspects of commutative algebra
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 100 , Issue 1 , August 2019 , pp. 48 - 57
Copyright
© 2019 Australian Mathematical Publishing Association Inc. 

References

Aramova, A., Herzog, J. and Hibi, T., ‘Squarefree lexsegment ideals’, Math. Z. 228 (1998), 353–378.Google Scholar
Bayati, S., ‘Multigraded shifts of matroidal ideals’, Arch. Math. 111(3) (2018), 239–246.Google Scholar
Bayer, D., Peeva, I. and Sturmfels, B., ‘Monomial resolutions’, Math. Res. Lett. 5 (1998), 31–46.Google Scholar
Bruns, W., Krattenthaler, C. and Uliczka, J., ‘Stanley decompositions and Hilbert depth in the Koszul complex’, J. Commut. Algebra 2(3) (2010), 327–357.Google Scholar
Eliahou, S. and Kervaire, M., ‘Minimal resolution of some monomial ideals’, J. Algebra 129(1) (1990), 1–25.Google Scholar
Fløystad, G., ‘Cellular resolutions of Cohen–Macaulay monomial ideals’, J. Commut. Algebra 1 (2009), 57–89.Google Scholar
Fløystad, G., ‘The linear space of Betti diagrams of multigraded Artinian modules’, Math. Res. Lett. 17(5) (2010), 943–958.Google Scholar
Francisco, C., Mermin, J. and Schweig, J., ‘Borel generators’, J. Algebra 332 (2011), 522–542.Google Scholar
Galligo, A., ‘À propos du théorème de préparation de Weierstrass’, in: Fonctions de plusieurs variables complexes, Lecture Notes in Mathematics, 409 (Springer, Berlin–Heidelberg, 1974), 543–579.Google Scholar
Gasharova, V., Hibi, T. and Peeva, I., ‘Resolutions of a-stable ideals’, J. Algebra 254(2) (2002), 375–394.Google Scholar
Herzog, J., Hibi, T. and Zheng, X., ‘Dirac’s theorem on chordal graphs and Alexander duality’, European J. Combin. 25(7) (2004), 949–960.Google Scholar
Iyengar, S., ‘Shifts in resolutions of multigraded modules’, Math. Proc. Cambridge Philos. Soc. 121(3) (1997), 437–441.Google Scholar
Mapes, S. and Piechnik, L., ‘Constructing monomial ideals with a given minimal resolution’, Rocky Mountain J. Math. 47(6) (2017), 1963–1985.Google Scholar
Miller, E. and Sturmfels, B., Combinatorial Commutative Algebra, Graduate Texts in Mathematics, 227 (Springer, New York, 2005).Google Scholar
Peeva, I. and Velasco, M., ‘Frames and degenerations of monomial resolutions’, Trans. Amer. Math. Soc. 363 (2011), 2029–2046.Google Scholar