Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-06T02:04:29.840Z Has data issue: false hasContentIssue false
  • English
  • Français

Laurent expansion of Dirichlet series

Published online by Cambridge University Press:  17 April 2009

U. Balakrishnan
U. Balakrishnan
Affiliation:
School of Mathematics, Tata Institute of Fundamental Research, Homi Bhabha Road, Bombay – 5, India.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 〈an〉 be an increasing sequence of real numbers and 〈bn a sequence of positive real numbers. We deal here with the Dirichlet series 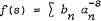and its Laurent expansion at the abscissa of convergence, λ, say. When an and bn behave like

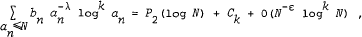
as N → ∞, where P2(x) is a certain polynomial, we obtain the Laurent expansion of f (s) at s = λ, namely

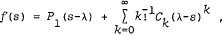
where P1(x) is a polynomial connected with P2(x) above. Also, the connection between P1 and P2 is made intuitively transparent in the proof.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 33 , Issue 3 , June 1986 , pp. 351 - 357
Copyright
Copyright © Australian Mathematical Society 1986

References

[1]Balakrishnan, U., ‘On the Laurent expansion of ζ(s, a) at s = 1’, J. Indian Math. Soc. 46 (1982), 181–187.Google Scholar
[2]Berndt, B.C., ‘On the Hurwitz zeta function’, Rocky mountain J. Math., 2 (1972), 151–157.CrossRefGoogle Scholar
[3]Briggs, W.E. and Buschman, R. G., ‘The power series coefficients of functions defined by Dirichlet series’, Illinois J. Math., 5 (1961), 43–44.CrossRefGoogle Scholar
[4]Knopfmacher, J., ‘Generalised Euler constants’, Proc. Edinburgh Math. Soc., 21 (1978), 25–32.CrossRefGoogle Scholar