Hostname: page-component-f554764f5-246sw Total loading time: 0 Render date: 2025-04-11T03:42:57.618Z Has data issue: false hasContentIssue false
  • English
  • Français

GROUPS SATISFYING THE DOUBLE CHAIN CONDITION ON ABELIAN SUBGROUPS

Part of: Structure and classification of infinite or finite groups

Published online by Cambridge University Press:  12 September 2018

MATTIA BRESCIA  and
ALESSIO RUSSO Open the ORCID record for ALESSIO RUSSO [Opens in a new window]
MATTIA BRESCIA
Affiliation:
Dipartimento di Matematica e Fisica, Università della Campania ‘Luigi Vanvitelli’, Viale Lincoln 5, Caserta, Italy email [email protected]
ALESSIO RUSSO*
Affiliation:
Dipartimento di Matematica e Fisica, Università della Campania ‘Luigi Vanvitelli’, Viale Lincoln 5, Caserta, Italy email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

If 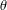$\unicode[STIX]{x1D703}$ is a subgroup property, a group $G$ is said to satisfy the double chain condition on $\unicode[STIX]{x1D703}$-subgroups if it admits no infinite double sequences

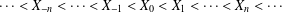$$\begin{eqnarray}\cdots <X_{-n}<\cdots <X_{-1}<X_{0}<X_{1}<\cdots <X_{n}<\cdots\end{eqnarray}$$
consisting of $\unicode[STIX]{x1D703}$-subgroups. We describe the structure of generalised radical groups satisfying the double chain condition on abelian subgroups.

Keywords

double chain conditionabelian subgroup

MSC classification

Primary: 20E15: Chains and lattices of subgroups, subnormal subgroups
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 99 , Issue 2 , April 2019 , pp. 212 - 218
Copyright
© 2018 Australian Mathematical Publishing Association Inc. 

Footnotes

The authors are members of GNSAGA-INdAM and this work was carried out within the ADV-AGTA project.

References

Baer, R., ‘Polyminimaxgruppen’, Math. Ann. 175 (1968), 1–43.Google Scholar
Brescia, M. and de Giovanni, F., ‘Groups satisfying the double chain condition on subnormal subgroups’, Ric. Mat. 65 (2016), 255–261.Google Scholar
Brescia, M. and de Giovanni, F., ‘Groups satisfying the double chain condition on non-pronormal subgroups’, Riv. Mat. Univ. Parma 8 (2017), 353–366.Google Scholar
De Mari, F. and de Giovanni, F., ‘Double chain conditions for infinite groups’, Ric. Mat. 54 (2005), 59–70.Google Scholar
Lennox, J. C. and Robinson, D. J. S., The Theory of Infinite Soluble Groups (Clarendon Press, Oxford, 2004).Google Scholar
Mal’cev, A. I., ‘On certain classes of infinite soluble groups’, Mat. Sb. 28 (1951), 567–588.Google Scholar
Robinson, D. J. S., Finiteness Conditions and Generalized Soluble Groups (Springer, Berlin, 1972).Google Scholar
Schmidt, O. J., ‘Infinite soluble groups’, Mat. Sb. 17 (1945), 145–162.Google Scholar
Shores, T. S., ‘A chain condition for groups’, Rocky Mountain J. Math. 3 (1973), 83–89.Google Scholar
Šunkov, V. P., ‘Locally finite groups with a minimality condition for abelian subgroups’, Algebra Logic 9 (1970), 350–370.Google Scholar
Zaicev, D. I., ‘On the theory of minimax groups’, Ukrainian Math. J. 23 (1971), 536–542.Google Scholar