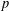Hostname: page-component-669899f699-8p65j Total loading time: 0 Render date: 2025-04-25T15:05:58.921Z Has data issue: false hasContentIssue false
  • English
  • Français

FINITE $p$-GROUPS ALL OF WHOSE NONNORMAL SUBGROUPS HAVE BOUNDED NORMAL CORES

Part of: Representation theory of groups Special aspects of infinite or finite groups

Published online by Cambridge University Press:  18 July 2019

DONGFANG YANG Open the ORCID record for DONGFANG YANG [Opens in a new window] ,
LIJIAN AN Open the ORCID record for LIJIAN AN [Opens in a new window]  and
HENG LV Open the ORCID record for HENG LV [Opens in a new window]
DONGFANG YANG*
Affiliation:
School of Mathematics and Statistics, Southwest University, Chongqing, 400715, PR China email [email protected]
LIJIAN AN
Affiliation:
Department of Mathematics, Shanxi Normal University, Linfen, Shanxi, 041004, PR China email [email protected]
HENG LV
Affiliation:
School of Mathematics and Statistics, Southwest University, Chongqing, 400715, PR China email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Given a positive integer $m$, a finite 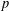$p$-group 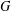$G$ is called a 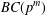$BC(p^{m})$-group if 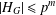$|H_{G}|\leq p^{m}$ for every nonnormal subgroup 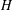$H$ of $G$, where 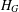$H_{G}$ is the normal core of 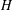$H$ in 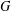$G$. We show that 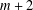$m+2$ is an upper bound for the nilpotent class of a finite 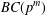$BC(p^{m})$-group and obtain a necessary and sufficient condition for a 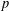$p$-group to be of maximal class. We also classify the $BC(p)$-groups.

Keywords

normal corenon-Dedekindian groupmaximal class

MSC classification

Primary: 20D15: Nilpotent groups, $p$-groups
Secondary: 20F19: Generalizations of solvable and nilpotent groups
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 101 , Issue 2 , April 2020 , pp. 255 - 265
Copyright
© 2019 Australian Mathematical Publishing Association Inc.

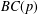Footnotes

This work was supported by the National Natural Science Foundation of China (11671324, 11471266).

References

Berkovich, Y., Groups of Prime Power Order, Vol. 1 (Walter de Gruyter, Berlin, 2008).Google Scholar
Buckley, J., Lennox, J. C., Neumann, B. H., Smith, H. and Wiegold, J., ‘Groups with all subgroups normal-by-finite’, J. Aust. Math. Soc. Ser. A 59 (1995), 384–398.Google Scholar
Cutolo, G., Khukhro, E. I., Lennox, J. C., Rinauro, S., Smith, H. and Weigold, J., ‘Finite core-p p-groups’, J. Algebra 188 (1997), 701–719.Google Scholar
Cutolo, G., Smith, H. and Wiegold, J., ‘On core-2-groups’, J. Algebra 237 (2001), 813–841.Google Scholar
Fernández-Alcober, G. A. and Moretó, A., ‘Groups with extreme character degrees and their normal subgroups’, Trans. Amer. Math. Soc. 353 (2001), 2271–2292.Google Scholar
Isaacs, I. M., Character Theory of Finite Groups (Academic Press, New York, 1976).Google Scholar
Janko, Z., ‘Some peculiar minimal situations by finite p-groups’, Glas. Mat. Ser. III 43(63) (2008), 111–120.Google Scholar
Lv, H., Zhou, W. and Guo, X., ‘Finite 2-groups with index of every cyclic subgroup in its normal closure no greater than 4’, J. Algebra 342 (2011), 256–264.Google Scholar
Lv, H., Zhou, W. and Liu, J., ‘On finite core-p 2  p-groups’, J. Pure Appl. Algebra 222(9) (2018), 2505–2513.Google Scholar
Lv, H., Zhou, W. and Yu, D., ‘Some finite p-groups with bounded index of every cyclic subgroup in its normal closure’, J. Algebra 338 (2011), 169–179.Google Scholar
Neumann, B. H., ‘Groups with finite classes of conjugate subgroups’, Math. Z. 63 (1955), 76–96.Google Scholar
Passman, D. S., ‘Nonnormal subgroups of p-groups’, J. Algebra 15(3) (1970), 352–370.Google Scholar
Zhang, J. and Zhang, Q., ‘Finite p-groups all of whose minimal nonnormal subgroups possess large normal closures’, Comm. Algebra 44 (2016), 628–638.Google Scholar
Zhao, L. and Guo, X., ‘Finite p-groups in which the normal closures of the nonnormal cyclic subgroups have small index’, J. Algebra Appl. 13(2) 1350087 (2014), 7 pages.Google Scholar