Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-05T05:38:02.260Z Has data issue: false hasContentIssue false
  • English
  • Français

Finite groups with large centralizers

Published online by Cambridge University Press:  17 April 2009

Edward A. Bertram  and
Marcel Herzog
Edward A. Bertram
Affiliation:
Department of MathematicsUniversity of Hawaii-ManoaHonolulu, Hawaii. 96822.
Marcel Herzog
Affiliation:
School of Mathematics Tel-Aviv UniversityRamat-Aviv, 69978 Tel-Aviv. Israel.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

It is known that a finite non-abelian group G has a proper centralizer of order 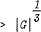if, for example, |G| is even and |Z(G)| is odd, or whenever G is solvable. Often the exponent 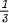can be improved to 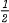, for example when G is supersolvable, or metabelian, or |G = pαqβ. Here we show more generally that this improvement is possible in many situations where G is factorizable into the product of two subgroups. In particular, much more evidence is presented to support the conjecture that some proper centralizer has order 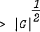whenever G is a finite non-abelian solvable group.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 32 , Issue 3 , December 1985 , pp. 399 - 414
Copyright
Copyright © Australian Mathematical Society 1985

References

[1]Baer, R., “Partitionen endlicher Gruppen”, Math. Zeit. 75 (1961) 333–372.Google Scholar
[2]Bertram, E. A., “Large centralizers in finite solvable groups”, Israel J. Math. 47 (1984), 335–344.CrossRefGoogle Scholar
[3]Gorenstein, D., “Finite Groups”, (Harper and Row, New York, 1968).Google Scholar
[4]Huppert, B., “Endlicher Gruppen”, Vol. I, (springer-Verlag, Berlin, New York, 1967).Google Scholar
[5]Rocke, D. M., “Groups with abelian centralizers”, Unpub. Part of Ph.D. Diss., Univ. of Illinois, 1972.Google Scholar
[6]Schmidt, R., “Zentralisatorverbände endlicher Gruppen”, Sem. Math. De Univ. Padova 44 (1970), 97–111.Google Scholar
[7]Suzuki, M., “Group Theory I”, Springer-Verlag, Berlin-Heidelberg-New York, 1982.CrossRefGoogle Scholar