Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-01-22T13:53:22.012Z Has data issue: false hasContentIssue false
  • English
  • Français

Extreme points in spaces between Dirichlet and Vanishing Mean Oscillation

Published online by Cambridge University Press:  17 April 2009

K. J. Wirths  and
J. Xiao
K. J. Wirths
Affiliation:
Institute of Analysis, TU-Braunschweig, D-38106 Braunschweig, Germany, e-mail: [email protected]
J. Xiao
Affiliation:
Department of Mathematics and Statistics, Memorial University of Newfoundland, St. John's, NL A1C 5S7, Canada, e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

For p ∈ (0, ∞) define Qp0(∂Δ) as the space of all Lebesgue measurable complex-valued functions f; on the unit circle ∂Δ for which ∫∂Δf;(z)|dz|/(2π) = 0 and

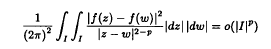
as the open subarc I of ∂Δ varies. Note that each Qp,0(∂Δ) lies between the Dirichlet space and Sarason's vanishing mean oscillation space. This paper determines the extreme points of the closed unit ball of Qp,0(∂Δ) equipped with an appropriate norm.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 67 , Issue 3 , June 2003 , pp. 365 - 375
Copyright
Copyright © Australian Mathematical Society 2003

References

[1]Aulaskar, R., Xiao, J. and Zhao, R., ‘On subspaces and subsets of BMOA and UBC’, Analysis 15 (1995), 101–121.CrossRefGoogle Scholar
[2]Axler, S. and Shields, A., ‘Extreme points in VMO and BMO’, Indiana Univ. Math. J. 31 (1982), 1–6.CrossRefGoogle Scholar
[3]Essén, M. and Xiao, J., ‘Some results on Qp spaces, 0 < p < 1’, J. Reine Angew. Math. 485 (1997), 173–195.Google Scholar
[4]Essén, M., Janson, S., Peng, L. and Xiao, J., ‘Q spaces of several real variables’, Indiana Univ. Math. J. 49 (2000), 576–615.CrossRefGoogle Scholar
[5]John, F. and Nirenberg, L., ‘On functions of bounded mean oscillation’, Common. Pure. Appl. Math. 14 (1961), 415–426.CrossRefGoogle Scholar
[6]Nicolau, A. and Xiao, J., ‘Bounded functions in Möbius invariant Dirichlet spaces’, J. Funct. Anal. 150 (1997), 383–425.CrossRefGoogle Scholar
[7]Rudin, W., Functional analysis (McGraw-Hill, N.J., 1973).Google Scholar
[8]Sarason, D., ‘Functions of vanishing mean oscillation’, Trans. Amer. Math. Soc. 207 (1975), 391–405.CrossRefGoogle Scholar
[9]Xiao, J., ‘Some essential properties of Qp (∂Δ)-spaces’, J. Fourier Anal. Appl. 6 (2000), 311–323.CrossRefGoogle Scholar