Published online by Cambridge University Press: 07 April 2010
Given a finite field 𝔽p={0,…,p−1} of p elements, where p is a prime, we consider the distribution of elements in the orbits of a transformation ξ↦ψ(ξ) associated with a rational function ψ∈𝔽p(X). We use bounds of exponential sums to show that if N≥p1/2+ε for some fixed ε then no N distinct consecutive elements of such an orbit are contained in any short interval, improving the trivial lower bound N on the length of such intervals. In the case of linear fractional functions 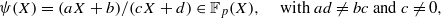 we use a different approach, based on some results of additive combinatorics due to Bourgain, that gives a nontrivial lower bound for essentially any admissible value of N.
we use a different approach, based on some results of additive combinatorics due to Bourgain, that gives a nontrivial lower bound for essentially any admissible value of N.
During the preparation of this paper, the first author was supported in part by Spain Ministry of Education and Science Grant MTM2007-67088 and the second author by the Australian Research Council Grant DP0556431.