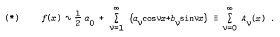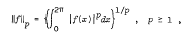Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Sahney, Badri N.
and
Gopal Rao, V. Venu
1972.
Error bounds in the approximation of functions: Corrigenda and an acknowledgement.
Bulletin of the Australian Mathematical Society,
Vol. 6,
Issue. 3,
p.
480.
Holland, A. S. B.
1981.
A Survey of Degree of Approximation of Continuous Functions.
SIAM Review,
Vol. 23,
Issue. 3,
p.
344.
Mohapatra, R. N.
and
Russell, D. C.
1983.
Some direct and inverse theorems in approximation of functions.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 34,
Issue. 2,
p.
143.
Das, G.
Ghosh, Tulika
and
Ray, B. K.
1996.
Degree of approximation of functions by their fourier series in the generalized Hölder metric.
Proceedings of the Indian Academy of Sciences - Section A,
Vol. 106,
Issue. 2,
p.
139.
Chandra, Prem
2002.
Trigonometric approximation of functions in Lp-norm.
Journal of Mathematical Analysis and Applications,
Vol. 275,
Issue. 1,
p.
13.
Mohapatra, Ram N.
and
Szal, Bogdan
2018.
On trigonometric approximation of functions in the Lq norm.
Demonstratio Mathematica,
Vol. 51,
Issue. 1,
p.
17.
Das, Shilpa
and
Dutta, Hemen
2021.
Degree of approximation of signals in certain Lipschitz classes by the Zweier–Euler product summability method of Fourier series.
Mathematical Methods in the Applied Sciences,
Vol. 44,
Issue. 6,
p.
4881.
Chandra, Prem
and
Karanjgaokar, Varsha
2022.
Trigonometric approximation of functions in $$L_1$$-norm.
Periodica Mathematica Hungarica,
Vol. 84,
Issue. 2,
p.
177.