Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sun, Taixiang
Chen, Zhanhe
Liu, Xinhe
and
Xi, Hongjian
2014.
Equicontinuity of dendrite maps.
Chaos, Solitons & Fractals,
Vol. 69,
Issue. ,
p.
10.
Sun, Tai Xiang
Su, Guang Wang
Xi, Hong Jian
and
Kong, Xin
2017.
Equicontinuity of maps on a dendrite with finite branch points.
Acta Mathematica Sinica, English Series,
Vol. 33,
Issue. 8,
p.
1125.
Camargo, Javier
Rincón, Michael
and
Uzcátegui, Carlos
2019.
Equicontinuity of maps on dendrites.
Chaos, Solitons & Fractals,
Vol. 126,
Issue. ,
p.
1.
Su, Guangwang
Sun, Taixiang
Han, Caihong
and
Qin, Bin
2020.
Characteristic of pointwise-recurrent maps on a dendrite.
Journal of Dynamical Systems and Geometric Theories,
Vol. 18,
Issue. 1,
p.
1.
Camargo, Javier
and
Cancino, Johan
2020.
The ω-limit function on dendrites.
Topology and its Applications,
Vol. 282,
Issue. ,
p.
107320.
Salem, Aymen Haj
Hattab, Hawete
and
Rejeiba, Tarek
2021.
Equicontinuous local dendrite maps.
Applied General Topology,
Vol. 22,
Issue. 1,
p.
67.
Abdelli, Hafedh
Askri, Ghassen
and
Kedim, Imed
2021.
Equicontinuity of Maps on Local Dendrites.
Qualitative Theory of Dynamical Systems,
Vol. 20,
Issue. 3,
Camargo, Javier
Cancino, Johan
and
Islas, Carlos
2024.
On the functions ωf
and Ω
f
.
Mathematica Slovaca,
Vol. 74,
Issue. 3,
p.
747.
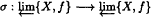 is a periodic map.
is a periodic map.