Published online by Cambridge University Press: 10 April 2014
A Brahmagupta quadrilateral is a cyclic quadrilateral whose sides, diagonals and area are all integer values. In this article, we characterise the notions of Brahmagupta, introduced by K. R. S. Sastry [‘Brahmagupta quadrilaterals’, Forum Geom. 2 (2002), 167–173], by means of elliptic curves. Motivated by these characterisations, we use Brahmagupta quadrilaterals to construct infinite families of elliptic curves with torsion group 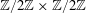 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathbb{Z}/2\mathbb{Z}\times \mathbb{Z}/2\mathbb{Z}$ having ranks (at least) four, five and six. Furthermore, by specialising we give examples from these families of specific curves with rank nine.
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathbb{Z}/2\mathbb{Z}\times \mathbb{Z}/2\mathbb{Z}$ having ranks (at least) four, five and six. Furthermore, by specialising we give examples from these families of specific curves with rank nine.