Published online by Cambridge University Press: 01 March 2022
Let
 $[t]$
be the integral part of the real number t. We study the distribution of the elements of the set
$[t]$
be the integral part of the real number t. We study the distribution of the elements of the set
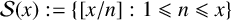 $\mathcal {S}(x) := \{[{x}/{n}] : 1\leqslant n\leqslant x\}$
in the arithmetical progression
$\mathcal {S}(x) := \{[{x}/{n}] : 1\leqslant n\leqslant x\}$
in the arithmetical progression
 $\{a+dq\}_{d\geqslant 0}$
. We give an asymptotic formula
$\{a+dq\}_{d\geqslant 0}$
. We give an asymptotic formula
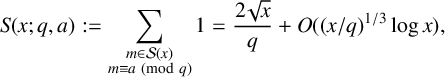 $$ \begin{align*} S(x; q, a) := \sum_{\substack{m\in \mathcal{S}(x)\\ m\equiv a \pmod q}} 1 = \frac{2\sqrt{x}}{q} + O((x/q)^{1/3}\log x), \end{align*} $$
$$ \begin{align*} S(x; q, a) := \sum_{\substack{m\in \mathcal{S}(x)\\ m\equiv a \pmod q}} 1 = \frac{2\sqrt{x}}{q} + O((x/q)^{1/3}\log x), \end{align*} $$
which holds uniformly for
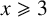 $x\geqslant 3$
,
$x\geqslant 3$
,
 $1\leqslant q\leqslant x^{1/4}/(\log x)^{3/2}$
and
$1\leqslant q\leqslant x^{1/4}/(\log x)^{3/2}$
and
 $1\leqslant a\leqslant q$
, where the implied constant is absolute. The special case
$1\leqslant a\leqslant q$
, where the implied constant is absolute. The special case
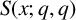 $S(x; q, q)$
confirms a recent numerical test of Heyman [‘Cardinality of a floor function set’, Integers 19 (2019), Article no. A67].
$S(x; q, q)$
confirms a recent numerical test of Heyman [‘Cardinality of a floor function set’, Integers 19 (2019), Article no. A67].
This work is in part supported by the National Natural Science Foundation of China (Grant Nos. 11771211, 11971370 and 12071375), by the NSF of Chongqing (Grant No. cstc2019jcy-msxm1651) and by the Young Talent-training Plan for college teachers in Henan province (2019GGJS241).