No CrossRef data available.
Published online by Cambridge University Press: 10 August 2023
We show that 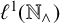 $\ell ^1(\mathbb {N}_\wedge )$ is
$\ell ^1(\mathbb {N}_\wedge )$ is 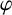 $\varphi $-amenable for each multiplicative linear functional
$\varphi $-amenable for each multiplicative linear functional 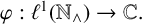 $\varphi :\ell ^1(\mathbb {N}_\wedge )\rightarrow \mathbb {C}.$ This is a counterexample to the final corollary of Jaberi and Mahmoodi [‘On
$\varphi :\ell ^1(\mathbb {N}_\wedge )\rightarrow \mathbb {C}.$ This is a counterexample to the final corollary of Jaberi and Mahmoodi [‘On 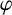 $\varphi $-amenability of dual Banach algebras’, Bull. Aust. Math. Soc. 105 (2022), 303–313] and shows that the final theorem in that paper is not valid.
$\varphi $-amenability of dual Banach algebras’, Bull. Aust. Math. Soc. 105 (2022), 303–313] and shows that the final theorem in that paper is not valid.