Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-25T19:36:12.210Z Has data issue: false hasContentIssue false
  • English
  • Français

A class of modules over a locally finite group III

Published online by Cambridge University Press:  17 April 2009

B. Hartley
B. Hartley
Affiliation:
Mathematics Institute, University of Warwick, Coventry, England; Department of Mathematics, University of Wisconsin, Madison, Wisconsin, USA.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let G be a locally finite group, k a field of characteristic p ≥ 0 and V a right kG-module. We say that V is an -module over kG, if each p′-subgroup H of G contains a finite subgroup F with the same fixed points as H in V. (By convention, 0′ is taken as the set of all primes.) Such modules arise as elementary abelian section of 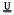-groups, a class of locally finite groups similar in many ways to the class of finite soluble groups.

The main theorem is that if V is an -module over kG with trivial Frattini submodule, and G is almost abelian, then every composition factor of V is complemented. This is a crucial ingredient in Tomkinson's theory of prefrattini subgroups in a certain subclass of 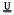. An example is given to show that the theorem breaks down for metabelian G. This leads to an example of a 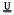-group in which there are no analogues of prefrattini subgroups - the first situation where one of the standard conjugacy classes of subgroups of finite soluble groups has no decent analogue in the whole class

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 14 , Issue 1 , February 1976 , pp. 95 - 110
Copyright
Copyright © Australian Mathematical Society 1976

References

[1]Farkas, Daniel R. and Snider, Robert L., “Group algebras whose simple modules are injective”, Trans. Amer. Math. Soc. 194 (1974), 241–248.CrossRefGoogle Scholar
[2]Gardiner, A.D., Hartley, B. and Tomkinson, M.J., “Saturated formations and Sylow structure in locally finite groups”, J. Algebra 17 (1971), 177–211.CrossRefGoogle Scholar
[3]Gillam, J.D., “Cover-avoid subgroups in finite solvable groups”, J. Algebra 29 (1974), 324–329.CrossRefGoogle Scholar
[4]Hartley, B., “Sylow subgroups of locally finite groups”, Proc. London Math. Soc. (3) 23 (1971), 159–192.CrossRefGoogle Scholar
[5]Hartley, B., “A class of modules over a locally finite group I”, J. Austral. Math. Soc. 16 (1973), 431–442.CrossRefGoogle Scholar
[6]Hartley, B., “A class of modules over a locally finite group II”, J. Austral. Math. Soc. Ser. A 19 (1975), 437–469.CrossRefGoogle Scholar
[7]Hartley, B., “Injective modules over group rings”, submitted.Google Scholar
[8]Kovács, L.G. and Newman, M.F., “Direct complementation in groups with operators”, Arch. Math. (Basel) 13 (1962), 427–433.CrossRefGoogle Scholar
[9]Passman, Donald S., Infinite group rings (Pure and Applied Mathematics, 6. Marcel Dekker, New York, 1971).Google Scholar
[10]Richardson, J.S., “Primitive idempotents and the socle in group algebras of periodic abelian groups”, submitted.Google Scholar
[11]Tomkinson, M.J., “Prefrattini subgroups and cover-avoidance properties in -groups”, Canad. J. Math. 27 (1975), 837–851.CrossRefGoogle Scholar
[12]Wehrfritz, B.A.F., Infinite linear groups. An account of the group-theoretic properties of infinite groups of matrices (Ergebnisse der Mathematik und ihrer Grenzgebiete, 76. Springer-Verlag, Berlin, Heidelberg, New York, 1973).Google Scholar