Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kumar, Vinod
and
Shukla, S. L.
1984.
Jakubowski starlike integral operators.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 37,
Issue. 1,
p.
117.
Cho, Nak Eun
and
Owa, Shigeyoshi
1992.
Certain differential operators for meromorphically $p$-valent convex functions.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 68,
Issue. 1,
Aouf, M. K.
1993.
New criteria for multivalent meromorphic starlike functions of order alpha.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 69,
Issue. 3,
Cho, N.E.
and
Owa, S.
1994.
On certain classes of meromorphically p-valent starlike functions.
Applied Mathematics Letters,
Vol. 7,
Issue. 3,
p.
25.
Cho, Nak Eun
Kwon, Oh Sang
and
Srivastava, H. M.
2005.
Inclusion relationships for certain subclasses of meromorphic functions associated with a family of multiplier transformations.
Integral Transforms and Special Functions,
Vol. 16,
Issue. 8,
p.
647.
Cho, Nak Eun
and
Noor, K. Inayat
2006.
Inclusion properties for certain classes of meromorphic functions associated with the Choi–Saigo–Srivastava operator.
Journal of Mathematical Analysis and Applications,
Vol. 320,
Issue. 2,
p.
779.
Cho, Nak Eun
and
Kim, In Hwa
2007.
Inclusion properties of certain classes of meromorphic functions associated with the generalized hypergeometric function.
Applied Mathematics and Computation,
Vol. 187,
Issue. 1,
p.
115.
Cho, NakEun
2009.
Inclusion Properties for Certain Classes of Meromorphic Functions Associated with a Family of Linear Operators.
Journal of Inequalities and Applications,
Vol. 2009,
Issue. 1,
p.
147069.
Aouf, M.K.
Shamandy, A.
Mostafa, A.O.
and
El-Emam, F.Z.
2011.
Inclusion properties of certain classes of meromorphic functions associated with the Wright generalized hypergeometric function.
Computers & Mathematics with Applications,
Vol. 61,
Issue. 5,
p.
1419.
Cho, N.E.
and
Yoon, M.
2011.
Subordination properties for meromorphic multivalent functions associated with the multiplier transformation.
Applied Mathematics and Computation,
Vol. 218,
Issue. 3,
p.
729.
Muhammad, Ali
2012.
On inclusion relationships of certain subclasses of meromorphic functions involving integral operator.
Annales UMCS, Mathematica,
Vol. 66,
Issue. 1,
Cho, Nak Eun
2012.
Sandwich-type theorems for meromorphic multivalent functions associated with the liu-srivastava operator.
Acta Mathematica Scientia,
Vol. 32,
Issue. 3,
p.
929.
Aouf, M.
Shamandy, A.
Mostafa, A.
and
El-Emam, F.
2012.
Some inclusion relationships and integral-preserving properties of certain subclasses of p-valent meromorphic functions associated with a family of linear operator.
Mathematica Slovaca,
Vol. 62,
Issue. 3,
p.
487.
El-Ashwah, R. M.
2012.
Inclusion Relationships for Certain Subclasses of Meromorphic Functions Defined by Using the Extended Multiplier Transformations.
ISRN Mathematical Analysis,
Vol. 2012,
Issue. ,
p.
1.
Andrei, Loriana
2014.
Some properties of certain subclasses of analytic functions involving adifferential operator.
Advances in Difference Equations,
Vol. 2014,
Issue. 1,
Cho, Nak Eun
2014.
Analytic Number Theory, Approximation Theory, and Special Functions.
p.
853.
Crişan, Oana
2016.
Subclasses of meromorphicallyp-valent functions involving a certain linear operator.
Mathematica Slovaca,
Vol. 66,
Issue. 3,
p.
605.
Mostafa, Adela O.
and
El-hawsh, Ghada M.
2019.
Differential subordination applications to a class of meromorphic multivalent functions associated with Mittag-Leffler function.
Journal of the Egyptian Mathematical Society,
Vol. 27,
Issue. 1,
Masih, Vali Soltani
Ebadian, Ali
and
Najafzadeh, Shahram
2020.
On Applications of Nunokawa and Sokół Theorem for p-Valency.
Bulletin of the Iranian Mathematical Society,
Vol. 46,
Issue. 2,
p.
471.
Ali, Ekram E.
El-Ashwah, Rabha M.
Albalahi, Abeer M.
and
Breaz, Nicoleta
2022.
Admissible Classes of Multivalent Meromorphic Functions Defined by a Linear Operator.
Mathematics,
Vol. 10,
Issue. 21,
p.
4136.
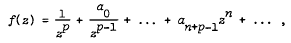
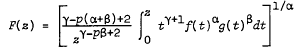
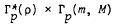 into
into  , where
, where  is the class of p-valent meromorphically starlike functions of order ρ, 0 ≤ ρ < 1. For the classes Γp(m, M) and Σp(m, M), we obtain class preserving integral operators of the form
is the class of p-valent meromorphically starlike functions of order ρ, 0 ≤ ρ < 1. For the classes Γp(m, M) and Σp(m, M), we obtain class preserving integral operators of the form