Published online by Cambridge University Press: 17 April 2009
The aim of this note is to obtain an intrinsic product formula for the centraliser of the injective tensor product of a couple of Banach spaces, Z(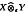 ). More precisely, we are going to prove that
). More precisely, we are going to prove that
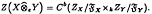
Here, the spaces 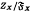 and
and 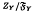 depend only on X and Y, respectively, and Xk denotes the topological k-product.
depend only on X and Y, respectively, and Xk denotes the topological k-product.
A Counterexaple used to demonstrate that the k-product cannot beavoided serves as an answer to a question posed by W. Rueß and D. Werner concerning the behaviour of M-ideals on 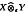 Y.
Y.