Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2025-01-03T20:46:23.559Z Has data issue: false hasContentIssue false
  • English
  • Français

Beurling's ordinary value

Published online by Cambridge University Press:  17 April 2009

Shinji Yamashita
Shinji Yamashita
Affiliation:
Department of Mathematics, Tokyo Metropolitan University, Fukazawa 2–1–1, Setagaya-ku, Tokyo, 158 Japan.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let n(w, f) be the number of w-points of f meromorphic in D = {|z| < 1}. Beurling defined the quantity 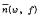and called w an ordinary value of f if 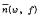< ∞. We shall consider the intermediate quantity ṉ(w, f) in the sense that n(w, f) ≤ ṉ(w, f) 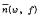, and construct two bounded holo-morphic functions f1 and f2 of finite Dirichlet integrals in D for which

and

.

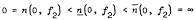Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 28 , Issue 1 , August 1983 , pp. 91 - 99
Copyright
Copyright © Australian Mathematical Society 1983

References

[1]Beurling, Arne, “Ensembles exceptionnels”, Acta Math. 72 (1940), 1–13.CrossRefGoogle Scholar
[2]Saks, Stanislaw, Theory of the integral, second revised edition (translated by Young, L.C.. Monografie Matematyczne, 7. Hafner, New York, 1937).Google Scholar