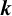Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-23T01:17:10.294Z Has data issue: false hasContentIssue false
  • English
  • Français

APPLICATIONS OF CIRCULANT MATRICES TO DETERMINANTS INVOLVING $\boldsymbol {k}$TH POWER RESIDUES

Part of: Algebraic number theory: global fields Exponential sums and character sums Polynomials and matrices

Published online by Cambridge University Press:  09 February 2022

HAI-LIANG WU Open the ORCID record for HAI-LIANG WU [Opens in a new window]  and
LI-YUAN WANG Open the ORCID record for LI-YUAN WANG [Opens in a new window]
HAI-LIANG WU
Affiliation:
School of Science, Nanjing University of Posts and Telecommunications, Nanjing 210023, PR China e-mail: [email protected]
LI-YUAN WANG*
Affiliation:
School of Physical and Mathematical Sciences, Nanjing Tech University, Nanjing 211816, PR China
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We use circulant matrices and hyperelliptic curves over finite fields to study some arithmetic properties of certain determinants involving Legendre symbols and kth power residues.

Keywords

determinantLegendre symbolcirculant matrix

MSC classification

Primary: 11C20: Matrices, determinants
Secondary: 11L05: Gauss and Kloosterman sums; generalizations 11R29: Class numbers, class groups, discriminants
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 106 , Issue 2 , October 2022 , pp. 243 - 253
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was supported by the National Natural Science Foundation of China (Grant No. 12101321) and the Natural Science Foundation of the Higher Education Institutions of Jiangsu Province (Grant No. 21KJB110002). The second author was supported by the Natural Science Foundation of the Higher Education Institutions of Jiangsu Province (Grant No. 21KJB110001).

References

Berndt, B. C., Evans, R. J. and Williams, K. S., Gauss and Jacobi Sums (Wiley, New York, 1998).Google Scholar
Carlitz, L., ‘Some cyclotomic matrices’, Acta Arith. 5 (1959), 293–308.CrossRefGoogle Scholar
Chapman, R., ‘Determinants of Legendre symbol matrices’, Acta Arith. 115 (2004), 231–244.CrossRefGoogle Scholar
Chapman, R., ‘My evil determinant problem’, Preprint, 2012, available from http://empslocal.ex.ac.uk/people/staff/rjchapma/etc/evildet.pdf.Google Scholar
Kra, I. and Simanca, S. R., ‘On circulant matrices’, Notices Amer. Math. Soc. 59 (2012), 368–377.CrossRefGoogle Scholar
Krachun, D., Petrov, F., Sun, Z.-W. and Vsemirnov, M., ‘On some determinants involving Jacobi symbols’, Finite Fields Appl. 64 (2020), 101672.CrossRefGoogle Scholar
Krattenthaler, C., ‘Advanced determinant calculus: a complement’, Linear Algebra Appl. 411 (2005), 68–166.CrossRefGoogle Scholar
Stembridge, J. R., ‘Nonintersecting paths, pfaffians and plane partitions’, Adv. Math. 83 (1990), 96–131.CrossRefGoogle Scholar
Sun, Z.-W., ‘On some determinants with Legendre symbol entries’, Finite Fields Appl. 56 (2019), 285–307.CrossRefGoogle Scholar
Vsemirnov, M., ‘On the evaluation of R. Chapman’s “evil determinant”’, Linear Algebra Appl. 436 (2012), 4101–4106.CrossRefGoogle Scholar
Vsemirnov, M., ‘On R. Chapman’s “evil determinant”: case $p\equiv 1\left(\mathrm{mod}\ 4\right)$ ’, Acta Arith. 159 (2013), 331–344.CrossRefGoogle Scholar
Wu, H.-L., ‘Determinants concerning Legendre symbols’, C. R. Math. Acad. Sci. Paris 359(6) (2021), 651–655.Google Scholar
Wu, H.-L., ‘Elliptic curves over ${F}_p$ and determinants of Legendre matrices’, Finite Fields Appl. 76 (2021), 101929.CrossRefGoogle Scholar
Wu, H.-L., She, Y.-F. and Ni, H.-X., ‘Trinomial coefficients and a determinant of Sun’, Preprint, 2021, arXiv:2108.10624.Google Scholar