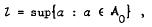Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Balder, E. J.
1984.
A General Approach to Lower Semicontinuity and Lower Closure in Optimal Control Theory.
SIAM Journal on Control and Optimization,
Vol. 22,
Issue. 4,
p.
570.
Balder, E. J.
1986.
On Seminormality of Integral Functionals and Their Integrands.
SIAM Journal on Control and Optimization,
Vol. 24,
Issue. 1,
p.
95.
Borisovich, Yu. G.
Gel'man, B. D.
Myshkis, A. D.
and
Obukhovskii, V. V.
1990.
New results in the theory of multivalued mappings. I. Topological characteristics and solvability of operator relations.
Journal of Soviet Mathematics,
Vol. 49,
Issue. 1,
p.
800.