No CrossRef data available.
Published online by Cambridge University Press: 25 October 2022
For a fixed integer h, the standard orthogonality relations for Ramanujan sums 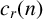 $c_r(n)$ give an asymptotic formula for the shifted convolution
$c_r(n)$ give an asymptotic formula for the shifted convolution 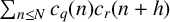 $\sum _{n\le N} c_q(n)c_r(n+h)$. We prove a generalised formula for affine convolutions
$\sum _{n\le N} c_q(n)c_r(n+h)$. We prove a generalised formula for affine convolutions  $\sum _{n\le N} c_q(n)c_r(kn+h)$. This allows us to study affine convolutions
$\sum _{n\le N} c_q(n)c_r(kn+h)$. This allows us to study affine convolutions 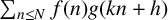 $\sum _{n\le N} f(n)g(kn+h)$ of arithmetical functions
$\sum _{n\le N} f(n)g(kn+h)$ of arithmetical functions  $f,g$ admitting a suitable Ramanujan–Fourier expansion. As an application, we give a heuristic justification of the Hardy–Littlewood conjectural asymptotic formula for counting Sophie Germain primes.
$f,g$ admitting a suitable Ramanujan–Fourier expansion. As an application, we give a heuristic justification of the Hardy–Littlewood conjectural asymptotic formula for counting Sophie Germain primes.