Published online by Cambridge University Press: 05 September 2022
We prove that a Banach algebra B that is a completion of the universal enveloping algebra of a finite-dimensional complex Lie algebra
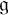 $\mathfrak {g}$
satisfies a polynomial identity if and only if the nilpotent radical
$\mathfrak {g}$
satisfies a polynomial identity if and only if the nilpotent radical
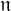 $\mathfrak {n}$
of
$\mathfrak {n}$
of
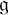 $\mathfrak {g}$
is associatively nilpotent in B. Furthermore, this holds if and only if a certain polynomial growth condition is satisfied on
$\mathfrak {g}$
is associatively nilpotent in B. Furthermore, this holds if and only if a certain polynomial growth condition is satisfied on
 $\mathfrak {n}$
.
$\mathfrak {n}$
.