No CrossRef data available.
Published online by Cambridge University Press: 06 October 2022
Let
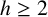 $h \geq 2$
be a positive integer. We introduce the concept of minimal restricted asymptotic bases and obtain some examples of minimal restricted asymptotic bases of order h.
$h \geq 2$
be a positive integer. We introduce the concept of minimal restricted asymptotic bases and obtain some examples of minimal restricted asymptotic bases of order h.
This work was supported by the National Natural Science Foundation of China (Grant No. 11971033), Top Talents Project of Anhui Department of Education (Grant No. gxbjZD05) and Key Project of Anhui Department of Education (Grant No. KJ2021A0099).