Article contents
A NOTE ON JEŚMANOWICZ’ CONJECTURE CONCERNING NONPRIMITIVE PYTHAGOREAN TRIPLES
Published online by Cambridge University Press: 21 October 2020
Abstract
Jeśmanowicz conjectured that
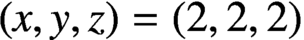 $(x,y,z)=(2,2,2)$
is the only positive integer solution of the equation
$(x,y,z)=(2,2,2)$
is the only positive integer solution of the equation
 $(*)\; ((\kern1.5pt f^2-g^2)n)^x+(2fgn)^y=((\kern1.5pt f^2+g^2)n)^x$
, where n is a positive integer and f, g are positive integers such that
$(*)\; ((\kern1.5pt f^2-g^2)n)^x+(2fgn)^y=((\kern1.5pt f^2+g^2)n)^x$
, where n is a positive integer and f, g are positive integers such that
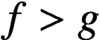 $f>g$
,
$f>g$
,
 $\gcd (\kern1.5pt f,g)=1$
and
$\gcd (\kern1.5pt f,g)=1$
and
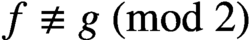 $f \not \equiv g\pmod 2$
. Using Baker’s method, we prove that: (i) if
$f \not \equiv g\pmod 2$
. Using Baker’s method, we prove that: (i) if
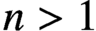 $n>1$
,
$n>1$
,
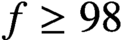 $f \ge 98$
and
$f \ge 98$
and
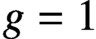 $g=1$
, then
$g=1$
, then
 $(*)$
has no positive integer solutions
$(*)$
has no positive integer solutions
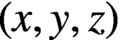 $(x,y,z)$
with
$(x,y,z)$
with
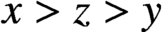 $x>z>y$
; and (ii) if
$x>z>y$
; and (ii) if
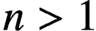 $n>1$
,
$n>1$
,
 $f=2^rs^2$
and
$f=2^rs^2$
and
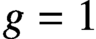 $g=1$
, where r, s are positive integers satisfying
$g=1$
, where r, s are positive integers satisfying
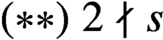 $(**)\; 2 \nmid s$
and
$(**)\; 2 \nmid s$
and
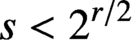 $s<2^{r/2}$
, then
$s<2^{r/2}$
, then
 $(*)$
has no positive integer solutions
$(*)$
has no positive integer solutions
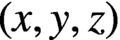 $(x,y,z)$
with
$(x,y,z)$
with
 $y>z>x$
. Thus, Jeśmanowicz’ conjecture is true if
$y>z>x$
. Thus, Jeśmanowicz’ conjecture is true if
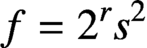 $f=2^rs^2$
and
$f=2^rs^2$
and
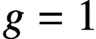 $g=1$
, where r, s are positive integers satisfying
$g=1$
, where r, s are positive integers satisfying
 $(**)$
.
$(**)$
.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.
References
 ${(({2}^{2m}-1)n)}^x+{({2}^{m+1}n)}^y={(({2}^{2m}+1)n)}^z$
’, Bull. Math. Soc. Sci. Math. Roumanie (N.S.) 57 (2014), 337–344.Google Scholar
${(({2}^{2m}-1)n)}^x+{({2}^{m+1}n)}^y={(({2}^{2m}+1)n)}^z$
’, Bull. Math. Soc. Sci. Math. Roumanie (N.S.) 57 (2014), 337–344.Google Scholar- 1
- Cited by



