Hostname: page-component-745bb68f8f-b95js Total loading time: 0 Render date: 2025-01-11T09:07:55.589Z Has data issue: false hasContentIssue false
  • English
  • Français

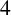A NEW HIGHER ORDER YANG–MILLS–HIGGS FLOW ON RIEMANNIAN $4$-MANIFOLDS

Part of: Variational problems in infinite-dimensional spaces Quantum field theory; related classical field theories Calculus on manifolds; nonlinear operators

Published online by Cambridge University Press:  29 November 2022

HEMANTH SARATCHANDRAN ,
JIAOGEN ZHANG  and
PAN ZHANG
HEMANTH SARATCHANDRAN
Affiliation:
School of Mathematical Sciences, University of Adelaide, Adelaide, South Australia 5005, Australia e-mail: [email protected]
JIAOGEN ZHANG
Affiliation:
School of Mathematical Sciences, University of Science and Technology of China, Hefei, Anhui 230026, PR China e-mail: [email protected]
PAN ZHANG*
Affiliation:
School of Mathematical Sciences, Anhui University, Hefei, Anhui 230601, PR China
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

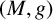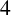Let $(M,g)$ be a closed Riemannian $4$ -manifold and let E be a vector bundle over M with structure group G, where G is a compact Lie group. We consider a new higher order Yang–Mills–Higgs functional, in which the Higgs field is a section of 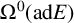$\Omega ^0(\text {ad}E)$ . We show that, under suitable conditions, solutions to the gradient flow do not hit any finite time singularities. In the case that E is a line bundle, we are able to use a different blow-up procedure and obtain an improvement of the long-time result of Zhang [‘Gradient flows of higher order Yang–Mills–Higgs functionals’, J. Aust. Math. Soc. 113 (2022), 257–287]. The proof relies on properties of the Green function, which is very different from the previous techniques.

Keywords

higher order Yang–Mills–Higgs flowline bundlelong-time existence

MSC classification

Primary: 58E15: Application to extremal problems in several variables; Yang-Mills functionals , etc.
Secondary: 58C99: None of the above, but in this section 81T13: Yang-Mills and other gauge theories
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 107 , Issue 2 , April 2023 , pp. 320 - 329
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The second and third authors are partially supported by the National Key R and D Program of China 2020YFA0713100 and the Natural Science Foundation of China (Grant Numbers 12141104 and 11721101). The first author is supported by the Australian Research Council via grant FL170100020. The second author is funded by the China Postdoctoral Science Foundation (Grant Number 2022M713057). The third author is supported by the Natural Science Foundation of China (Grant Number 12201001), the Natural Science Foundation of Anhui Province (Grant Number 2108085QA17) and the Natural Science Foundation of Universities of Anhui Province (Grant Number KJ2020A0009).

References

Alesker, S. and Shelukhin, E., ‘On a uniform estimate for the quaternionic Calabi problem’, Israel J. Math. 197 (2013), 309–327.CrossRefGoogle Scholar
Atiyah, M. and Bott, R., ‘The Yang–Mills equations over Riemann surfaces’, Philos. Trans. Roy. Soc. A 308 (1982), 523–615.Google Scholar
Donaldson, S. K. and Kronheimer, P., The Geometry of Four-Manifolds (Clarendon Press, Oxford, 1990).Google Scholar
Hassell, A., ‘The Yang–Mills–Higgs heat flow on ${\mathbb{R}}^3$ ’, J. Funct. Anal. 111 (1993), 431–448.10.1006/jfan.1993.1020CrossRefGoogle Scholar
Hong, M. C. and Tian, G., ‘Global existence of the $m$ -equivariant Yang–Mills flow in four dimensional spaces’, Comm. Anal. Geom. 12 (2004), 183–211.10.4310/CAG.2004.v12.n1.a10CrossRefGoogle Scholar
Kelleher, C., ‘Higher order Yang–Mills flow’, Calc. Var. Partial Differential Equations 100 (2019), Article no. 100.Google Scholar
Kuwert, E. and Schätzle, R., ‘Gradient flow for the Willmore functional’, Comm. Anal. Geom. 10 (2002), 307–339.10.4310/CAG.2002.v10.n2.a4CrossRefGoogle Scholar
Li, J. and Zhang, X., ‘The limit of the Yang–Mills–Higgs flow on Higgs bundles’, Int. Math. Res. Not. IMRN 2017 (2017), 232–276.CrossRefGoogle Scholar
Saratchandran, H., ‘Higher order Seiberg–Witten functionals and their associated gradient flows’, Manuscripta Math. 160 (2019), 411–481.CrossRefGoogle Scholar
Song, C. and Wang, C., ‘Heat flow of Yang–Mills–Higgs functionals in dimension two’, J. Funct. Anal. 272 (2017), 4709–4751.10.1016/j.jfa.2017.02.002CrossRefGoogle Scholar
Uhlenbeck, K. K., ‘Connections with ${L}^p$ -bounds on curvature’, Comm. Math. Phys. 83 (1982), 31–42.CrossRefGoogle Scholar
Waldron, A., ‘Long-time existence for Yang–Mills flow’, Invent. Math. 217 (2019), 1069–1147.CrossRefGoogle Scholar
Zhang, P., ‘Gradient flows of higher order Yang–Mills–Higgs functionals’, J. Aust. Math. Soc. 113 (2022), 257–287.CrossRefGoogle Scholar