Published online by Cambridge University Press: 08 October 2019
If  $\mathfrak{X}$ is a class of groups, we define a sequence
$\mathfrak{X}$ is a class of groups, we define a sequence  $\mathfrak{X}_{1},\mathfrak{X}_{2},\ldots ,\mathfrak{X}_{k},\ldots$ of group classes by putting
$\mathfrak{X}_{1},\mathfrak{X}_{2},\ldots ,\mathfrak{X}_{k},\ldots$ of group classes by putting 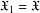 $\mathfrak{X}_{1}=\mathfrak{X}$ and choosing
$\mathfrak{X}_{1}=\mathfrak{X}$ and choosing  $\mathfrak{X}_{k+1}$ as the class of all groups whose nonnormal subgroups belong to
$\mathfrak{X}_{k+1}$ as the class of all groups whose nonnormal subgroups belong to 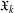 $\mathfrak{X}_{k}$. In particular, if
$\mathfrak{X}_{k}$. In particular, if 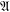 $\mathfrak{A}$ is the class of abelian groups,
$\mathfrak{A}$ is the class of abelian groups, 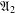 $\mathfrak{A}_{2}$ is the class of metahamiltonian groups, that is, groups whose nonnormal subgroups are abelian. The aim of this paper is to study the structure of
$\mathfrak{A}_{2}$ is the class of metahamiltonian groups, that is, groups whose nonnormal subgroups are abelian. The aim of this paper is to study the structure of 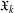 $\mathfrak{X}_{k}$-groups, with special emphasis on the case
$\mathfrak{X}_{k}$-groups, with special emphasis on the case 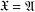 $\mathfrak{X}=\mathfrak{A}$. Among other results, it will be proved that a group has a finite commutator subgroup if and only if it is locally graded and belongs to
$\mathfrak{X}=\mathfrak{A}$. Among other results, it will be proved that a group has a finite commutator subgroup if and only if it is locally graded and belongs to 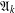 $\mathfrak{A}_{k}$ for some positive integer
$\mathfrak{A}_{k}$ for some positive integer 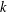 $k$.
$k$.
The authors are supported by GNSAGA (INdAM); the last two authors are members of AGTA—Advances in Group Theory and Applications (www.advgrouptheory.com).