This dissertation is highly motivated by d.r.e. Nondensity Theorem, which is interesting in two perspectives. One is that it contrasts Sacks Density Theorem, and hence shows that the structures of r.e. degrees and d.r.e. degrees are different. The other is to investigate what other properties a maximal degree can have.

In Chapter 1, we briefly review the backgrounds of Recursion Theory which motivate the topics of this dissertation.

In Chapter 2, we introduce the notion of 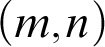$(m,n)$ -cupping degree. It is closely related to the notion of maximal d.r.e. degree. In fact, a 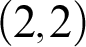$(2,2)$ -cupping degree is maximal d.r.e. degree. We then prove that there exists an isolated 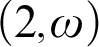$(2,\omega )$ -cupping degree by combining strategies for maximality and isolation with some efforts.

Chapter 3 is part of a joint project with Steffen Lempp, Yiqun Liu, Keng Meng Ng, Cheng Peng, and Guohua Wu. In this chapter, we prove that any finite boolean algebra can be embedded into d.r.e. degrees as a final segment. We examine the proof of d.r.e. Nondensity Theorem and make developments to the technique to make it work for our theorem. The goal of the project is to see what lattice can be embedded into d.r.e. degrees as a final segment, as we observe that the technique has potential be developed further to produce other interesting results.

Abstract prepared by Yong Liu.

E-mail: [email protected]