In silico exploration of the mechanisms that underlie parasite-induced anorexia in sheep

Yan C. S. M. Laurenson; Stephen C. Bishop; Ilias Kyriazakis

doi:10.1017/S0007114511001371

In silico exploration of the mechanisms that underlie parasite-induced anorexia in sheep

Published online by Cambridge University Press: 20 April 2011

Yan C. S. M. Laurenson ,

Stephen C. Bishop and

Ilias Kyriazakis

Show author details

Yan C. S. M. Laurenson*: Affiliation:
The Roslin Institute and Royal (Dick) School of Veterinary Studies, University of Edinburgh, MidlothianEH25 9RG, UK
Stephen C. Bishop: Affiliation:
The Roslin Institute and Royal (Dick) School of Veterinary Studies, University of Edinburgh, MidlothianEH25 9RG, UK
Ilias Kyriazakis: Affiliation:
School of Agriculture, Food and Rural Development, Newcastle University, Newcastle upon TyneNE1 7RU, UK Veterinary Faculty, University of Thessaly, PO Box 199, 43100Karditsa, Greece
*: *Corresponding author: Mr Yan Laurenson, email [email protected]

Article contents

Abstract
Materials and methods
Results
Discussion
Conclusion
References

Rights & Permissions

Abstract

A model was used to investigate two mechanisms describing reductions in food intake (anorexia) observed during gastrointestinal parasitism in lambs, and to explore relationships between anorexia and food composition. The mechanisms were either a reduction in intrinsic growth rate, leading to a consequent reduction in food intake (mechanism 1; M1), or a direct reduction in food intake (mechanism 2; M2). For both mechanisms, lambs growing from 2 to 6 months of age were modelled, with one of three levels of trickle challenge with Teladorsagia circumcincta. Scenarios were simulated for feeds varying in either protein or energy content, or both. Major differences were found between the predictions resulting from M1 and M2 on low-energy foods that constrained the intake of uninfected lambs through bulk. With M1, food intake was governed by the first operating constraint, whereas with M2 an additivity of constraints was observed. On the other foods, the duration of anorexia increased with increasing energy content of feed for M1, whilst the duration of anorexia decreased with increasing protein content of feed for M2.For foods that did not have an impact upon lambs' gastrointestinal tract capacity, published data were consistent with predictions of M2. Due to an absence of experimental data, no conclusions could be drawn for relationships between anorexia and food composition in the presence of other limiting constraints, such as bulk for low-energy foods. In conclusion, available experimental data and model predictions were consistent with anorexia having an impact directly on food intake, and with impacts of anorexia increasing with decreasing protein content.

Keywords

Lambs Food composition Modelling Nematodes

Type: Full Papers
Information: British Journal of Nutrition , Volume 106 , Issue 7 , 14 October 2011 , pp. 1023 - 1039

DOI: https://doi.org/10.1017/S0007114511001371 [Opens in a new window]
Copyright: Copyright © The Authors 2011

Gastrointestinal parasitism is one of the most pervasive challenges to the health and welfare of mammalian hosts. One of the main consequences of gastrointestinal parasitism is the occurrence of a reduction in voluntary food intake, henceforth called anorexia, which under subclinical infections accounts for up to a 20 % reduction in the food intake of parasitised animals, compared with their non-infected counterparts⁽Reference Coop, Sykes and Angus¹^–Reference Greer, Sedcole and Jay³⁾. Clinical helminth infections, however, may lead to a complete cessation of eating⁽Reference Reid, Armour and Urquhart⁴^–Reference Saad, Hussein and Dargie⁶⁾. Given the fact that gastrointestinal parasitism imposes nutritional penalties on the host⁽Reference Sykes, Kyriazakis, Meng, Ren and Cao⁷⁾, anorexia and its subsequent consequences are the major contributors to the impacts of parasitism on host performance and overall fitness. The effects on host performance can be large; for example, the economic cost of gastrointestinal parasitism of grazing sheep in the UK was estimated at about £84 million per year⁽Reference Nieuwhof and Bishop⁸⁾. In the present paper we deal with the issue of anorexia during gastrointestinal parasitism in sheep because of its apparent economic significance and the consequent attention the phenomenon has received in these hosts. We are of the view, however, that the principles we develop will be relevant to other parasite–mammalian host systems.

There are both functional and causal hypotheses to account for the occurrence of anorexia during parasitism in most mammalian hosts, including sheep. For example, Kyriazakis et al. ⁽Reference Kyriazakis, Tolkamp and Hutchings⁹⁾ have suggested that anorexia develops in order to allow hosts to cope with the exposure to pathogens. Langhans⁽Reference Langhans¹⁰⁾ and Plata-Salamán⁽Reference Plata-Salamán¹¹⁾, on the other hand, have suggested that anorexia is a consequence of the activation of the immune response by anorexinogenic cytokines, which are produced in response to infection. However, neither of these two groups of hypotheses is able to adequately account for or predict the extent of anorexia, nor do they provide a framework for predicting consequences on the food intake and performance of parasitised animals. Thus, this phenomenon continues to be paradoxical⁽Reference Kyriazakis, Tolkamp and Hutchings⁹⁾.

There are at least two ways to account for the reduction in the food intake of growing animals during exposure to environmental and other stressors. Wellock et al. ⁽Reference Wellock, Emmans and Kyriazakis¹²⁾ have suggested that the reduction in food intake is a consequence of a reduction in the intrinsic growth rate of the animals; the implication of this is that the reduction in food intake may be modelled as a consequence of reduction in growth (mechanism 1). This mechanism was implemented by Vagenas et al. ⁽Reference Vagenas, Bishop and Kyriazakis¹³^, Reference Vagenas, Bishop and Kyriazakis¹⁴⁾ when modelling host–parasite interactions in growing lambs, and implies that animals' intrinsic growth capability changes, for example, in a manner akin to the gene expression alterations seen with fetal programming⁽Reference Burdge, Hanson and Slater-Jefferies¹⁵⁾. Sandberg et al. ⁽Reference Sandberg, Emmans and Kyriazakis²⁾, on the other hand, have suggested that anorexia could be modelled as a direct reduction in the relative food intake of the parasitised animals (mechanism 2), which may be due to inappetence caused by immune response components such as cytokines. These authors have used this approach to model the time trend of food intake during the course of infection, mainly in pigs. A third approach has been proposed by Black et al. ⁽Reference Black, Bray, Giles and Kyriazakis¹⁶⁾ in which the above two mechanisms were used in equal proportion to account for the reduction in food intake. This approach is not considered any further in the present paper, due to it simply being a composite of the other two proposed mechanisms. To date, no attempt has been made to compare and consider the consequences of the above mechanisms on the extent of anorexia and its consequences on the performance of parasitised animals.

Further gaps exist in our understanding of the relationship between food composition and anorexia. For example, currently there is little known on whether and how food composition affects the characteristics of anorexia, such as its extent, duration and impact on animal performance. A recent paper by Kyriazakis⁽Reference Kyriazakis¹⁷⁾ reviewed extensively the literature on this issue and concluded that there was a significant lack of experimental evidence that would allow us to reach unequivocal conclusions about this relationship, and use it to the advantage of parasitised hosts. One issue that was specifically identified by the review was the lack of evidence on the nature of parasite-induced anorexia on low-quality foods, irrespective of whether such foods were low in N or energy.

The aim of the present paper was to investigate, in silico, the consequences of the above two mechanisms on the food intake of parasitised sheep. Parasitism was through infection with Teladorsagia circumcincta, which is the most prevalent parasite of sheep in temperate climates⁽Reference Stear, Doligalska and Donskow-Schmelter¹⁸⁾. We used the model of Vagenas et al. ⁽Reference Vagenas, Bishop and Kyriazakis¹³^, Reference Vagenas, Bishop and Kyriazakis¹⁴⁾ as our starting point, as it is capable of accounting for the interaction between nutrition and gastrointestinal parasitism for growing and immunologically naive sheep. Several modifications were made to the model, which are described below, to alter both the cause and the mechanism of impact of anorexia. To model the consequences of T. circumcinta infection, anorexia was parameterised as a function of immune responses, rather than the worm mass parameterisation used by Vagenas et al. ⁽Reference Vagenas, Bishop and Kyriazakis¹³⁾. Further, the model was altered to allow for the investigation of the two proposed mechanisms by which anorexia leads to reduced food intake. The outcomes of this exercise were compared with existing data on the food intake of parasitised sheep in order to provide insights into the nature of anorexia during gastrointestinal parasitism in sheep and its likely impacts on host performance. In addition, we investigated the relationship between anorexia and food composition. Our hypothesis was that we would observe interactions between food composition and mechanism of anorexia, in terms of the observed anorexia, total food intake and level of parasitism. The outcomes of this exercise were expected to have heuristic value, giving insight into the design of future experiments that wish to address this issue.

Materials and methods

Host–parasite interaction model

A previously developed model⁽Reference Vagenas, Bishop and Kyriazakis¹³⁾ that describes the impact of host nutrition, genotype and gastrointestinal parasitism in a growing lamb was modified and used to explore two mechanisms to account for parasite-induced anorexia. A schematic diagram describing the structure of the model is provided in Fig. 1. A description of each component of the model is given below. Equations and relationships previously published are given in the corresponding sections of Appendix 1, whereas modifications to the model remain in the main body of the text. First, the model for daily growth of an unchallenged animal is described for both non-limiting and nutritionally limiting conditions; the model is then extended to accommodate parasitic challenges, host immunity and host–parasite interactions, and to predict the growth of the lamb and its parasitic burden over time.

Fig. 1 Schematic description of host–parasite interactions and parasite-induced anorexia mechanisms (mechanism 1: intrinsic growth reduction mechanism (); mechanism 2: food intake reduction mechanism ()) in sheep infected with gastrointestinal nematodes. Rectangular boxes indicate the flow of food resources (for descriptions of the components, see the Methods section). Rounded boxes indicate host–parasite interactions and diamond boxes indicate key quantifiable parasite lifecycle stages.

The parasite-free animal

Intrinsic growth model

The growing lamb is described by the initial fleece-free empty body weight (body weight minus gut fill and wool) and the expected protein and lipid body content at maturity (P _m and L _m, respectively). The intrinsic growth rate of the lamb (B; kg/d) is given as equation 1 in Appendix 1.

The fleece-free empty body is considered to be the sum of the body protein, ash, water and lipid, and it is assumed that the lamb aims to achieve its expected intrinsic growth for these components. The desired maximum daily protein growth (ΔPG _max; kg/d), desired daily lipid growth (ΔL _des; kg/d), daily accretion of ash (ΔAsh; kg/d) and daily accretion of water (ΔWater; kg/d) are given as equations 2, 3, 4 and 5, respectively, in Appendix 1.

The live weight of the lamb is given by the fleece-free empty body weight plus wool and gut fill. The expected maximum daily wool growth (ΔPWool _max; kg/d) and gut fill (GF; kg) are given as equations 6 and 7 in Appendix 1.

Resource requirements and food intake

The protein and energy requirements to fulfil the expected growth rates are subsequently estimated. Only the protein and energy requirements have been considered⁽Reference Wellock, Emmans and Kyriazakis¹⁹⁾, as all other nutrient requirements are assumed to be satisfied by the diet. The daily protein requirements for maintenance, growth and wool are estimated by equations 8, 9 and 10 in Appendix 1. Energy requirements are estimated assuming that the deposition of wool protein has the same energy requirement per unit mass as body protein⁽²⁰⁾. The daily energy requirements for maintenance, growth and wool are estimated by equations 11, 12 and 13 in Appendix 1. Total protein requirements (PR) and total energy requirements (ER) are simply the sum of the individual requirements for maintenance, growth and wool.

It is assumed that the lamb will attempt to ingest sufficient nutrients to meet its expected requirements for growth. The desired feed intake is therefore the feed intake necessary to meet the expected requirements. Desired food intake for meeting, separately, the energy (FI _E) and protein (FI _P) requirements of the lamb are estimated by equations 14 and 15 in Appendix 1. The desired food intake of the animal is calculated as the higher of FI _E and FI _P. The energy requirements of the lamb were expressed in terms of effective energy (EE) (MJ/kg) in accordance with Emmans⁽Reference Emmans²¹⁾. These EE requirements were linked to the metabolisable energy (ME) (MJ/kg) yielded by a feed using equation 16 in Appendix 1.

Constrained resources

Under many circumstances resources may be constrained or insufficient to meet requirements. The procedure described above results in a food intake that increases as the quality (protein and energy content) of the feed decreases. However, it has been observed that the rate of increase in daily food intake declines as feed quality declines, and daily food intake may decrease for feeds with a low energy content⁽Reference Kyriazakis and Emmans²²⁾ due to an assumed maximum capacity for bulk. To represent this, a quantity called constrained food intake (CFI) is defined by equation 17 in Appendix 1. This relationship implies that the capacity of the animal for daily indigestible organic matter (CAP; kg) and the energy content of the food jointly determine the constrained food intake. CAP in young lambs has been found to increase linearly, equal to proportionally 0·0223 of the current body weight up to 0·51 of mature body weight, and to remain constant thereafter⁽Reference Lewis, Macfarlane and Simm²³⁾. Thus, CAP is given by equation 18 in Appendix 1.

Actual food intake is then the lower of desired food intake and CFI. Efficiency of digestion, accounting for level of feeding (LF), rumen outflow rate and current state of the lamb, and hence metabolisable protein (MP) available to the animal, were calculated using the equations described by the Agricultural and Food Research Council (AFRC)⁽²⁰⁾.

Allocation of nutrients

Ingested protein and energy are allocated to various bodily functions. The maintenance needs of the lamb are assumed to be satisfied first and remaining nutrients are allocated to production (body protein, body lipid and wool growth). The energy remaining after allocation to maintenance and production is subsequently stored as additional lipid. The daily lipid deposited (ΔLipid) is given by equation 19 in Appendix 1. If ΔLipid is negative, then lipid will be catabolised to satisfy the animal's energetic needs for other functions as given by equation 20 in Appendix 1.

If the lamb has a MP intake that is below its maintenance requirements, it is assumed to use its body reserves to cover its maintenance functions. If this protein inadequacy is prolonged the lamb will catabolise body protein, eventually leading to death. The quantity of protein that the animal can mobilise from its body, i.e. labile protein (P _Labile)⁽Reference Houdijk, Jessop and Kyriazakis²⁴^, Reference Sykes²⁵⁾, is defined by equation 21 in Appendix 1.

The baseline body lipid level (L _base), i.e. the minimum body lipid content for animal survival, is estimated as a proportion of its body protein content (P) as given by equation 22 in Appendix 1. If the energy intake of the lamb is not sufficient to meet this baseline body lipid level, then energy allocated towards protein growth is retracted and reallocated to lipid accretion. This scenario, which was absent from our previous model, is modelled by first calculating the required protein reduction (PR _Red) that would be sufficient to fulfil the L _base energy requirement. This is estimated as:

$\begin{eqnarray} PR _{Red} = \frac {\left (\left (\frac { bl }{ bp }\right )\cdot (( L _{base}\cdot P ) - L )\right )}{\left (\left ( L _{base}\cdot \left (\frac { bl }{ bp }\right )\right ) + 1\right )}\,(kg), \end{eqnarray}$

where P = current body protein (kg), L = current body lipid (kg), bl = energetic cost per kg of lipid deposition (56 MJ/kg)⁽Reference Emmans²¹⁾ and bp = energetic cost per kg of protein deposition (50 MJ/kg)⁽Reference Emmans²¹⁾.

The energy lipid shortfall (E _LS) is therefore calculated as:

$\begin{eqnarray} E _{LS} = PR _{Red}\cdot bp \,(MJ). \end{eqnarray}$

Subsequently, daily protein (ΔP) and daily lipid (ΔL) deposited are given as:

$\begin{eqnarray} \Delta P = \Delta PG - PR _{Red}\,(kg), \end{eqnarray}$

$\begin{eqnarray} \Delta L = \Delta Lipid + \left (\frac { E _{LS}}{ bl }\right )\,(kg), \end{eqnarray}$

where ΔPG = protein growth (kg).

The parasitised animal

Protein loss

Parasitism leads to protein loss in animals through damage to the gastrointestinal tract by ingested larvae and adult worms that develop from such larvae. Ingested larvae have a cost to the host manifested by protein loss, for example, tissue loss or plasma loss⁽Reference Houdijk, Jessop and Kyriazakis²⁴⁾. The potential protein loss (PLI _Pot) due to larval intake (LI) when there is no immune response is given by equation 23 in Appendix 1.

The animal is able to reduce the damage caused by LI through its immune response. Thus in the presence of an immune response the protein loss due to LI (PLI) is assumed to decrease. Actual protein loss due to LI is therefore given by equation 24 in Appendix 1.

A proportion of the ingested larvae (LI) will establish in the host gastrointestinal tract and develop to adult worms (see below). The adult worms will also cause protein loss to the host, for example, via damaged tissue or reduced absorption. However, the total number of adult worms present in the gastrointestinal tract (worm burden; WB) does not provide a complete description of the parasitic burden of the lamb⁽Reference Bishop and Stear²⁶⁾, because this does not take into account the mass of the adult worm burden or the effects of population density. To fully account for this, it is necessary to account for the total mass of the worm burden. Worm length has been shown to be strongly positively correlated with the fecundity (F) of the worm (number of eggs produced)⁽Reference Stear and Bishop²⁷⁾. Further, it is assumed that worm length is closely related to, and hence may be used as a proxy for, worm mass. Therefore, the worm mass (WM) of a population of worms can be approximated as:

$\begin{eqnarray} WM \approx F \cdot WB . \end{eqnarray}$

To take into account the density-dependence effects upon the worm population, in which individual worm size and fecundity decrease with increasing worm burden, fecundity was scaled as an inverse function of worm burden, assuming a mean worm burden of 2500. Thus, fecundity was scaled as given by equation 26 in Appendix 1, and worm mass given by equation 27 in Appendix 1.

The protein loss caused by worm mass (PWM) is given by equation 28 in Appendix 1. Total protein loss (PLoss) due to parasitism is therefore estimated as the sum of protein loss due to LI (PLI) and protein loss caused by worm mass (PWM).

Immune response

The lamb is assumed to invest in the immune response in order to reduce the impact of parasitism. Lambs are initially naive to parasites and they develop immunity as a function of their exposure to infective larvae. The immune response is represented by the host-controlled traits of nematode establishment (ɛ), fecundity (F) and mortality (μ). The functions used to describe these three immune response traits, modified from those described by Louie et al. ⁽Reference Louie, Vlassoff and Mackay²⁸⁾ and Vagenas et al. ⁽Reference Vagenas, Bishop and Kyriazakis¹³⁾, are given by the following sigmoidal relationships:

$\begin{eqnarray} \varepsilon = \left (\frac { \varepsilon _{max}\cdot K _{ \varepsilon }^{^{3}}}{ K _{ \varepsilon }^{^{3}} + \left ({ \sum _{ t } } LI ^{^\ast }\right )^{3}}\right ) + \varepsilon _{min}\quad (proportion\,of\,larvae\,establishing), \end{eqnarray}$

$\begin{eqnarray} \mu = \left (\frac { \mu _{max}\cdot \left ({ \sum _{ t } } LI ^{^\ast }\right )^{3}}{ mi ^{3} + \left ({ \sum _{ t } } LI ^{^\ast }\right )^{3}}\right ) + \mu _{min}\quad (proportion\,of\,adult\,worms/d), \end{eqnarray}$

$\begin{eqnarray} F = \left (\frac { F _{max}\cdot fi ^{3}}{ fi ^{3} + \left ({ \sum _{ t } } LI ^{^\ast }\right )^{3}}\right ) + F _{\,min\,}\quad (eggs/worm/d), \end{eqnarray}$

where ɛ_max, μ_max and F _max = maximum establishment (0·7⁽Reference Jackson, Greer and Huntley²⁹⁾), mortality (0·11⁽Reference Jackson, Greer and Huntley²⁹⁾) and fecundity (20⁽Reference Bishop and Stear²⁶⁾) rates, respectively, ɛ_min, μ_min and F _min = minimum establishment (0·06⁽Reference Jackson, Greer and Huntley²⁹⁾), mortality (0·01⁽Reference Kao, Leathwick and Roberts³⁰⁾) and fecundity (5⁽Reference Vagenas, Bishop and Kyriazakis¹³^, Reference Vagenas, Bishop and Kyriazakis¹⁴⁾) rates, respectively, ${ \sum _{ t } } LI ^{^\ast }$ = scaled cumulative LI (see below), and K _ɛ, mi and fi = rate constants for establishment (200 000), mortality (450 000) and fecundity (230 000), respectively.

Scaled cumulative LI ( ${ \sum _{ t } } LI ^{^\ast }$ ) is given as:

$\begin{eqnarray} { \sum _{ t } } LI ^{^\ast } = { \sum _{ t - 1} } LI ^{^\ast } + \left (\left ( LI _{max}\cdot \frac { LI }{ LI + cli }\right )\cdot \left (\frac { PAC _{Imm}}{( PRQ _{Imm})_{Tot}}\right )\right ), \end{eqnarray}$

where cli = constant of relationship between ${ \sum _{ t } } LI ^{^\ast }$ and ${ \sum _{ t - 1} } LI ^{^\ast }$ (2000⁽Reference Vagenas, Bishop and Kyriazakis¹³⁾).

The immune response is assumed to be predominantly driven by protein; as such, the protein requirements for immunity are calculated separately for LI and worm mass (WM) and are given as equations 29 and 30 in Appendix 1. Thus the total protein required for immunity ((PRQ _Imm)_Tot) is given by the sum of requirements for LI and worm mass.

Effect of parasitism on protein partitioning

As in the case of no parasitic challenge, it is assumed that the maintenance needs of the lamb will be satisfied first. If the available protein is less than the requirements for maintenance then the lamb must catabolise protein. In this case, no protein is allocated to immunity or production. Otherwise, nutrients remaining after allocation to maintenance are allocated to immunity and production (body and wool growth) in proportion to their requirements. Protein allocated to production (PAC_Growth) and protein allocated to immunity (PAC_Imm) are given by equations 31 and 32 in Appendix 1. This approach is different from the traditional view of considering the requirements for the immune response as part of the maintenance requirements⁽Reference Coop and Kyriazakis³¹⁾. Metabolised protein allocated to immunity is assumed to be used with an efficiency of 0·59⁽²⁰⁾; thus the quantity of protein associated with the immune function per d is given by equation 33 in Appendix 1.

Due to protein being allocated to immunity there will be a reduction in protein loss due to parasitism. Protein loss due to worm mass is then re-estimated after the reduction in fecundity and recalculating worm mass, and the protein loss spared added back to the available protein (P _Avail). Subsequently the final protein allocated to production ( $PA C _{Prod}^{F}$ ) is estimated as:

$\begin{eqnarray} PA C _{Prod}^{F} = P _{Avail} - ( PAC _{Imm} + PLoss )\,(kg/d). \end{eqnarray}$

Effect of parasitism on food intake

In the model of Vagenas et al. ⁽Reference Vagenas, Bishop and Kyriazakis¹³⁾, anorexia was assumed to be a function of adult worm burden; however, this mechanism leads to anorexia commencing too late (i.e. after 21 d post-infection) for T. circumcincta; Coop et al. ⁽Reference Coop, Sykes and Angus¹⁾ and Greer et al. ⁽Reference Greer, Huntley and Mackellar³²⁾ reported that anorexia became apparent 7–10 d after initial challenge with T. circumcincta. Because immune response components such as cytokines cause inappetance, we modelled anorexia as a direct function of the rate of acquisition of immunity as this formulation captures the time-dependent dynamics of T. circumcincta-induced anorexia. Anorexia was then applied to either the intrinsic growth rate or actual food intake, as described below, through a reduction parameter (RED). RED is calculated as a direct function of the rates (i.e. 1st derivatives) of immune response acquisition as:

$\begin{eqnarray} RED = R _{c}\cdot \left (\frac { d\varepsilon }{ dx } + \frac { d\mu }{ dx } + \frac { dF }{ dx }\right ), \end{eqnarray}$

where R _c = constant linking the reduction to the immune response, ɛ = establishment, F = fecundity and μ = mortality. During the course of an infection RED will start at zero, rise to a maximum and then decline towards zero as immunity is fully acquired.

Anorexia was then implemented through the two mechanisms as follows.

Mechanism 1: The reduction was applied to the intrinsic growth rate; thus the reduction in food intake may be modelled as a consequence of a reduction in growth. In order to represent the reduction in intrinsic growth the reduction calculated above is implemented as⁽Reference Vagenas, Bishop and Kyriazakis¹³⁾:

$\begin{eqnarray} B _{New} = B \cdot RED , \end{eqnarray}$

where B _New = new rate of tissue mass retention.

Mechanism 2: The reduction equation was applied directly to the food intake (FI) of the lamb to obtain:

$\begin{eqnarray} FI _{New} = FI \cdot RED . \end{eqnarray}$

FI _New therefore gives the food intake of the lamb as a consequence of parasite-induced anorexia.

Experimental design

The model was used to explore the consequences of the two different mechanisms for parasite-induced anorexia. For both mechanisms, the model was used to investigate the effect of nutrition and varying levels of challenge with T. circumcincta on the performance of a lamb growing from 2 to 6 months of age. This time period was chosen to represent the period in which the lambs are growing at their maximum rate whilst not being fully immune, and thus the period in which parasitism can be expected to have its greatest impact upon weight gain. The model predicts events for time increments of 1 d and it was updated on a daily basis, with predictions from the previous day being used as the starting point for the current day.

The lambs were simulated to have an initial live weight of about 20 kg corresponding to an initial empty body weight of 12·73 kg and an initial body protein weight of 2·03 kg. The genotype traits of the lamb were a protein weight at maturity (P _m) of 9·525 kg, a lipid weight at maturity (L _m) of 40·11 kg and a growth rate parameter (B) of 0·0125. These parameter values were chosen to give growth characteristics similar to those of Scottish Blackface lambs, a common British breed.

For both mechanisms of parasite-induced anorexia, lambs were given a trickle challenge infection of T. circumcincta L₃ larvae of either control, 1000 or 5000 L₃ per d, from day 1. Numerous levels of larval challenge were initially investigated; the 5000 L₃ per d challenge is reported here as it corresponds to the high level of subclinical T. circumcincta infections investigated by Coop et al. ⁽Reference Coop, Graham and Jackson³³⁾, which lead to parasite-induced anorexia and reduced growth rate. The 1000 L₃ per d challenge was chosen to represent a lower challenge level as used by Valderrábano et al. ⁽Reference Valderrábano, Delfa and Uriarte³⁴⁾ and Coop et al. ⁽Reference Coop, Sykes and Angus¹⁾, and for comparison with the previously published model of Vagenas et al. ⁽Reference Vagenas, Bishop and Kyriazakis¹³^, Reference Vagenas, Bishop and Kyriazakis¹⁴⁾. This level of infection also has consequences on the food intake predicted by the two different mechanisms and therefore has a heuristic value.

This scenario was simulated for each of seven different grass qualities (Table 1; Fig. 2). Foods 1, 4 and 7 were taken from appendix 1 of the AFRC⁽²⁰⁾ manual as being representative of poor-, medium- and good-quality grasses; they were equally spaced in terms of energy and N contents. Foods 1 and 7 have been previously used in the same model⁽Reference Vagenas, Bishop and Kyriazakis¹³^, Reference Vagenas, Bishop and Kyriazakis¹⁴^, Reference Doeschl-Wilson, Vagenas and Kyriazakis³⁵⁾ to investigate the impact of food composition on the extent of parasitism in growing lambs. Four additional ‘grasses’ (foods 2, 3, 5 and 6) were also used for the purposes of the simulations; their composition was considered appropriate and within realistic bounds to investigate the impact of food energy or protein content alone on anorexia during parasitism. Foods 2 and 6 were isoenergetic in terms of ME to grass 4 (10 MJ ME/kg DM), but contained N levels that were placed between foods 1, 4 and 7, whereas foods 3 and 5 were isonitrogenous in terms of crude protein (CP) to grass 4 (140 g CP/kg DM), but contained ME levels that were placed between the same foods. The composition of foods 2, 3, 5 and 7 was also estimated in accordance with AFRC recommendations⁽²⁰⁾. All foods were assumed to have the same ash content (70 g/kg DM) and the same fat content (30 g/kg DM⁽Reference Noci, Monahan and French³⁶⁾). For the calculation of the MP content of the foods, the assumptions made regarding the impact of the feeding level in relation to maintenance were adjusted as the animals grew, but the yields of protein given in Table 1 are for a level of feeding of six times maintenance.

Table 1 Composition of the foods used in the experiments*

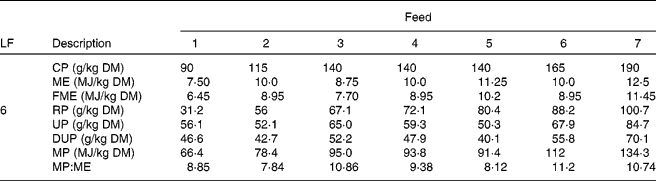
LF, level of feeding as multiples of energy requirements for maintenance; CP, crude protein; ME, metabolisable energy; FME, fermentable metabolisable energy; RP, rumen degradable protein; UP, undegradable protein; DUP, digestible undegradable protein; MP, metabolisable protein; AFRC, Agricultural and Food Research Council.

* The composition of foods 1, 4 and 7 were taken from appendix 1 of the recommendations of the AFRC⁽²⁰⁾ as examples of poor-, medium- and good-quality grass, respectively. For foods 2, 4 and 6 the CP content of the food was varied systematically whilst keeping ME content constant, and for foods 3, 4 and 5 the ME content was varied systematically whilst the CP content remained constant.

Fig. 2 Nutritional space occupied by the foods used in the simulated experiments. Foods 2, 4 and 6 differed in their crude protein (CP) contents, foods 3, 4 and 5 differed in their metabolisable energy (ME) contents and foods 1, 4 and 7 differed in both their CP and ME contents.

The outputs from the model are presented for food intake (kg/d) and daily worm egg count (eggs/d). The food intake was reported in order to present the differences that occur for the two mechanisms of parasite-induced anorexia. The live-weight predictions are not presented here, as they are a direct consequence of the food intake of the lamb. The daily worm egg count, which is the total number of eggs produced per d, was chosen to present the parasitological outcomes of our predictions. This was preferred over faecal egg count (FEC), which is the number of eggs per g faeces, in order to overcome the dilution effect of the quantity of faeces produced upon the parasitological predictions.

Model validation

The model was parameterised using the results of Coop et al. ⁽Reference Coop, Sykes and Angus¹⁾. However, to ensure that the values predicted by the model were representative of values reported in experiments other than Coop et al. ⁽Reference Coop, Sykes and Angus¹⁾, a search was carried out for published comparable experiments. Experiments investigating the impacts of T. circumcinta infection on growing lambs were checked against selected criteria. These criteria were: sufficient information on food composition, ad libitum feeding and the use of non-parasitised control animals. Two experiments met these criteria and contained sufficient information to enable simulations to be carried out for comparison; these were experiments described by Greer et al. ⁽Reference Greer, Huntley and Mackellar³²⁾ and Valderrábano et al. ⁽Reference Valderrábano, Delfa and Uriarte³⁴⁾.

Greer et al. ⁽Reference Greer, Huntley and Mackellar³²⁾ infected immunologically naive Coopworth ewe lambs with either control or 4000 T. circumcincta L₃ per d for 9 weeks, and offered them ad libitum access to food containing 10·5 MJ ME/kg DM and 146 g crude protein (CP)/kg DM. Coopworth lambs were assumed to be similar in terms of their growth characteristics to the sheep used for our simulations.

Valderrábano et al. ⁽Reference Valderrábano, Delfa and Uriarte³⁴⁾ infected immunologically naive Rasa Aragonesa female lambs with either 0 or 1000 T. circumcincta L₃ per d for 6 weeks, and offered ad libitum access to food containing 13·25 MJ ME/kg DM and 175·5 g CP/kg DM. Genetic descriptions of Rasa Aragonesa lambs in the terms required by our model do not appear to exist in the literature. For this reason the model was calibrated for the growth rates and food intake of the non-infected sheep; thus the performance and food intake of the infected sheep were model predictions.

Results

Validation

The model predictions for anorexia and FEC were close to those reported by Greer et al. ⁽Reference Greer, Huntley and Mackellar³²⁾; however, impacts of parasitism on growth rate were under-predicted. The model predicted a reduction in the food intake of infected lambs of 0·16 from 15 to 28 d post-infection in comparison with uninfected lambs; this is similar to the reported 0·17 reduction in the intake of the infected lambs over the same time period. Infected lambs were predicted to have a 26 % slower growth rate than uninfected lambs until day 38 post-infection, after which growth rates became similar. Infected lambs were predicted to remain proportionately 0·09 lighter than control lambs at 63 d post-infection. The reported growth rate for infected sheep was 43 % lower than that of uninfected sheep up to day 35 of infection and infected lambs were proportionately 0·11 lighter at 63 d post-infection. FEC was predicted to peak 30 d post-infection, and the observed FEC peak occurred 28 d post-infection.

Simulations reproducing the experiment of Valderrábano et al. ⁽Reference Valderrábano, Delfa and Uriarte³⁴⁾ predicted an average food intake of 1060 g/d for infected and 999 g/d for infected sheep, showing a 6 % reduction in the average food intake of infected lambs in comparison with their uninfected counterparts over the experimental period. These compare favourably with the reported intakes of 1070 and 960 g/d, respectively, although the observed reduction in the average food intake of infected lambs was slightly higher (10 %). Some of this difference could be due to the assumptions made to convert the reported CP content of the feed to MP.

Food intake

The daily food intakes for uninfected lambs are shown in Fig. 3 for foods differing in both energy and protein content (a), for the three isoenergetic foods (b) and for the three isonitrogenous foods (c). Figure 4 shows the food intake for lambs challenged with control, 1000 or 5000 larvae per d offered access to either food 4 for mechanism 1 (Fig. 1(a)) and for mechanism 2 (Fig. 1(b)) or food 1 for mechanism 1 (Fig. 1(c)) and for mechanism 2 (Fig. 1(d)). Average food intake predictions for uninfected lambs, and the relative food intake of lambs challenged with 1000 or 5000 larvae per d (given as a proportion of uninfected lambs) for both anorexia mechanisms and for all foods are summarised in Table 2. The maximum extent of anorexia (largest reduction predicted in comparison with uninfected lambs), including the day at which the maximum extent was observed, and the duration of anorexia are summarised in Table 3. Duration was defined as the number of days during which the proportional reduction in food intake was greater than 0·05.

Fig. 3 Food intake predictions for uninfected lambs given ad libitum access to foods of different crude protein (CP) and metabolisable energy (ME) content (for details of foods, see Table 1). (a) Foods of different CP and ME contents (, Food 1; , food 4; , food 7). (b) Foods of different CP content but the same ME content (10 MJ/kg DM) (, Food 2; , food 4; , food 6). (c) Foods of different ME content but the same CP content (140 g/kg DM) (, Food 3; , food 4; , food 5).

Fig. 4 (a) Food intake predictions for mechanism 1 (reduction in the intrinsic capacity for growth) for lambs given access to food 4 (crude protein = 140 g/kg DM; metabolisable energy = 10 MJ/kg DM), whilst exposed to control (), 1000 () or 5000 () Teladorsagia circumcincta L₃ per d. (b) Food intake predictions for mechanism 2 (direct reduction in food intake) for lambs given access to food 4. (c) Food intake predictions for mechanism 1 for lambs given access to food 1 (crude protein = 90 g/kg DM; metabolisable energy = 7·5 MJ/kg DM). (d) Food intake predictions for mechanism 2 for lambs given access to food 1.

Table 2 Average food intake (kg) predictions for uninfected (control) lambs, and total relative food intake predictions for mechanisms 1 and 2 for lambs given access to foods of different crude protein and metabolisable energy content and exposed to 1000 or 5000 Teladorsagia circumcincta L₃ per d over 121 d

* For details of foods, see Table 1.

† Total food intake given as a proportion of that of uninfected lambs.

‡ Reduction in the intrinsic capacity for growth.

§ Direct reduction in food intake.

Table 3 Maximum extent of anorexia and duration of anorexia predictions for mechanisms 1 and 2 for lambs given access to foods of different crude protein and metabolisable energy content and exposed to 1000 or 5000 Teladorsagia circumcincta L₃ per d over 121 d

LRP, largest reduction predicted.

* For details of foods, see Table 1.

† Reduction in the intrinsic capacity for growth.

‡ Direct reduction in food intake.

§ The number of days during which reduction was greater than 0·05.

‖ Anorexia still present at day 121.

Across all foods investigated, with the exception of the food low in both protein and energy (food 1), food intake for infected lambs presented similar patterns for both mechanisms of anorexia, differing only in the predicted the duration and extent of anorexia; the extent of anorexia was greater for larger levels of challenge. In general, mechanism 2 had a greater maximum extent of anorexia and lower relative food intake than mechanism 1 for both levels of challenge and for all foods. For mechanism 1 the duration of anorexia remained similar for both levels of infection, whilst for mechanism 2 for all foods the duration of anorexia was longer for lambs challenged with 1000 larvae per d than for lambs challenged with 5000 larvae per d.

Comparisons between foods for both mechanisms are explored in more detail below.

Effect of nitrogen content on food intake (foods 2, 4 and 6)

The effect of N content on food intake of uninfected lambs was small (Fig. 3(b)), with the exception of the food intake of the lambs on the lowest-N food during the early stages of growth (food 2). The model predicted that lambs offered access to food 2 would compensate for the food N content by increasing their food intake for the first 28 d of the simulated experiment, when this food was first limiting in MP.

For infected lambs, N content of the food had different impacts on relative food intake, and the maximum extent and duration of anorexia for the two mechanisms. Little effect of the N content of the food was seen on relative food intake, and the maximum extent and duration of anorexia for mechanism 1. However, for mechanism 2 the N content of the food affected the duration of anorexia for lambs challenged with 5000 larvae per d. The duration of anorexia decreased from 84 to 74 d as food CP content increased from 115 to 165 g/kg DM, although the maximum extent of anorexia was not affected significantly by food N content, remaining at about 0·26 for all three foods. For lambs challenged with 5000 larvae per d, food was predicted to increase from 0·88 to 0·9 as food CP content increased from 115 to 165 g/kg DM. However, similar relationships between anorexia traits and N content of the food were not observed in lambs challenged with 1000 larvae per d, with anorexia still being present at the end of the simulation.

Effect of energy content on food intake (foods 3, 4 and 5)

The effect of energy content on the food intake of uninfected lambs was large (Fig. 3(c)). This was due to the model predicting that lambs would compensate for a reduction in food energy content by increasing food intake.

For mechanism 1, the energy content of the food had little impact on the maximum extent of anorexia for both levels of parasitic challenge (Table 3); however, differences were predicted in the duration of anorexia for both levels of challenge. For lambs challenged with 1000 larvae per d the duration of anorexia was predicted to increase from 57 to 82 d as food ME content increased from 8·75 to 11·25 MJ/kg DM, whilst for lambs challenged with 5000 larvae per d the equivalent predicted increase in the duration of anorexia was from 73 to 82 d. Likewise, for lambs challenged with 1000 larvae per d the relative food intake was predicted to decrease from 0·95 to 0·93 as food ME content increased from 8·75 to 11·25 MJ/kg DM. For lambs challenged with 5000 larvae per d the equivalent predicted decrease in relative food intake was from 0·91 to 0·89.

For mechanism 2, the energy content of the feed affected the maximum extent and duration of anorexia for lambs challenged with 5000 larvae per d. The maximum extent of anorexia was predicted to be the same for foods 4 and 5 (0·27), whilst for food 3 the maximum extent of anorexia increased to 0·36. The duration of anorexia was similar (77 to 78 d) for foods 3 and 4, whilst for food 5 the duration of anorexia increased to 87 d. The relative food intakes of foods 3 and 5 were the same (0·87), whilst for food 4 (10 MJ/kg DM) the relative food intake was predicted to be 0·89. Similar relationships were not observed in lambs challenged with 1000 larvae per d, as anorexia was still present at the end of the simulation.

Effect of varying both energy and protein content on food intake (foods 1, 4 and 7)

For uninfected lambs, food intakes when offered foods 4 and 7 reflected the relative energy densities of the diets; however, bulk constraints due to the maximum capacity of the gastrointestinal tract were observed for food 1 (Fig. 3(a)).

For mechanism 1, there was little difference between the food intake characteristics of lambs offered food 4 or 7 when challenged with 1000 larvae per d, or between these foods when challenged with 5000 larvae per d. However, predicted food intake patterns differed substantially in the case of lambs offered food 1. Both larval challenge levels were predicted to result in the same maximum extent (0·08), relative food intake (0·96) and duration of anorexia (48 to 49 d). For food 1, the severity and extent of anorexia were less than those seen for the other foods.

For mechanism 2, lambs challenged with 5000 larvae per d and offered either food 4 or 7 had similar food intake and anorexia characteristics, as did lambs challenged with 1000 larvae per d. However, the food intake predictions for lambs offered food 1 differed substantially, as shown in Fig. 4(d). Lambs challenged with 5000 larvae per d and offered food 1 were predicted to have a maximum extent of 0·38, whilst lambs challenged with 1000 larvae per d were predicted to have a maximum extent of anorexia of 0·28. For both levels of challenge, the duration of anorexia was longer for food 1 than for all other foods, such that anorexia was still present at the end of the simulated time period. Lambs challenged with 5000 larvae per d and offered food 1 had a relative food intake of 0·73, whilst lambs challenged with 1000 larvae per d were predicted to have a relative food intake of 0·83.

Effect of differing levels of parasitic challenge on anorexia

The maximum extent of anorexia for lambs given access to food 4 for increasing levels of parasite challenge is given in Fig. 5, with predictions given for both mechanisms. The maximum extent of anorexia showed a non-linear increase with increasing challenge level, being on average 17 % greater for mechanism 2 than mechanism 1.

Fig. 5 Maximum extent of anorexia (i.e. the largest reduction predicted in comparison with uninfected lambs) predictions for mechanism 1 (reduction in the intrinsic capacity for growth; ) and mechanism 2 (direct reduction in food intake; ) for lambs given access to food 4 (crude protein = 140 g/kg DM; metabolisable energy = 10 MJ/kg DM), whilst exposed to increasing levels of larval challenge.

Daily egg counts

The predicted daily egg counts for infected lambs for food 4 (mechanism 2) are provided in Fig. 6, as an example of the profile for all foods. Whilst total egg count was always higher for the higher challenge level, differences between foods were often small. The maximum daily egg count predicted (including day of occurrence) for mechanisms 1 and 2 for lambs offered access to all foods, whilst challenged with either 1000 or 5000 larvae per d, are summarised in Table 4.

Fig. 6 Daily egg count (eggs/d) prediction for lambs given access to food 4 (crude protein = 140 g/kg DM; metabolisable energy = 10 MJ/kg DM) for mechanism 2 (direct reduction in food intake), whilst exposed to either 1000 () or 5000 () Teladorsagia circumcincta L₃ per d.

Table 4 Maximum daily egg count (10⁻³ eggs/d) predictions for mechanisms 1 and 2 for lambs given access to foods of different crude protein and metabolisable energy content and exposed to 1000 or 5000 Teladorsagia circumcincta L₃ per d

* For details of foods, see Table 1.

† Reduction in the intrinsic capacity for growth.

‡ Direct reduction in food intake.

Effect of nitrogen content on daily egg count (foods 2, 4 and 6)

Results from mechanism 1 suggested that the protein content of the food would have no impact upon the maximum daily egg count predicted for either level of larval challenge. Results from mechanism 2 similarly predicted no impact on maximum daily egg count for lambs given a challenge of 1000 larvae per d. However, for the lambs challenged with 5000 larvae per d, the protein content of the food had a small impact upon the predicted maximum daily egg count, with a 1·5 % increase for food 4 and a 6 % increase for food 2 in comparison with food 6.

Effect of energy content on daily egg count (foods 3, 4 and 5)

For lambs challenged with 1000 larvae per d, essentially no differences were predicted in maximum daily egg count for foods 3, 4 and 5, for both mechanisms. For lambs challenged with 5000 larvae per d, mechanism 1 resulted in a 3 % increase in predicted maximum egg counts for food 5 in comparison with foods 3 and 4. Mechanism 2 led to a 1·5 % increase for food 4 and a 15 % increase for food 5 in comparison with the maximum daily egg count predicted for food 3.

Effect of varying both energy and protein content on daily egg count (foods 1, 4 and 7)

For mechanism 1, no differences in maximum daily egg count were predicted for foods 4 and 7. However, for food 1, daily egg counts were predicted to be 20 and 34 % greater for lambs challenged with 1000 and 5000 larvae per d, respectively. For mechanism 2, lambs challenged with 1000 larvae per d were predicted to have no differences in maximum daily egg count for foods 4 and 7 and a 26 % increase for food 1 in comparison with the other foods. Lambs challenged with 5000 larvae per d were predicted to have a 1·5 % increase for food 4 and a 56 % increase for food 1 in comparison with the maximum daily egg count predicted for food 7.

Discussion

The aim of the present paper was to investigate the consequences of two proposed mechanisms for parasite-induced anorexia on the food intake of parasitised sheep, and to explore the relationship between anorexia and food composition. In addition to exploring these results we will also compare our predictions to appropriate, published experimental data. The comparisons are qualitative as there are no experiments in the literature that have investigated the effect of food composition on the food intake of sheep infected with T. circumcincta; however, they do enable us to draw conclusions about the nature of anorexia in parasitised sheep. We conclude by proposing experiments that need to be performed in order to gain further understanding of the nature of parasite-induced anorexia and its relationship to feed composition.

Accounting for the predictions made by each mechanism

Mechanism 1

Anorexia was observed on all foods with the exception of food 1. This was due to the energy content of the food being low, so that although lambs attempted to eat sufficient quantities of the food to meet energy requirements, they were constrained in doing so by their maximum gastrointestinal tract capacity. Whilst the desired food intake for growth was reduced due to parasitism, the maximum gastrointestinal tract capacity caused a constraint greater than this and consequently anorexia was not observed. However, a 4 % reduction in total food intake (Table 2) was still predicted for food 1. This was due to the lamb being unable to compensate for nutrient loss due to parasitism because of the maximum gastrointestinal tract capacity. Therefore the implication of mechanism 1 is that the food intake of the animals will be dictated by the first operating constraint, which in this case was gut fill⁽Reference Kyriazakis, Wiseman, Varley and Kemp³⁷⁾.

For lambs challenged with 5000 larvae per d the maximum extent of anorexia was about 0·23 in all remaining foods, in comparison with uninfected control lambs, and for lambs challenged with 1000 larvae per d the maximum extent of anorexia was about 0·10. The marginally increased maximum reduction in food intake and duration of anorexia predicted for food 5 is discussed below. The duration of anorexia for both levels of larval challenge was unaffected by the N content of the food; however, in both cases the duration of anorexia increased with the increasing energy content of the food.

The maximum daily egg count for infected lambs on all foods was the same except for foods 1 and 5. For lambs offered food 1 the increases predicted for both challenge levels arise from the maximum gastrointestinal tract capacity. Due to this, the food intake of the lambs did not meet the intake required for growth rate and the acquisition of immunity. Therefore a reduction in the rate of acquisition of immunity allowed more worms to establish, survive and produce eggs in comparison with foods 2, 3, 4, 6 and 7; subsequently the daily egg count increased. Intake of food 5 was not constrained by the maximum gastrointestinal tract capacity; nevertheless increases of 1 and 3 % in the maximum daily egg count were predicted for challenge levels of 1000 and 5000 larvae per d, respectively, in comparison with foods 2, 3, 4, 6 and 7. For each of these latter diets, if a lamb eats to meet its desired energy intake then it receives an excess of protein. Hence, with the reduction in the intrinsic growth rate with mechanism 1, the immune function will receive sufficient protein to achieve the optimal rate of acquisition even when anorexia is present. However, if the ME content of the food is high and the MP:ME ratio is low, as seen for food 5, a marginal deficiency in protein intake occurs in our model when anorexia is present. As a consequence, with this diet the model predicted a reduction in both growth rate and the acquisition rate of immunity (hence an increased duration of anorexia), leading to an increase in parasite burden.

Mechanism 2

Anorexia was observed for all foods at both challenge levels. For lambs given a challenge of 1000 larvae per d no differences were predicted in the maximum extent of anorexia for foods 3, 4, 6 and 7. The maximum extent of anorexia was largest for food 1 due to the added impact of the gut capacity, whilst foods 2 and 5 were predicted to have a small increase in comparison with foods 3, 4, 6 and 7. Similar differences were predicted for the duration of anorexia; however, further conclusions could not be drawn for this level of challenge, as anorexia was not complete by the end of the simulation.

For lambs challenged with 5000 larvae per d, the maximum extent of anorexia predicted was similar for foods 2, 4, 5, 6 and 7, but higher for foods with low ME contents (i.e. foods 1 and 3). These effects are attributable to the maximum gastrointestinal capacity constraint predicted throughout the simulation for food 1 and from day 78 for food 3. The duration of anorexia generally decreased with increasing N content of the diet, the exception being an increase in duration for food 5, due to the impact of a low MP:ME ratio on protein intake described above. A marked increase in duration of anorexia for food 1 can be attributed to the gut fill constraint preventing food intake recovery. The maximum daily egg count predictions followed a similar pattern, with a 15 % increase predicted for food 5 and a 56 % increase predicted for food 1.

Summary of differences between mechanisms

The two mechanisms resulted in different predicted outcomes and implications. For mechanism 1, the energy content of the food had an impact on the duration of anorexia, and the maximum extent and duration of anorexia were affected by the combination of a high ME content and a low MP:ME ratio (food 5). Other than this, no relationships were observed between anorexia and food composition, except in the presence of the maximum gastrointestinal tract capacity (food 1). The absence of anorexia for food 1 implies that the food intake of the animal is dictated by the first operating constraint, which in this case was the gut fill.

For mechanism 2, the N content of the food had an impact on the duration of anorexia, and the maximum extent and duration of anorexia were affected by the combination of a high ME content and a low MP:ME ratio (food 5). Further to this, the maximum gastrointestinal tract capacity and anorexia constraints were additive, as can be seen in the predictions for infected lambs offered access to food 1.

Comparison of predictions with experimental evidence

Whilst experiments have been performed quantifying impacts of T. circumcincta infection in sheep⁽Reference Coop, Sykes and Angus¹^, Reference Coop, Graham and Jackson³³⁾, the experimental data reported did not allow us to draw conclusions about the relationship between anorexia and food composition in parasitised sheep. However, data are available for Trichostrongylus colubriformis infections on a variety of different feeds. Although there are many differences between these two nematode species, for example, site of parasitism (abomasum v. small intestine), development rate, worm fecundity and acquisition of host immunity⁽Reference Coop and Kyriazakis³¹⁾, for purposes of comparison it is assumed that the anorexinogenic components of the immune response involved in T. colubriformis infections are similar to those for T. circumcincta ⁽Reference Kyriazakis, Tolkamp and Hutchings⁹^, Reference Kyriazakis¹⁷⁾, and consequently may be affected by food composition in a similar manner.

Surprisingly, there are few experiments that have investigated the effects of food energy content on the extent of anorexia; hence it is not possible to draw strong conclusions. However, the effect of protein content on the extent of anorexia has been investigated. First, Greer et al. ⁽Reference Greer, Sedcole and Jay³⁾ infected immunologically naive lambs with 2000 T. colubriformis larvae per d and gave them access to either a high-protein diet (energy = 10·5 MJ/kg DM; CP = 175 g/kg DM) or a low-protein diet (energy = 11·1 MJ/kg DM; CP = 93 g/kg DM). The maximum daily egg count at day 42 was 400 000 eggs for lambs fed the high-protein diet, and 700 000 eggs for the low-protein diet. The mean reduction in food intake over the period that anorexia was observed was 0·25 and 0·15 for lambs fed the low- and high-protein food, respectively, in comparison with uninfected control lambs. To compare these results with our model predictions, we ran simulations for both anorexia mechanisms using feed descriptions, live-weight range and level of larval challenge similar to Greer et al. ⁽Reference Greer, Sedcole and Jay³⁾; the only difference was that our simulations assumed T. circumcincta infections. Mechanism 1 predicted that the maximum daily egg count remained at about 289 000 eggs for both the high- and low-protein food. The mean reduction in food intake over the period that anorexia was observed was 0·09 and 0·10 for the low- and high-protein-fed lambs, respectively, in comparison with uninfected lambs. On the other hand, mechanism 2 predicted that the maximum daily egg count was about 306 000 eggs for lambs fed the low-protein food, but this decreased to about 289 000 eggs for lambs fed the high-protein food. Further to this, the mean reduction in food intake over the period that anorexia was observed was predicted to be 0·12 and 0·10 for lambs fed the low- and high-protein food, respectively, in comparison with uninfected control lambs. In summary, Greer et al. ⁽Reference Greer, Sedcole and Jay³⁾ observed that the maximum daily egg count decreased by 75 %, and the mean reduction in food intake also decreased, as the protein content of the food increased. For mechanism 1, the maximum daily egg count remained constant despite the change in protein content, and the mean reduction in food intake increased as the protein content of the food increased. For mechanism 2, the maximum daily egg count increased and the mean reduction in food intake decreased, as the protein content of the food increased. Due to differences in the nematode species the comparisons made here are qualitative rather than quantitative. However, whilst the changes in maximum daily egg count and food intake were smaller than those observed by Greer et al. ⁽Reference Greer, Sedcole and Jay³⁾, it was mechanism 2 that resulted in the correct predicted patterns.

Second, Kyriazakis et al. ⁽Reference Kyriazakis, Oldham and Coop³⁸⁾ infected immunologically naive lambs with 2500 T. colubriformis larvae per d and offered them isoenergetic foods (10·4 MJ/kg DM) that differed in CP content (90, 164 or 214 g/kg DM). The protein content of the food had no impact upon the extent of anorexia, with the reduction in mean food intake being 0·10 in comparison with uninfected lambs for all foods. Again, we simulated these experimental conditions. Mechanism 1 predicted a reduction in mean food intake of 0·07 for lambs offered the low-protein diet in comparison with uninfected lambs, and a reduction in mean food intake of 0·08 for lambs offered the medium- or high-protein diets in comparison with the controls. Mechanism 2 predicted no impact upon the extent of anorexia, with all foods showing a reduction in mean food intake of 0·10 in comparison with uninfected lambs, the same as that reported by Kyriazakis et al. ⁽Reference Kyriazakis, Oldham and Coop³⁸⁾.

Last, Kyriazakis et al. ⁽Reference Kyriazakis, Anderson and Oldham³⁹⁾ infected immunologically naive lambs with 2500 T. colubriformis larvae per d, and offered them access to isoenergetic foods (10·4 MJ/kg DM) differing in their CP content (86 or 206 g/kg DM). The reduction in mean food intake was 0·18 and 0·11 for lambs offered the low- and high-protein diets, respectively, compared with uninfected lambs. For simulations carried out using the same food descriptions and level of larval challenge, over the same time period, mechanism 1 predicted a reduction in mean food intake of 0·11 and 0·12 for lambs offered the low- and high-protein foods, respectively, in comparison with uninfected lambs. With mechanism 2, reductions in mean food intake were predicted to be 0·14 and 0·10 for lambs offered the low- and high-protein diets, respectively, showing a similar trend to that reported by Kyriazakis et al. ⁽Reference Kyriazakis, Anderson and Oldham³⁹⁾. Thus, in all three cases investigated it was mechanism 2 that led to more accurate representations of the trends observed in the experimental data, predicting a relationship between food composition and the extent of anorexia, with impacts on daily egg counts.

Interpretation and implications of model predictions

In terms of the duration of anorexia, there is convincing evidence that food composition has an impact, with duration being reduced on high-quality foods⁽Reference Kyriazakis, Anderson and Oldham³⁹^–Reference Knox and Steel⁴¹⁾. This relationship between food composition and the duration of anorexia has been suggested to be due to food composition affecting the degree of expression of immunity in pathogen-challenged hosts⁽Reference Coop and Kyriazakis³¹⁾, subsequently leading to the observed effect on the duration of anorexia via an impact upon immunity and parasite burden. Thus, animals on poor-quality diets (for example, foods of low protein and energy content) may be expected to suffer proportionally more the consequences of infection than animals on good-quality diets (for example, foods of high protein and energy content)⁽Reference Coop and Kyriazakis³¹⁾. Unfortunately, mechanism 1 predicted the opposite of this, with the duration of anorexia tending to increase as the energy content of the food increased.

There is also evidence that the protein content of the feed affects the daily egg count, with lambs offered lower-protein feeds having a higher daily egg count than lambs offered a higher-protein feed⁽Reference Greer, Sedcole and Jay³⁾. This provides further support to the finding that food composition affects the degree of expression of immunity, with a consequent relationship between food composition and duration of anorexia as suggested above. Once again, these findings are consistent with mechanism 2 in which food composition affected daily egg counts and the duration of anorexia.

Unfortunately, no comparable experimental data could be found for infected lambs on diets of sufficiently low energy content to incur the maximum gastrointestinal tract capacity constraint. In studies that have used foods of sufficiently low quality to cause this constraint, for example, Anindo et al. ⁽Reference Anindo, Toé and Tembely⁴²⁾, insufficient detail has been given on food composition and there have been no uninfected control groups. This lack of data may reflect the view and practice that parasitised lambs need to be fed better-quality feeds; whilst this may be a fair conclusion, it may not always be possible in practice.

Although mechanism 2 has been more consistent with the experimental data, the lack of comparable experimental data for foods that impose the maximum gastrointestinal tract capacity constraint does not allow us to draw conclusions on the additivity of signals involved in the regulation of food intake. Mechanism 1 implies that food intake would be determined by the most limiting constraint. It has previously been suggested that the processes regulating appetite are disrupted by cytokine release that accompanies infection⁽Reference Langhans¹⁰^, Reference Plata-Salamán¹¹⁾, and thus there is a redundancy of the signals operating to regulate food intake⁽Reference Kyriazakis, Wiseman, Varley and Kemp³⁷⁾. On the other hand, mechanism 2 implies that there is an additivity in the effects of the signals that control voluntary food intake. It has previously been proposed that various satiety signals act additively to control voluntary food intake⁽Reference Forbes⁴³^, Reference Anil, Mbanya and Symonds⁴⁴⁾. Thus both mechanisms present viable approaches to describing the regulation of voluntary food intake, but conclusions cannot be drawn on this topic until the relevant experiments have been carried out.

Further experimental research would help determine the relationship between food composition and parasite-induced anorexia. Whilst several experiments have systematically investigated the effects of food protein content on the extent of anorexia and the impacts of parasitism, further experiments are required to investigate the impacts of food energy and protein content, separately and in conjunction. It would be of particular interest to perform these challenge experiments using T. circumcintca, both to obtain de novo data for sheep infected by this parasite and to provide data that may be compared with those obtained from sheep challenged with T. colubriformis. It would also be of interest to obtain data for foods of sufficiently low energy content as to impose the maximum gastrointestinal tract capacity constraint. This would enable us to determine whether the factors that regulate voluntary food intake act additively, and whether low larval challenges are sufficient to cause anorexia for low-quality foods.

In summary, it would be of great biological interest to better understand the causes and consequences of anorexia. The suggested experiments coupled with predictive models may allow us to achieve this.

Conclusion

The mechanism by which anorexia is modelled leads to different predicted outcomes from infection. Mechanism 1, reduced intrinsic growth with consequent reductions in food intake, led to predictions that the duration of anorexia increases with increasing energy content of the food, and that food intake is determined by the first operating constraint (maximum gastrointestinal tract capacity). Mechanism 2, a direct reduction in food intake, led to predictions that the duration of anorexia decreases with increasing protein content of the food, and that impacts of anorexia and the maximum gastrointestinal tract capacity upon voluntary food intake are possibly additive. Mechanism 2 was more consistent with the theories and experimental data presented for a wide range of food qualities.

Acknowledgements

Y. C. S. M. L. was funded by a Biotechnology and Biological Sciences Research Council (BBSRC) CASE studentship in conjunction with Merial. We would also like to acknowledge further financial support from Biosciences Knowledge Transfer Network (Biosciences KTN). We are grateful to Dr Andy Forbes of Merial for the support and encouragement provided to us throughout the course of this project, and Dr Andrea Doeschl-Wilson for helpful comments on the manuscript. The contribution from S. C. B. was funded by a BBSRC Institute Strategic Programme Grant. All three authors have contributed equally to the model construction, design and conduct of the experiments, and interpretation of the outcomes.

The authors declare no conflicts of interest.