Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-23T15:13:45.237Z Has data issue: false hasContentIssue false
  • English
  • Français

On Risk Processes with Stochastic Intensity function

Published online by Cambridge University Press:  29 August 2014

Jan Grandell
Jan Grandell*
Affiliation:
University of Stockholm
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we are going to study some properties of a stochastic process, which has been proposed by Cramér (1968) as a model of the claims arising in an insurance company. This process has been studied by Cox in a different context. A few elementary results, concerning moments, are given by Cox and Lewis (1966). The present paper will be a survey of some results derived by the author (1970:1) and (1970:2). For detailed proofs we refer to these papers.

Let λ(t) be a real-valued stochastic process, such that P{λ(t) < o} = o. We further assume that Eλ(t) = 1 and that Eλ2(t) < ∞ for every fixed value of t. We denote the covariance

The process λ(t) will play the role of an intensity function. That means, that for every fixed realization of the process, the probability of

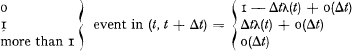
and that the number of events in disjoint intervals are independent.

We now define a point process N(t), where N(t) is the number of events which have occurred in (o, t]). With this definition we get

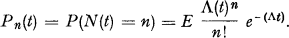
where

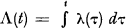
The integral is assumed to exist almost surely. This process will be called the N-process.

Type
Research Article
Information
ASTIN Bulletin: The Journal of the IAA , Volume 6 , Issue 2 , December 1971 , pp. 116 - 128
Copyright
Copyright © International Actuarial Association 1971

References

[1]Ammeter, H. (1948): A generalization of the collective theory of risk in regard to fluctuating basic probabilities. Skandinavisk Aktuarietidskrift.Google Scholar
[2]Cox, D. H. and Lewis, P. A. W. (1966): The Statistical Analysis of Events. Methuen.CrossRefGoogle Scholar
[3]Cramér, H. (1969): On streams of random events. Skandinavisck Antuarietidskrift. Suppl.Google Scholar
[4]Grandell, J. (1970:1): On stochastic processes generated by a stochastic intensity function. Research Report No. 49, Institute of Mathematical Statistics and Actuarial Mathematics, University of Stockholm.Google Scholar
[5]Grandell, J. (1970:2): On the estimation of intensities in a stochastic process generated by a stochastic intensity sequence. Research Report No. 54, Institute of Mathematical Statistics and Actuarial Mathematics, University of Stockholm.Google Scholar
[6]Grenander, U. and Szegö, G. (1958): Toeplitz forms and their applications. University of California Press.CrossRefGoogle Scholar
[7]Lundberg, O. (1940): On random processes and their application to sickness and accident statistics. Almqvist & Wicksell.Google Scholar
[8]Robbins, H. E. (1948): The asymptotic distribution of the sum of a random number of random variables. Bull. Amer. Math. Soc. Vol. 54.CrossRefGoogle Scholar