We are using the following terminology—essentially following Feller:

a) Compound Poisson Variable

This is a random variable

where X1, X2, … Xn, … independent, identically distributed (X0 = o) and N a Poisson counting variable

hence

(common) distribution function of the Xj with j ≠ 0 or in the language of characteristic functions

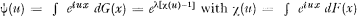
b) Weighted Compound Poisson Variable

This is a random variable Z obtained from a class of Compound Poisson Variables by weighting over λ with a weight function S(λ)

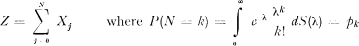
hence

or in the language of characteristic functions

Let [Z(t); t ≥ o] be a homogeneous Weighted Compound Poisson Process. The characteristic function at the time epoch t reads then

It is most remarkable that in many instances φt(u) can be represented as a (non weighted) Compound Poisson Variable. Our main result is given as a theorem.