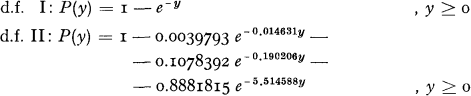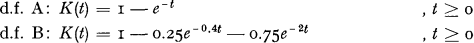Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Beekman, John A.
and
Bowers, Newton L.
1972.
An approximation to the finite time ruin function, part II.
Scandinavian Actuarial Journal,
Vol. 1972,
Issue. 2,
p.
128.
Beekman, John A.
1975.
Compound Poisson processes, as modified by Ornstein-Uhlenbeck processes.
Scandinavian Actuarial Journal,
Vol. 1975,
Issue. 4,
p.
226.
Beekman, John A.
1976.
Compound poisson processes, as modified by Ornstein-Uhlenbeck processes, Part II.
Scandinavian Actuarial Journal,
Vol. 1976,
Issue. 1,
p.
30.
Taylor, G. C.
1976.
Use of differential and integral inequalities to bound ruin and queuing probabilities.
Scandinavian Actuarial Journal,
Vol. 1976,
Issue. 4,
p.
197.
Wikstad, Nils
1977.
How to calculate ruin probabilities according to the classical risk theory.
Scandinavian Actuarial Journal,
Vol. 1977,
Issue. sup1,
p.
19.
Taylor, G. C.
1978.
Representation and explicit calculation of Finite-time ruin probabilities.
Scandinavian Actuarial Journal,
Vol. 1978,
Issue. 1,
p.
1.
De Vylder, Fl.
1978.
A practical solution to the problem of ultimate ruin probability.
Scandinavian Actuarial Journal,
Vol. 1978,
Issue. 2,
p.
114.
Janssen, J.
1980.
H. L. Seal, Survival Probabilities (The Goal of Risk Theory)Chichester: John Wiley & Sons Inc., 1978, x+ 103, $ 24.50..
ASTIN Bulletin,
Vol. 11,
Issue. 1,
p.
73.
Thorin, Olof
1982.
Probabilities of ruin.
Scandinavian Actuarial Journal,
Vol. 1982,
Issue. 2,
p.
65.
De Vylder, F.
and
Goovaerts, M.J.
1988.
Recursive calculation of finite-time ruin probabilities.
Insurance: Mathematics and Economics,
Vol. 7,
Issue. 1,
p.
1.
Dickson, David C. M.
and
Waters, Howard R.
1991.
Recursive Calculation of Survival Probabilities.
ASTIN Bulletin,
Vol. 21,
Issue. 2,
p.
199.
Steenackers, A.
and
Goovaerts, M.
1991.
A review of the numerical calculation of ruin probabilities by means of recursions.
Applied Stochastic Models and Data Analysis,
Vol. 7,
Issue. 1,
p.
77.
Kling, B.M.
and
Goovaerts, M.J.
1991.
A recursive evaluation of the finite time ruin probability based on an equation of Seal.
Insurance: Mathematics and Economics,
Vol. 10,
Issue. 2,
p.
93.
De Vylder, F.
and
Goovaerts, M.J.
1994.
A note on the solution of practical ruin problems.
Insurance: Mathematics and Economics,
Vol. 15,
Issue. 2-3,
p.
181.
De Vylder, F.
and
Marceau, E.
1996.
Classical numerical ruin probabilities.
Scandinavian Actuarial Journal,
Vol. 1996,
Issue. 2,
p.
109.
Usábel, Miguel
1999.
Calculating multivariate ruin probabilities via Gaver–Stehfest inversion technique.
Insurance: Mathematics and Economics,
Vol. 25,
Issue. 2,
p.
133.
Usábel, M.A.
1999.
Practical approximations for multivariate characteristics of risk processes.
Insurance: Mathematics and Economics,
Vol. 25,
Issue. 3,
p.
397.
De Vylder, F.Etienne
and
Goovaerts, Marc J.
1999.
Explicit finite-time and infinite-time ruin probabilities in the continuous case.
Insurance: Mathematics and Economics,
Vol. 24,
Issue. 3,
p.
155.
Grandell, Jan
2000.
Simple approximations of ruin probabilities.
Insurance: Mathematics and Economics,
Vol. 26,
Issue. 2-3,
p.
157.
Gyllenberg, Mats
and
S. Silvestrov, Dmitrii
2000.
Cramér–Lundberg approximation for nonlinearly perturbed risk processes.
Insurance: Mathematics and Economics,
Vol. 26,
Issue. 1,
p.
75.