Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Zayed, E. M. E.
1990.
Hearing the shape of a general doubly connected domain in R3 with impedance boundary conditions.
Journal of Mathematical Physics,
Vol. 31,
Issue. 10,
p.
2361.
Zayed, E. M. E.
and
Younis, A. I.
1994.
On hearing the shape of rectilinear regions.
Journal of Mathematical Physics,
Vol. 35,
Issue. 7,
p.
3490.
Zayed, E. M. E.
1995.
An inverse problem for a general multiply connected bounded domain.
Applicable Analysis,
Vol. 59,
Issue. 1-4,
p.
553.
Zayed, E.M.E.
and
Abdel-Halim, I.H.
2001.
The 3D inverse problem for waves with fractal and general annular bounded domain with piecewise smooth Robin boundary.
Chaos, Solitons & Fractals,
Vol. 12,
Issue. 12,
p.
2307.
Zayed, E.M.E.
and
Abdel-Halim, I.H.
2001.
The wave equation approach to the two-dimensional inverse problem for a general bounded domain with piecewise smooth mixed boundary conditions.
Acta Mathematica Scientia,
Vol. 21,
Issue. 2,
p.
171.
Zayed, E.M.E.
2001.
An inverse problem of the heat equation for a general multi-connected drum with applications in Physics.
Chaos, Solitons & Fractals,
Vol. 12,
Issue. 10,
p.
1861.
Zayed, E.M.E.
2001.
Short—time Asymptotics of the Heat Kernel of the Laplacian for a Multiply-connected Domain inR2with Robin Boundary Conditions.
Applicable Analysis,
Vol. 77,
Issue. 1-2,
p.
177.
Zayed, E.M.E.
and
Abdel-Halim, I.H.
2002.
An inverse problem of the wave equation for a general doubly connected region in with a finite number of piecewise smooth Robin boundary conditions.
Applied Mathematics and Computation,
Vol. 132,
Issue. 1,
p.
187.
Zayed, E.M.E.
2002.
An inverse problem for a general annular-bounded domain in with mixed boundary conditions and its physical applications.
Applied Mathematics and Computation,
Vol. 129,
Issue. 2-3,
p.
237.
Zayed, E. M. E.
2003.
Asymptotic Expansions of the Heat Kernel of the Laplacian for General Annular Bounded Domains with Robin Boundary Conditions: Further Results.
Acta Mathematica Sinica, English Series,
Vol. 19,
Issue. 4,
p.
679.
Zayed, E.M.E.
2003.
The wave equation approach to an inverse problem for a general multi-connected domain in R2 with mixed boundary conditions.
Applied Mathematics and Computation,
Vol. 144,
Issue. 2-3,
p.
457.
Zayed, E.M.E.
2003.
INVERSE PROBLEMS FOR A GENERAL MULTI-CONNECTED BOUNDED DRUM WITH APPLICATIONS IN PHYSICS.
Acta Mathematica Scientia,
Vol. 23,
Issue. 1,
p.
104.
Zayed, E.M.E.
2003.
On hearing the shape of a general multi-connected vibrating membrane in with piecewise smooth positive functions in the Robin boundary conditions.
Applied Mathematics and Computation,
Vol. 135,
Issue. 2-3,
p.
361.
Zayed, E.M.E.
2003.
Inverse problems for a general multi-connected bounded drum with applications in physics.
Applied Mathematics and Computation,
Vol. 139,
Issue. 2-3,
p.
231.
Zayed, E.M.E.
2004.
Short-time asymptotics of the two-dimensional wave equation for an annular vibrating membrane with applications in the mathematical physics.
Chaos, Solitons & Fractals,
Vol. 19,
Issue. 3,
p.
679.
Zayed, E.M.E
2004.
An inverse eigenvalue problem of the wave equation for a multi-connected region in R2 together with three different types of boundary conditions.
Applied Mathematics and Computation,
Vol. 154,
Issue. 2,
p.
361.
Zayed, E. M. E.
2004.
Short-time Asymptotics of the Heat Kernel on Bounded Domain with Piecewise Smooth Boundary Conditions and Its Applications to an Ideal Gas.
Acta Mathematicae Applicatae Sinica, English Series,
Vol. 20,
Issue. 2,
Zayed, E. M. E.
2004.
The Asymptotics of the Two-Dimensional Wave Equation for a General Multi-Connected Vibrating Membrane with Piecewise Smooth Robin Boundary Conditions.
Acta Mathematica Sinica, English Series,
Vol. 20,
Issue. 2,
p.
209.


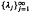 for the two-dimensional Laplacian using the asymptotic expansion of the spectral function
for the two-dimensional Laplacian using the asymptotic expansion of the spectral function  for small positive
for small positive 