Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-25T09:08:44.841Z Has data issue: false hasContentIssue false
  • English
  • Français

MODELLING OF RESISTIVE PULSE SENSING: FLEXIBLE METHODS FOR SUBMICRON PARTICLES

Part of: General models, approaches, and methods

Published online by Cambridge University Press:  06 June 2014

G. R. WILLMOTT  and
B. G. SMITH
G. R. WILLMOTT*
Affiliation:
The MacDiarmid Institute for Advanced Materials and Nanotechnology, Callaghan Innovation, PO Box 31310, Lower Hutt 5040, New Zealand
B. G. SMITH
Affiliation:
Callaghan Innovation, PO Box 2225, Auckland 1140, New Zealand email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Nanopore science, the study of individual nanoscale pores within thin membranes, is a fast-growing field which presents numerous interesting problems for physicists and applied mathematicians. Nanopores are most commonly applied to resistive pulse sensing (RPS) of individual particles suspended in aqueous electrolyte. The form of a resistive pulse is dependent on an array of experimental variables, including electrolyte characteristics, electrophoretic and convective transport, and (especially) pore and particle geometry. The level of analysis required depends on the application, but any broadly useful approach should be simple and flexible, due to the requirement for high data throughput and variations between different experimental systems and specimens. Here we review analytic methods for interpreting RPS experiments for particles in the approximate range 100 nm to 1 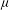$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mu $m, focusing on calculation of resistance change as a function of the particle’s position. We detail a recently developed semi-analytical model and compare the modelled electric field with finite element results. The model can also be used to calculate particle motion, so that the experimental current–time history can be reconstructed. This approach is useful for a wide range of pore and particle geometries, and includes consideration of entrance effects. Tunable elastomeric pores with truncated linear cone geometry are used as a model system.

Keywords

nanoporeresistive pulse sensingtunable pore

MSC classification

Secondary: 70G99: None of the above, but in this section
Type
Research Article
Information
The ANZIAM Journal , Volume 55 , Issue 3 , January 2014 , pp. 197 - 213
Copyright
Copyright © 2014 Australian Mathematical Society 

References

Ali, M., Ramirez, P., Mafé, S., Neumann, R. and Ensinger, W., “A pH-tunable nanofluidic diode with a broad range of rectifying properties”, ACS Nano 3 (2009) 603–608; doi:10.1021/nn900039f.Google Scholar
An, R., Uram, J. D., Yusko, E. C., Ke, K., Mayer, M. and Hunt, A. J., “Ultrafast laser fabrication of submicrometer pores in borosilicate glass”, Optics Letters 33 (2008) 1153–1155; doi:10.1364/OL.33.001153.Google Scholar
Bacri, L., Oukhaled, A. G., Schiedt, B., Patriarche, G., Bourhis, E., Gierak, J., Pelta, J. and Auvray, L., “Dynamics of colloids in single solid-state nanopores”, J. Phys. Chem. B 115 (2011) 2890–2898; doi:10.1021/jp200326w.Google Scholar
Benner, S., Chen, R. J. A., Wilson, N. A., Abu-Shumays, R., Hurt, N., Lieberman, K. R., Deamer, D. W., Dunbar, W. B. and Akeson, M., “Sequence-specific detection of individual DNA polymerase complexes in real time using a nanopore”, Nature Nanotech. 2 (2007) 718–724; doi:10.1038/nnano.2007.344.Google Scholar
Berge, L. I., Jossang, T. and Feder, J., “Off-axis response for particles passing through long apertures in Coulter-type counters”, Meas. Sci. Technol. 1 (1990) 471–474; doi:10.1088/0957-0233/1/6/001.CrossRefGoogle Scholar
Brar, S. K., “Measurement of nanoparticles by light-scattering techniques”, Trends Anal. Chem. 30 (2011) 4–17; doi:10.1016/j.trac.2010.08.008.Google Scholar
Cervera, J., Alcaraz, A., Schiedt, B., Neumann, R. and Ramirez, P., “Asymmetric selectivity of synthetic conical nanopores probed by reversal potential measurements”, J. Phys. Chem. C 111 (2007) 12265–12273; doi:10.1021/jp071884c.Google Scholar
Cervera, J., Ramirez, P., Mafé, S. and Stroeve, P., “Asymmetric nanopore rectification for ion pumping, electrical power generation, and information processing applications”, Electrochimica Acta 56 (2011) 4504–4511; doi:10.1016/j.electacta.2011.02.056.Google Scholar
Clarke, J., Wu, H.-C., Jayasinghe, L., Patel, A., Reid, S. and Bayley, H., “Continuous base identification for single-molecule nanopore DNA sequencing”, Nature Nanotech. 4 (2009) 265–270; doi:10.1038/nnano.2009.12.Google Scholar
Cockroft, S. L., Chu, J., Amorin, M. and Ghadiri, M. R., “A single-molecule nanopore device detects DNA polymerase activity with single-nucleotide resolution”, J. Am. Chem. Soc. 130 (2008) 818–820; doi:10.1021/ja077082c.Google Scholar
Constantin, D. and Siwy, Z. S., “Poisson–Nernst–Planck model of ion current rectification through a nanofluidic diode”, Phys. Rev. E 76 (2007) 041202; doi:10.1103/PhysRevE.76.041202.CrossRefGoogle ScholarPubMed
Cummings, E. B., Griffiths, S. K., Nilson, R. H. and Paul, P. H., “Conditions for similitude between the fluid velocity and electric field in electroosmotic flow”, Anal. Chem. 72 (2000) 2526–2532; doi:10.1021/ac991165x.Google Scholar
Dagan, Z., Weinbaum, S. and Pfeffer, R., “An infinite-series solution for the creeping motion through an orifice of finite length”, J. Fluid Mech. 115 (1982) 505–523; doi:10.1017/S0022112082000883.Google Scholar
Deamer, D. W. and Branton, D., “Characterization of nucleic acids by nanopore analysis”, Acc. Chem. Res. 35 (2002) 817–825; doi:10.1021/ar000138m.CrossRefGoogle ScholarPubMed
DeBlois, R. W. and Bean, C. P., “Counting and sizing of submicron particles by the resistive pulse technique”, Rev. Sci. Instrum. 41 (1970) 909–916; doi:10.1063/1.1684724.Google Scholar
DeBlois, R. W., Bean, C. P. and Wesley, R. K. A., “Electrokinetic measurements with submicron particles and pores by the resistive pulse technique”, J. Colloid. Interf. Sci. 61 (1977) 323–335; doi:10.1016/0021-9797(77)90395-2.Google Scholar
DeBlois, R. W. and Wesley, R. K. A., “Sizes and concentrations of several type C oncornaviruses and bacteriophage T2 by the resistive-pulse technique”, J. Virology 23 (1977) 227–233.CrossRefGoogle ScholarPubMed
Dekker, C., “Solid-state nanopores”, Nature Nanotech. 2 (2007) 209–215; doi:10.1038/nnano.2007.27.Google Scholar
Devasenathipathy, S. and Santiago, J. G., Electrokinetic flow diagnostics, in micro- and nano-scale diagnostic techniques (Springer-Verlag, New York, 2005).Google Scholar
Firnkes, M., Pedone, D., Knezevic, J., Döblinger, M. and Rant, U., “Electrically facilitated translocations of proteins through silicon nitride nanopores: Conjoint and competitive action of diffusion, electrophoresis, and electroosmosis”, Nano Lett. 10 (2010) 2162–2167; doi:10.1021/nl100861c.Google Scholar
Gregg, E. C. and Steidley, K. D., “Electrical counting and sizing of mammalian cells in suspension”, Biophys. J. 5 (1965) 393–405; doi:10.1016/S0006-3495(65)86724-8.Google Scholar
Grossman, P. D., Capillary electrophoresis (Academic Press, San Diego, CA, 1992).Google Scholar
Hall, J. E., “Access resistance of a small circular pore”, J. Gen. Physiol. 66 (1975) 531–532; doi:10.1085/jgp.66.4.531.Google Scholar
Han, A., Schürmann, G., Mondin, G., Bitterli, R. A., Hegelbach, N. G., de Rooij, N. F. and Staufer, U., “Sensing protein molecules using nanofabricated pores”, Appl. Phys. Lett. 88 (2006) 093901; doi:10.1063/1.2180868.Google Scholar
Happel, J. and Brenner, H., Low Reynolds number hydrodynamics (Noordhoff International, Leiden, The Netherlands, 1973); doi:10.1007/978-94-009-8352-6.Google Scholar
Heins, E. A., Siwy, Z. S., Baker, L. A. and Martin, C. R., “Detecting single porphyrin molecules in a conically shaped synthetic nanopore”, Nano Lett. 5 (2005) 1824–1829; doi:10.1021/nl050925i.Google Scholar
Heng, J. B., Aksimentiev, A., Ho, C., Marks, P., Grinkova, Y. V., Sligar, S., Schulten, K. and Timp, G., “Stretching DNA using the electric field in a synthetic nanopore”, Nano Lett. 5 (2005) 1883–1888; doi:10.1021/nl0510816.Google Scholar
Ito, T., Sun, L., Bevan, M. A. and Crooks, R. M., “Comparison of nanoparticle size and electrophoretic mobility measurements using a carbon-nanotube-based Coulter counter, dynamic light scattering, transmission electron microscopy, and phase analysis light scattering”, Langmuir 20 (2004) 6940–6945; doi:10.1021/la049524t.Google Scholar
Ito, T., Sun, L. and Crooks, R. M., “Simultaneous determination of the size and surface charge of individual nanoparticles using a carbon nanotube-based Coulter counter”, Anal. Chem. 75 (2003) 2399–2406; doi:10.1021/ac034072v.Google Scholar
Jagtiani, A. V., Carletta, J. and Zhe, J., “A microfluidic multichannel resistive pulse sensor using frequency division multiplexing for high throughput counting of micro particles”, J. Micromech. Microeng. 21 (2011) 065004; doi:10.1088/0960-1317/21/6/065004.Google Scholar
Jansen, M. L., Willmott, G. R., Hoek, I. and Arnold, W. M., “Fast piezoelectric actuation of an elastomeric micropore”, Measurement 46 (2013) 3560–3567; doi:10.1016/j.measurement.2013.05.023.Google Scholar
Kasianowicz, J. J., Brandin, E., Branton, D. and Deamer, D. W., “Characterization of individual polynucleotide molecules using a membrane channel”, Proc. Natl. Acad. Sci. USA 93 (1996) 13770–13773; doi:10.1073/pnas.93.24.13770.CrossRefGoogle ScholarPubMed
Kim, M. J., Wanunu, M., Bell, D. C. and Meller, A., “Rapid fabrication of uniformly sized nanopores and nanopore arrays for parallel DNA analysis”, Adv. Mater. 18 (2006) 3149–3153; doi:10.1002/adma.200601191.Google Scholar
Kirby, B. J. and Hasselbrink, E. F. Jr, “Zeta potential of microfluidic substrates: 1. Theory, experimental techniques, and effects on separations”, Electrophoresis 25 (2004) 187–202; doi:10.1002/elps.200305754.Google Scholar
Kowalczyk, S. W., Grosberg, A. Y., Rabinand, Y. and Dekker, C., “Modeling the conductance and DNA blockade of solid-state nanopores”, Nanotechnology 22 (2011) 315101; doi:10.1088/0957-4484/22/31/315101.Google Scholar
Kozak, D., Anderson, W., Vogel, R. and Trau, M., “Advances in resistive pulse sensors: Devices bridging the void between molecular and microscopic detection”, Nano Today 6 (2011) 531–545; doi:10.1016/j.nantod.2011.08.012.Google Scholar
Lan, W. J., Holden, D. A., Liu, J. and White, H. S., “Pressure-driven nanoparticle transport across glass membranes containing a conical-shaped nanopore”, J. Phys. Chem. C 115 (2011) 18445–18452; doi:0.11021/jp204839j.Google Scholar
Lan, W. J., Holden, D. A., Zhang, B. and White, H. S., “Nanoparticle transport in conical-shaped nanopores”, Anal. Chem. 83 (2011) 3840–3847; doi:10.1021/ac200312n.Google Scholar
Lee, S., Zhang, Y., White, H. S., Harrell, C. C. and Martin, C. R., “Electrophoretic capture and detection of nanoparticles at the opening of a membrane pore using scanning electrochemical microscopy”, Anal. Chem. 76 (2004) 6108–6115; doi:10.1021/ac049147p.Google Scholar
Liebes, Y., Drozdov, M., Avital, Y. Y., Kauffmann, Y., Rapaport, H., Kaplan, W. D. and Ashkenasy, N., “Reconstructing solid state nanopore shape from electrical measurements”, Appl. Phys. Lett. 97 (2010) 223105; doi:10.1063/1.3521411.Google Scholar
Low, M., Yu, S., Han, M. Y. and Su, X., “Investigative study of nucleic acid–gold nanoparticle interactions using laser-based techniques, electron microscopy, and resistive pulse sensing with a nanopore”, Aust. J. Chem. 64 (2011) 1229–1234; doi:10.1071/CH11200.Google Scholar
Platt, M., Willmott, G. R. and Lee, G. U., “Resistive pulse sensing of analyte-induced multicomponent rod aggregation using tunable pores”, Small 8 (2012) 2436–2444; doi:10.1002/smll.201200058.CrossRefGoogle ScholarPubMed
Qin, Z., Zhe, J. and Wang, G. X., “Effects of particle’s off-axis position, shape, orientation and entry position on resistance changes of micro Coulter counting devices”, Meas. Sci. Technol. 22 (2011) 045804; doi:10.1088/0957-0233/22/4/045804.Google Scholar
Ramírez, P., Apel, P. Y., Cervera, J. and Mafé, S., “Pore structure and function of synthetic nanopores with fixed charges: Tip shape and rectification properties”, Nanotechnology 19 (2008) 315707; doi:10.1088/0957-4484/19/31/315707.Google Scholar
Rice, C. L. and Whitehead, R., “Electrokinetic flow in a narrow cylindrical capillary”, J. Phys. Chem. 69 (1965) 4017–4025; doi:10.1021/j100895a062.CrossRefGoogle Scholar
Roberts, G. S., Kozak, D., Anderson, W., Broom, M. F., Vogel, R. and Trau, M., “Tunable nano/micropores for particle detection and discrimination: Scanning ion occlusion spectroscopy”, Small 6 (2010) 2653–2658; doi:10.1002/smll.201001129.Google Scholar
Roberts, G. S., Yu, S., Zeng, Q., Chan, L. C. L., Anderson, W., Colby, A. H., Grinstaff, M. W., Reid, S. and Vogel, R., “Tunable pores for measuring concentrations of synthetic and biological nanoparticle dispersions”, Biosens. Bioelectron. (2012); doi:10.1016/j.bios.2011.09.040.Google Scholar
Saleh, O. A. and Sohn, L. L., “An artificial nanopore for molecular sensing”, Nano Lett. 3 (2003) 37–38; doi:10.1021/nl0255202.Google Scholar
Sampson, R. A., “On Stokes’s current function”, Phil. Trans. R. Soc. Lond. A 182 (1891) 449–518; doi:10.1098/rsta.1891.0012.Google Scholar
Santiago, J. G., “Electroosmotic flows in microchannels with finite inertial and pressure forces”, Anal. Chem. 73 (2001) 2353–2365; doi:10.1021/ac0101398.Google Scholar
Schoch, R. B., Han, J. and Renaud, P., “Transport phenomena in nanofluidics”, Rev. Mod. Phys. 80 (2008) 839–883; doi:10.1103/RevModPhys.80.839.Google Scholar
Sexton, L. T., Horne, L. P. and Martin, C. R., “Developing synthetic conical nanopores for biosensing applications”, Mol. BioSyst. 3 (2007) 667–685; doi:10.1039/b708725j.Google Scholar
Siwy, Z. S. and Davenport, M., “Making nanopores from nanotubes”, Nat. Nanotech. 5 (2010) 174–175; doi:10.1038/nnano.2010.33.Google Scholar
Smythe, W. R., “Flow around the spheroid in a circular tube”, Phys. Fluids 7 (1964) 633–638; doi:10.1063/1.1711260.Google Scholar
Song, Y., Zhang, H., Chon, C. H., Pan, X. and Li, D., “Nanoparticle detection by microfluidic resistive pulse sensor with a submicron sensing gate and dual detecting channels—two stage differential amplifier”, Sens. Actuators B 155 (2011) 930–936; doi:10.1016/j.snb.2011.01.004.Google Scholar
Sowerby, S. J., Broom, M. F. and Petersen, G. B., “Dynamically resizable nanometre-scale apertures for molecular sensing”, Sens. Actuators B 123 (2007) 325–330; doi:10.1016/j.snb.2006.08.03.Google Scholar
Steinbock, L. J., Stober, G. and Keyser, U. F., “Sensing DNA-coatings of microparticles using micropipettes”, Biosens. Bioelectron. 24 (2009) 2423–2427; doi:10.1016/j.bios.2008.12.026.Google Scholar
Stober, G., Steinbock, L. J. and Keyser, U. F., “Modeling of colloidal transport in capillaries”, J. Appl. Phys. 105 (2009) 084702; doi:10.1063/1.3095761.Google Scholar
Sun, L. and Crooks, R. M., “Single carbon nanotube membranes: A well-defined model for studying mass transport through nanoporous materials”, J. Am. Chem. Soc. 122 (2000) 12340–12345; doi:10.1021/ja002429w.Google Scholar
van Dorp, S., Keyser, U. F., Dekker, N. H., Dekker, C. and Lemay, S. G., “Origin of the electrophoretic force on DNA in solid-state nanopores”, Nature Phys. 5 (2009) 347–351; doi:10.1038/nphys1230.Google Scholar
Vogel, R., Anderson, W., Eldridge, J., Glossop, B. and Willmott, G. R., “A variable pressure method for characterizing nanoparticle surface charge using pore sensors”, Anal. Chem. 84 (2012) 3125–3132; doi:10.1021/ac2030915.CrossRefGoogle ScholarPubMed
Vogel, R., Willmott, G. R., Kozak, D., Roberts, G. S., Anderson, W., Groenewegen, L., Glossop, B., Barnett, A., Turner, A. and Trau, M., “Quantitative sizing of nano/microparticles with a tunable elastomeric pore sensor”, Anal. Chem. 83 (2011) 3499–3506; doi:10.1021/ac200195n.Google Scholar
Wang, G., Zhang, B., Wayment, J. R., Harris, J. M. and White, H. S., “Electrostatic-gated transport in chemically modified glass nanopore electrodes”, J. Am. Chem. Soc. 128 (2006) 7679–7686; doi:10.1021/ja061357r.Google Scholar
Wang-yi, W. and Skalak, R., “The Stokes flow from half-space into semi-infinite circular cylinder”, Appl. Math. Mech. 6 (1985) 9–23; doi:10.1007/BF01895679.Google Scholar
Washburn, E. W., “The dynamics of capillary flow”, Phys. Rev. 17 (1921) 273–283; doi:10.1103/PhysRev.17.273.Google Scholar
Wharton, J. E., Jin, P., Sexton, L. T., Horne, L. P., Sherrill, S. A., Mino, W. K. and Martin, C. R., “A method for reproducibly preparing synthetic nanopores for resistive-pulse biosensors”, Small 3 (2007) 1424–1430; doi:10.1002/smll.200700106.Google Scholar
Willmott, G. R., Broom, M. F., Jansen, M. L., Young, R. M. and Arnold, W. M., Tunable elastomeric pores, in molecular- and nano-tubes (Springer-Verlag, Berlin, 2011).Google Scholar
Willmott, G. R., Chaturvedi, R., Cummins, S. and Groenewegen, L. G., “Actuation of tunable elastomeric pores: Resistance measurements and finite element modelling”, Exper. Mech. (2013); doi:10.1007/s11340-013-9795-5.Google Scholar
Willmott, G. R. and Moore, P W., “Reversible mechanical actuation of elastomeric nanopores”, Nanotechnology 19 (2008) 475504; doi:10.1088/0957-4484/19/47/475504.Google Scholar
Willmott, G. R. and Parry, B. E. T., “Resistive pulse asymmetry for nanospheres passing through tunable submicron pores”, J. Appl. Phys. 109 (2011) 094307; doi:10.1063/1.3580283.Google Scholar
Willmott, G. R., Platt, M. and Lee, G. U., “Resistive pulse sensing of magnetic beads and supraparticle structures using tunable pores”, Biomicrofluidics 6 (2012) 014103; doi:10.1063/1.3673596.Google Scholar
Willmott, G. R. and Smith, B. G., “Comment on ‘Modeling the conductance and DNA blockade of solid-state nanopores’”, Nanotechnology 23 (2012) 088001; doi:10.1088/0957-4484/23/8/088001.CrossRefGoogle ScholarPubMed
Willmott, G. R., Vogel, R., Yu, S. S. C., Groenewegen, L. G., Roberts, G. S., Kozak, D., Anderson, W. and Trau, M., “Use of tunable nanopore blockade rates to investigate colloidal dispersions”, J. Phys.: Condens. Matter 22 (2010) 454116; doi:10.1088/0953-8984/22/45/454116.Google Scholar
Willmott, G. R., Yu, S. S. C. and Vogel, R., “Pressure dependence of particle transport through resizable nanopores”, Proceedings of ICONN 2010, Sydney, Australia; doi:10.1109/ICONN.2010.6045207.Google Scholar
Wu, S., Park, S. R. and Ling, X. S., “Lithography-free formation of nanopores in plastic membranes using laser heating”, Nano Lett. 6 (2006) 2571–2576; doi:10.1021/nl0619498.Google Scholar