Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-23T10:17:23.523Z Has data issue: false hasContentIssue false
  • English
  • Français

A EUROPEAN OPTION GENERAL FIRST-ORDER ERROR FORMULA

Part of: Applications Mathematical finance

Published online by Cambridge University Press:  04 September 2013

GUILLAUME LEDUC
GUILLAUME LEDUC*
Affiliation:
American University of Sharjah, PO Box 26666, Sharjah, UAE email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

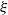We study the value of European security derivatives in the Black–Scholes model when the underlying asset $\xi $ is approximated by random walks 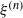${\xi }^{(n)} $. We obtain an explicit error formula, up to a term of order $ \mathcal{O} ({n}^{- 3/ 2} )$, which is valid for general approximating schemes and general payoff functions. We show how this error formula can be used to find random walks 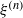${\xi }^{(n)} $ for which option values converge at a speed of 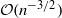$ \mathcal{O} ({n}^{- 3/ 2} )$.

Keywords

European optionsapproximation schemeerror formulaBlack–Scholes

MSC classification

Secondary: 91G20: Derivative securities 91G60: Numerical methods (including Monte Carlo methods) 62P05: Applications to actuarial sciences and financial mathematics
Type
Research Article
Information
The ANZIAM Journal , Volume 54 , Issue 4 , April 2013 , pp. 248 - 272
Copyright
Copyright ©2013 Australian Mathematical Society 

References

Carbone, R., “Binomial approximation of Brownian motion and its maximum”, Stat. Prob. Lett. 69 (2004) 271–285; doi:10.1016/j.spl.2004.06.020.CrossRefGoogle Scholar
Chang, L.-B. and Palmer, K., “Smooth convergence in the binomial model”, Finance Stoch. 11 (2007) 91–105; doi:10.1007/s00780-006-0020-6.CrossRefGoogle Scholar
Cox, J. C., Ross, S. A. and Rubinstein, M., “Option pricing: a simplified approach”, J. Financ. Econ. 7 (1979) 229–263; doi:10.1016/0304-405X(79)90015-1.CrossRefGoogle Scholar
Diener, F. and Diener, M., “Asymptotics of the price oscillations of a European call option in a tree model”, Math. Finance 14 (2004) 271–293; doi:10.1111/j.0960-1627.2004.00192.x.CrossRefGoogle Scholar
Diener, F. and Diener, M., “Higher-order terms for the de Moivre–Laplace theorem”, Contemp. Math. 373 (2005) 191–206; doi:10.1090/conm/373.CrossRefGoogle Scholar
Dupuis, P. and Wang, H., “Optimal stopping with random intervention times”, Adv. Appl. Prob. 34 (2002) 141–157; doi:10.1239/aap/1019160954.CrossRefGoogle Scholar
Dupuis, P. and Wang, H., “On the convergence from discrete to continuous time in an optimal stopping problem”, Ann. Appl. Probab. 15 (2005) 1339–1366; doi:10.1214/105051605000000034.CrossRefGoogle Scholar
Heston, S. and Zhou, G., “On the rate of convergence of discrete-time contingent claims”, Math. Finance 10 (2000) 53–75; doi:10.1111/1467-9965.00080.CrossRefGoogle Scholar
Hu, B., Liang, J. and Jiang, L., “Optimal convergence rate of the explicit finite difference scheme for American option valuation”, J. Comput. Appl. Math. 230 (2009) 583–599; doi:10.1016/j.cam.2008.12.018.CrossRefGoogle Scholar
Joshi, M. S., “Achieving smooth asymptotics for the prices of European options in binomial trees”, Quant. Finance. 9 (2009) 171–176; doi:10.1080/14697680802624955.CrossRefGoogle Scholar
Joshi, M. S., “Achieving higher order convergence for the prices of European options in binomial trees”, Math. Finance 20 (2010) 89–103; doi:10.1111/j.1467-9965.2009.00390.x.CrossRefGoogle Scholar
Kifer, Y., “Error estimates for binomial approximations of game options”, Ann. Appl. Probab. 16 (2006) 2273–2275; doi:10.1214/105051606000000808.CrossRefGoogle Scholar
Korn, R. and Müller, S., “The optimal-drift model: an accelerated binomial scheme”, Finance Stoch. 17 (2013) 135–160; doi:10.1007/s00780-012-0179-y.CrossRefGoogle Scholar
Lamberton, D., “Error estimates for the binomial approximation of American put options”, Ann. Appl. Probab. 8 (1998) 206–233; doi:10.1214/aoap/1027961041.CrossRefGoogle Scholar
Lamberton, D., “Vitesse de convergence pour des approximations de type binomial”, in: Mathématiques financières: modèles économiques et mathématiques des produits dérivés (INRIA, Rocquencourt, 1999), 347–359.Google Scholar
Lamberton, D., “Brownian optimal stopping and random walks”, Appl. Math. Optim. 45 (2002) 283–324; doi:10.1007/s00245-001-0033-7.CrossRefGoogle Scholar
Lamberton, D. and Rogers, L. C. G., “Optimal stopping and embedding”, J. Appl. Probab. 37 (2000) 1143–1148; doi:10.1239/jap/1014843094.CrossRefGoogle Scholar
Leduc, G., “Exercisability randomization of the American option”, Stoch. Anal. Appl. 26 (2008) 832–855; doi:10.1080/07362990802128669.CrossRefGoogle Scholar
Leduc, G., “Convergence rate of the binomial tree scheme for continuously paying options”, Ann. Sci. Math. Québec 36 (2012) 155–168; http://www.labmath.uqam.ca/annales/english/volumes/36-1.html.Google Scholar
Leisen, D. P. J., “Pricing the American put option: a detailed convergence analysis for binomial models”, J. Econom. Dynam. Control 22 (1998) 1419–1444; doi:10.1016/S0165-1889(98)00019-0.CrossRefGoogle Scholar
Leisen, D. P. J. and Reimer, M., “Binomial models for option valuation—examining and improving convergence”, Appl. Math. Finance 3 (1996) 319–346; doi:10.1080/13504869600000015.CrossRefGoogle Scholar
Liang, J., Hu, B., Jiang, L. and Bian, B., “On the rate of convergence of the binomial tree scheme for American options”, Numer. Math. 107 (2007) 333–352; doi:10.1007/s00211-007-0091-0.CrossRefGoogle Scholar
Lin, J. and Palmer, K., “Convergence of barrier option prices in the binomial model”, Math. Finance 23 (2013) 318–338; doi:10.1111/j.1467-9965.2011.00501.x.CrossRefGoogle Scholar
Tian, Y., “A flexible binomial option pricing model”, J. Futures Markets 19 (1999) 817–843; doi:10.1002/(SICI)1096-9934(199910)19:7<817::AID-FUT5>3.0.CO;2-D.3.0.CO;2-D>CrossRefGoogle Scholar
Walsh, J. B., “The rate of convergence of the binomial tree scheme”, Finance Stoch. 7 (2003) 337–361; doi:10.1007/s007800200094.CrossRefGoogle Scholar