The effect of yawing a wing in high-speed flow is to delay the onset of shock waves and to increase the critical Mach number. This is because shock waves can only develop along the isobars (running parallel to the edges of the wing, which is assumed untapered and infinitely long), and therefore only the velocity component normal to the edges is significant. To some extent the same is true for a finite sheared or swept-back wing, though the problem is made more complicated by the various additional factors, such as finite aspect ratio (tip effect), plan form (e.g. taper effect) and, what is perhaps the most important, the central kink effect. The experimental technique is extremely cumbersome because of the many shape parameters involved (see Figs. 1, 2, 3), and since analytical solutions present fundamental difficulties and pitfalls, designers tend to favour rule of thumb methods. The present paper contains a general review of the theoretical work done at The Royal Aircraft Establishment, which was limited to the case of zero incidence. This limitation is not a severe one, as flight at high speeds often implies low incidence. Incidences which are not negligible involve the solution of another fundamental problem (that of the lift distribution).

The method of analysis adopted by Cameron has been extended to develop more general equations for the correction of level speed and rate of climb for variation of air temperature and aircraft weight, applicable to aircraft with reciprocating engines of any type driving constant-speed propellers, operating at speeds sufficiently high for compressibility effects on drag and propeller efficiency to be appreciable. Allowance is made in the equations for ram effect on engine power.

The practical application of the equations is discussed, and illustrated by a worked example. The influence of errors in the estimation of the parameters expressing the variation of engine power and propeller efficiency on the corrections, in this particular case, is discussed.

When a uniform, two-dimensional supersonic flow expands suddenly round a corner in a wall it forms a pattern known as a Prandtl-Meyer expansion or centred simple wave. If the flow is two-dimensional but not initially uniform, or if it is axially-symmetrical, the expansion is still centred, but is not a simple wave. An approximate solution is given in this paper for the isentropic, irrotational, steady two-dimensional or axially-symmetrical flow of a perfect gas in the neighbourhood of the centre of such an expansion. The solution is designed to replace the conventional method of characteristics in such a region.

The main application is to a jet issuing from a nozzle that discharges into a container with a pressure lower than that in the nozzle; in particular, a formula is derived for the initial curvature, at the lip of the nozzle, of the boundary of the jet. The solution also applies to the flow near an edge in a boundary wall, and a formula is derived for the velocity gradient on the wall immediately downstream of the edge.

It is shown to be a consequence of kinematics and statics that the aerodynamic derivative expressing the rate of change of hinge moment of a plain flap with the angular setting of the flap varies parabolically with the chordwise position of the hinge. The derivative expressing the rate of change of hinge moment with incidence of the main surface varies linearly with the hinge position. Similar conclusions hold for the derivatives of the hinge moment with respect to the corresponding angular velocities.

The present note deals with the peculiarities of the lift distribution on swept wings. The influence of the angle of sweep is expected to be greatest in its effect on the chordwise lift distribution at the centre section of the wing. It is assumed that the essential characteristics of this chordwise lift distribution are predominantly determined by the special configuration of the bound vortices and not so much by the trailing vortices. In calculating the downwash, induced by a continuous chordwise distribution of swept vortex lines of infinite span, it is found that the downwash at the centre line is to be expected to differ from its two-dimensional form mainly by a term proportional to the local vortex strength. Solutions of this simplified downwash equation have been obtained. 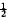They differ from the ordinary fiat-plate distribution only in the exponent which instead of becomes 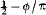.It is suggested that this type of function is more appropriate for the centre-section of swept wings and that it may replace the ordinary flat-plate distribution in all those cases where the lifting surface is swept.