Article contents
A weighted random walk model, with application to a genetic algorithm
Published online by Cambridge University Press: 01 July 2016
Abstract
We consider a weighted random walk model defined as follows. An n-step random walk on the integers with distribution Pn is weighted by giving the path S=(S0,…,Sn) a probability proportional to 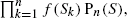 where the function f is the so-called fitness function. In the case of power-type fitness, we prove the convergence of the renormalized path to a deterministic function with exponential speed. This function is a solution to a variational problem. In the case of the simple symmetric random walk, explicit computations are done. Our result relies on large deviations techniques and Varadhan's integral lemma. We then study an application of this model to mutation-selection dynamics on the integers where a random walk operates the mutation. This dynamics is the infinite-population limit of that of mutation-selection genetic algorithms. We prove that the population grows to ∞ and make explicit its growth speed. This is a toy model for modelling the effect of stronger selection at ∞ for genetic algorithms taking place in a noncompact space.
where the function f is the so-called fitness function. In the case of power-type fitness, we prove the convergence of the renormalized path to a deterministic function with exponential speed. This function is a solution to a variational problem. In the case of the simple symmetric random walk, explicit computations are done. Our result relies on large deviations techniques and Varadhan's integral lemma. We then study an application of this model to mutation-selection dynamics on the integers where a random walk operates the mutation. This dynamics is the infinite-population limit of that of mutation-selection genetic algorithms. We prove that the population grows to ∞ and make explicit its growth speed. This is a toy model for modelling the effect of stronger selection at ∞ for genetic algorithms taking place in a noncompact space.
MSC classification
- Type
- General Applied Probability
- Information
- Copyright
- Copyright © Applied Probability Trust 2007
References
- 1
- Cited by


