No CrossRef data available.
Article contents
Random high-dimensional orders
Published online by Cambridge University Press: 01 July 2016
Abstract
The random k-dimensional partial order Pk(n) on n points is defined by taking n points uniformly at random from [0,1]k. Previous work has concentrated on the case where k is constant: we consider the model where k increases with n.
We pay particular attention to the height Hk(n) of Pk(n). We show that k = (t/log t!) log n is a sharp threshold function for the existence of a t-chain in Pk(n): if k – (t/log t!) log n tends to + ∞ then the probability that Pk(n) contains a t-chain tends to 0; whereas if the quantity tends to − ∞ then the probability tends to 1. We describe the behaviour of Hk(n) for the entire range of k(n).
We also consider the maximum degree of Pk(n). We show that, for each fixed d ≧ 2, 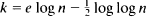 is a threshold function for the appearance of an element of degree d. Thus the maximum degree undergoes very rapid growth near this value of k.
is a threshold function for the appearance of an element of degree d. Thus the maximum degree undergoes very rapid growth near this value of k.
We make some remarks on the existence of threshold functions in general, and give some bounds on the dimension of Pk(n) for large k(n).
Keywords
MSC classification
- Type
- General Applied Probability
- Information
- Copyright
- Copyright © Applied Probability Trust 1995


