Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-03T04:17:08.947Z Has data issue: false hasContentIssue false
  • English
  • Français

A point-process approach to almost-sure behaviour of record values and order statistics

Part of: Limit theorems Stochastic processes

Published online by Cambridge University Press:  01 July 2016

Charles M. Goldie  and
Ross A. Maller
Charles M. Goldie
Affiliation:
Queen Mary and Westfield College, University of London
Ross A. Maller*
Affiliation:
University of Western Australia
*
∗∗ Postal address: Department of Mathematics, University of Western Australia, Nedlands, W. A. 6097, Australia.
Get access Rights & Permissions [Opens in a new window]

Abstract

Point-process and other techniques are used to make a comprehensive investigation of the almost-sure behaviour of partial maxima 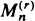(the rth largest among a sample of n i.i.d. random variables), partial record values 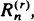and differences and quotients involving them. In particular, we obtain characterizations of such asymptotic properties as 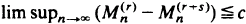a.s. for some finite constant c, or 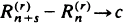a.s. for some constant c in [0,∞], which tell us, in various ways, how quickly the sequences increase. These characterizations take the form of integral conditions on the tail of F, which furthermore characterize such properties as stability and relative stability of the sequence of maxima. We also develop their relation to the large-sample behaviour of trimmed sums, and discuss some statistical applications.

Keywords

ALMOST-SURE BOUNDSHEAVY TAILSLIGHT TAILSORDER STATISTICSOUTLIER PRONEOUTLIER RESISTANTRECORD VALUERELATIVE STABILITYSTABILITYTRIMMED SUM

MSC classification

Primary: 60G70: Extreme value theory; extremal processes
Secondary: 60F15: Strong theorems 60G55: Point processes
Type
General Applied Probability
Information
Advances in Applied Probability , Volume 28 , Issue 2 , June 1996 , pp. 426 - 462
Copyright
Copyright © Applied Probability Trust 1996 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

∗

Present address: School of Mathematical Sciences, University of Sussex, Brighton, BN1 6HE, UK.

References

Barndorff-Nielsen, O. (1961) On the rate of growth of the partial maxima of a sequence of independent identically distributed random variables. Math. Scand. 9, 383–394.CrossRefGoogle Scholar
Barndorff-Nielsen, O. (1963) On the limit behaviour of extreme order statistics. Ann. Math. Statist. 34, 992–1002.CrossRefGoogle Scholar
Bingham, N. H., Goldie, C. M. and Teugels, J. L. (1989) Regular Variation (revised paperback edn). Encycl. of Math. and its Appl. 27. Cambridge University Press, Cambridge.Google Scholar
Deheuvels, P. (1981) The strong approximation of extremal processes. Z. Wahrscheinlichkeitsth. 58, 1–6.CrossRefGoogle Scholar
Deheuvels, P. (1983) The strong approximation of extremal processes II. Z. Wahrscheinlichkeitsth. 62, 7–15.CrossRefGoogle Scholar
Engelen, R., Tommassen, P. and Vervaat, W. (1988) Ignatov's theorem: a new and short proof. J. Appl. Prob. 25A, 229–236.CrossRefGoogle Scholar
Geffroy, J. (1958/59). Contributions à la théorie de valeurs extrêmes. Publ. Inst. Stat. Univ. Paris 7/8, 37–185.Google Scholar
Gnedin, A. V. (1994a) On a best-choice problem with dependent criteria. J. Appl. Prob. 31, 221–234.CrossRefGoogle Scholar
Gnedin, A. V. (1994b) Multiple maxima of a normal sample. Report. Universität Göttingen.Google Scholar
Goldie, C. M. and Resnick, S. I. (1989) Records in a partially ordered set. Ann. Prob. 17, 678–699.CrossRefGoogle Scholar
Goldie, C. M. and Rogers, L. C. G. (1984) The k-record processes are i.i.d. Z. Wahrscheinlichkeitsth. 67, 197–211.CrossRefGoogle Scholar
Green, R. F. (1976) Outlier-prone and outlier-resistant distributions. J. Amer. Statist. Assoc. 71, 502–505.CrossRefGoogle Scholar
Haan, L. De and Resnick, S. I. (1973) Almost sure limit points of record values. J. Appl. Prob. 10, 528–542.CrossRefGoogle Scholar
Haan, L. De and Resnick, S. I. (1977) Limit theory for multivariate sample extremes. Z. Wahrscheinlichkeitsth. 40, 317–337.CrossRefGoogle Scholar
Haiman, G. (1987) Etude des extrêmes d'une suite stationnaire m-dépendante avec une application relative aux accroissements du processus de Wiener. Ann. Inst. H. Poincaré–Prob. et Stat. 23, 425–458.Google Scholar
Hall, P. G. (1979a). On the relative stability of large order statistics. Math. Proc. Camb. Phil. Soc. 86, 467–475.CrossRefGoogle Scholar
Hall, P. G. (1979b) A note on a paper of Barnes and Tucker. J. London Math. Soc. 19, 170–174.CrossRefGoogle Scholar
Kesten, H. (1991) Unpublished manuscript.Google Scholar
Kesten, H. and Maller, R. A. (1992) Ratios of trimmed sums and order statistics. Ann. Prob. 20, 1805–1842.CrossRefGoogle Scholar
Kinoshita, K. and Resnick, S. I. (1991) Convergence of scaled random samples in Ann. Prob. 19, 1640–1663.CrossRefGoogle Scholar
Klass, M. J. (1986) The Robbins-Siegmund series criterion for partial maxima. Ann. Prob. 13, 1369–1370.Google Scholar
Li, Z. F. and Tomkins, R. J. (1991). Complete stability of large order statistics. J. Theor. Prob. 4, 213–221.CrossRefGoogle Scholar
Maller, R. A. and Resnick, S. I. (1984). Limiting behaviour of sums and the term of maximum modulus. Proc. London Math. Soc. 49, 385–422.CrossRefGoogle Scholar
Mathar, R. (1984) The limit behaviour of the maximum of random variables with applications to outlier resistance. J. Appl. Prob. 21, 646–650.CrossRefGoogle Scholar
Mori, T. (1976) The strong law of large numbers when extreme terms are excluded from sums. Z. Wahrscheinlichkeitsth. 36, 189–194.CrossRefGoogle Scholar
Nayak, S. S. and Wali, K. S. (1992) On the number of boundary crossings related to LIL and SLLN for record values and partial maxima of i.i.d. sequences and extremes of uniform spacings. Stoch. Proc. Appl. 43, 317–329.CrossRefGoogle Scholar
Neyman, J. and Scott, E. L. (1971) Outlier proneness of phenomena and of related distributions. In Optimizing Methods in Statistics. ed. Rustagi, J. S. Academic Press, New York.Google Scholar
Pickands, J. (1971) The two-dimensional Poisson process and extremal processes. J. Appl. Prob. 8, 745–756.CrossRefGoogle Scholar
Pruitt, W. E. (1987) The contribution to the sum of the summand of maximum modulus. Ann. Prob. 15, 885–896.CrossRefGoogle Scholar
Resnick, S. I. (1973) Record values and maxima. Ann. Prob. 4, 650–662.Google Scholar
Resnick, S. I. (1986) Point processes, regular variation and weak convergence. Adv. Appl. Prob. 18, 66–138.CrossRefGoogle Scholar
Resnick, S. I. (1987) Extreme Values, Regular Variation and Point Processes. (Applied Probability 4) Springer, New York.CrossRefGoogle Scholar
Resnick, S. I. and Tomkins, R. J. (1973) Almost sure stability of maxima. J. Appl. Prob. 10, 387–401.CrossRefGoogle Scholar
Sepanski, S. J. (1993) Almost sure bootstrap of the mean under random normalization. Ann. Prob. 21, 917–925.CrossRefGoogle Scholar
Shanbhag, D. N. (1979) Some refinements in distribution theory. Sankhya A 41, 251–262.Google Scholar
Tomkins, R. J. (1986) Regular variation and the stability of maxima. Ann. Prob. 14, 984–995.CrossRefGoogle Scholar
Vervaat, W. (1977) Success Epochs in Bernoulli Trials. 2nd edn. (Math. Centrum Tracts 42) Math. Centrum, Amsterdam.Google Scholar
Wang, H. and Tomkins, R. J. (1992) A zero-one law for large order statistics. Canad. J. Statist. 20, 323–334.CrossRefGoogle Scholar
Zaanen, A. C. (1958) An Introduction to the Theory of Integration. North-Holland, Amsterdam.Google Scholar