Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-23T17:17:28.949Z Has data issue: false hasContentIssue false
  • English
  • Français

On a problem of ammunition rationing

Published online by Cambridge University Press:  01 July 2016

Lawrence A. Shepp ,
Gordon Simons  and
Yi-Ching Yao
Lawrence A. Shepp*
Affiliation:
AT & T Bell Laboratories
Gordon Simons*
Affiliation:
University of North Carolina
Yi-Ching Yao*
Affiliation:
Colorado State University
*
∗Postal address: AT&T Bell Laboratories, Room 2c-3, 600 Mountain Avenue, Murray Hill, NJ 07974-2070, USA.
∗∗Postal address: Department of Statistics, CB #3260, Phillips Hall, University of North Carolina, Chapel Hill, NC 27599-3260, USA.
∗∗∗Postal address: Department of Statistics, Colorado State University, Fort Collins, CO 80523, USA.
Get access Rights & Permissions [Opens in a new window]

Abstract

Suppose you have u units of ammunition and want to destroy as many as possible of a sequence of attacking enemy aircraft. If you fire v = v(u), 0 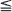, units of your ammunition at the first enemy, it survives with probability qv, where 0 < q < 1 is given, and then kills you. With the complementary probability, 1 – qv, you destroy the aircraft and you live to face the next enemy with only u – v units of ammunition remaining. It seems almost obvious that any strategy which maximizes the expected number of enemies destroyed before you die will fire more units at the first enemy as u increases, i.e., it seems obvious that v′(u) 0 under optimal play. We show this to be false, thereby disproving an appealing conjecture proposed by Weber. We also consider a variant of this problem and find that the counterpart of Weber's conjecture holds in some cases.

Keywords

OPTIMAL ALLOCATIONBOMBER PROBLEM
Type
Research Article
Information
Advances in Applied Probability , Volume 23 , Issue 3 , September 1991 , pp. 624 - 641
Copyright
Copyright © Applied Probability Trust 1991 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Research supported by the National Science Foundation, Grant No. DMS-8701201.

References

Klinger, A. and Brown, T. A. (1968) Allocating unreliable units to random demands. Stochastic Optimization and Control , ed. Karreman, H., pp. 173–209. Publ. No. 20, Math. Research Center, U.S. Army and Univ. Wisconsin, Wiley, New York.Google Scholar
Samuel, E. (1970) On some problems of operations research. J. Appl. Prob. 7, 157–164.CrossRefGoogle Scholar
Simons, G. and Yao, Y.-C. (1990) Some results on the bomber problem. Adv. Appl. Prob. 22, 412–432.CrossRefGoogle Scholar
Weber, R. (1985) A problem of ammunition rationing. Abstract, Conference Report: Stochastic Dynamic Optimization and Applications in Scheduling and Related Areas, held at Universität Passau, Fakultät für Mathematik und Informatik, p. 148.Google Scholar