Published online by Cambridge University Press: 06 March 2023
We study a general model of recursive trees where vertices are equipped with independent weights and at each time-step a vertex is sampled with probability proportional to its fitness function, which is a function of its weight and degree, and connects to
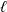 $\ell$
new-coming vertices. Under a certain technical assumption, applying the theory of Crump–Mode–Jagers branching processes, we derive formulas for the limiting distributions of the proportion of vertices with a given degree and weight, and proportion of edges with endpoint having a certain weight. As an application of this theorem, we rigorously prove observations of Bianconi related to the evolving Cayley tree (Phys. Rev. E 66, paper no. 036116, 2002). We also study the process in depth when the technical condition can fail in the particular case when the fitness function is affine, a model we call ‘generalised preferential attachment with fitness’. We show that this model can exhibit condensation, where a positive proportion of edges accumulates around vertices with maximal weight, or, more drastically, can have a degenerate limiting degree distribution, where the entire proportion of edges accumulates around these vertices. Finally, we prove stochastic convergence for the degree distribution under a different assumption of a strong law of large numbers for the partition function associated with the process.
$\ell$
new-coming vertices. Under a certain technical assumption, applying the theory of Crump–Mode–Jagers branching processes, we derive formulas for the limiting distributions of the proportion of vertices with a given degree and weight, and proportion of edges with endpoint having a certain weight. As an application of this theorem, we rigorously prove observations of Bianconi related to the evolving Cayley tree (Phys. Rev. E 66, paper no. 036116, 2002). We also study the process in depth when the technical condition can fail in the particular case when the fitness function is affine, a model we call ‘generalised preferential attachment with fitness’. We show that this model can exhibit condensation, where a positive proportion of edges accumulates around vertices with maximal weight, or, more drastically, can have a degenerate limiting degree distribution, where the entire proportion of edges accumulates around these vertices. Finally, we prove stochastic convergence for the degree distribution under a different assumption of a strong law of large numbers for the partition function associated with the process.