Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-16T18:24:09.470Z Has data issue: false hasContentIssue false
  • English
  • Français

A branching-process solution of the polydisperse coagulation equation

Published online by Cambridge University Press:  01 July 2016

John L. Spouge
John L. Spouge*
Affiliation:
Trinity College, Oxford
Get access Rights & Permissions [Opens in a new window]

Abstract

The polydisperse coagulation equation 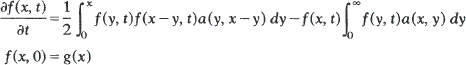models irreversible aggregation of particles with varying masses. This paper uses a one-parameter family of discrete-time continuous multitype branching processes to solve the polydisperse coagulation equation when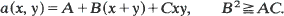

The critical time tc when diverges corresponds to a critical branching process, while post-critical times t> tc correspond to supercritical branching processes.

Keywords

SMOLUCHOWSKI COAGULATION EQUATIONPOLYMERISATIONBRANCHING PROCESSES
Type
Research Article
Information
Advances in Applied Probability , Volume 16 , Issue 1 , March 1984 , pp. 56 - 69
Copyright
Copyright © Applied Probability Trust 1984 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Drake, R. (1972a) The scalar transport equation of coalescence theory: moments and kernels. J. Atmos. Sci. 29, 537–547.Google Scholar
Drake, R. (1972b) in Topics in Current Aerosol Research, ed. Hidy, G. M. and Brock, J. R., 3, 201.Google Scholar
Dusek, K. (1979) Correspondence between the theory of branching processes and the kinetic theory for random cross-linking in the post-gel state. Poly. Bull. 1, 523–528.Google Scholar
Ernst, M. H., Hendriks, E. M. and Ziff, R. M. (1982a) Critical kinetics near the gelation transition. J. Phys. A. Math. Gen. 15, L743–L747.Google Scholar
Ernst, M. H., Hendriks, E. M. and Ziff, R. M. (1982b) Exact solutions to the coagulation equation. Phys. Lett. 92A, 267–270.CrossRefGoogle Scholar
Flory, P. J. (1941a) Molecular size distribution in three dimensional polymers. I. Gelation. J. Amer. Chem. Soc. 63, 3083–3090.Google Scholar
Flory, P. J. (1941b) Molecular size distribution in three dimensional polymers. II. Trifunctional branching units. J. Amer. Chem. Soc. 63, 3091–3096.Google Scholar
Flory, P. J. (1941c) Molecular size distribution in three dimensional polymers. III. Tetrafunctional branching units. J. Amer. Chem. Soc. 63, 3096–3100.Google Scholar
Flory, P. J. (1953) Principles of Polymer Chemistry. Cornell University Press, Ithaca, New York.Google Scholar
Good, I. J. (1948) The number of individuals in a cascade process. Proc. Camb. Phil. Soc. 45, 360–363.Google Scholar
Gordon, M. (1962) Good's theory of cascade processes applied to the statistics of polymer distributions. Proc. R. Soc. London, A268, 240–259.Google Scholar
Gordon, M. and Malcolm, G. N. (1966) Configurational statistics of copolymer systems. Proc. R. Soc. London A295, 29–54.Google Scholar
Harris, T. E. (1963) The Theory of Branching Processes. Springer-Verlag, Berlin.CrossRefGoogle Scholar
Hendriks, E. M., Ernst, M. H. and Ziff, R. M. (1982) Coagulation equations with gelation. J. Statist. Phys. Google Scholar
Smoluchowski, M. Von (1917) Versuch einer mathematischen theorie der koagulationskinetic kolloider Losungen. Z. Phys. Chem. 92, 129–168.Google Scholar
Spouge, J. L. (1983a) Solutions and critical times for the polydisperse coagulation equation when a(x, y) = A + B(x + y) + Cxy. J. Phys. A 16, 3127–3132.Google Scholar
Spouge, J. L. (1983b) Equilibrium polymer distributions. Macromolecules 16, 121–127.CrossRefGoogle Scholar
Spouge, J. L. (1983c) The size distribution for the AgRBf-g model of polymerization. J. Statist. Phys. 31, 363–378.CrossRefGoogle Scholar
Spouge, J. L. (1983d) Asymmetric bonding of identical units: a general AgRBf-g polymer model. Macromolecules 16, 831–835.Google Scholar
Spouge, J. L. (1983e) Tree models of aggregation: multiple particle- and bond-types. Proc. R. Soc. London A 387, 351–365.Google Scholar
Stockmayer, W. H. (1943) Theory of molecular size distributions and gel formation in branched-chain polymers. J. Chem. Phys. 11, 45–55.Google Scholar
Stockmayer, W. H. (1944) Theory of molecular size distributions and gel formation in branched-chain polymers. II. General cross-linking. J. Chem. Phys. 12, 125–131.Google Scholar
Whittle, P. (1965) The equilibrium statistics of a clustering process in the uncondensed phase. Proc. R. Soc. London, A285, 501–519.Google Scholar
Whittle, P. (1980a) Polymerisation processes with intrapolymer bonding. I. One type of unit. Adv. Appl. Prob. 12, 94–115.Google Scholar
Whittle, P. (1980b) Polymerisation processes with intrapolymer bonding. II. Stratified processes. Adv. Appl. Prob. 12, 115–134.Google Scholar
Whittle, P. (1980C) Polymerisation processes with intrapolymer bonding. III. Several types of unit. Adv. Appl. Prob. 12, 135–153.Google Scholar
Whittle, P. (1980d) A direct derivation of the equilibrium distribution of a polymer process. Theory Prob. Appl. 26, 350–361.Google Scholar
Ziff, R. M. (1980) Kinetics of polymerization. J. Statist. Phys. 23, 241–263.CrossRefGoogle Scholar
Ziff, R. M. and Stell, G. (1980) Kinetics of polymer gelation. J. Chem. Phys. 73, 3492–3499.CrossRefGoogle Scholar