Article contents
Asymptotic stationarity of multichannel queues
Published online by Cambridge University Press: 01 July 2016
Abstract
The paper deals with asymptotic stationarity of the process 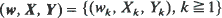 where
where 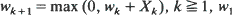 is a vector in
is a vector in  with non-negative coordinates,
with non-negative coordinates, 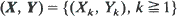 is an
is an 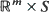 -valued process, S is a separable metric space and all operations in
-valued process, S is a separable metric space and all operations in 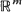 are meant in the coordinate-wise sense. It is shown that a type of asymptotic stationarity of (X, Y), together with some conditions, implies the same type of asymptotic stationarity of (w, X, Y). This result is applied to analyze asymptotic stationarity of multichannel queues. It may also be used to analyze asymptotic stationarity of series of multichannel queues.
are meant in the coordinate-wise sense. It is shown that a type of asymptotic stationarity of (X, Y), together with some conditions, implies the same type of asymptotic stationarity of (w, X, Y). This result is applied to analyze asymptotic stationarity of multichannel queues. It may also be used to analyze asymptotic stationarity of series of multichannel queues.
Keywords
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © Applied Probability Trust 1993
References
- 1
- Cited by


